A ball is projected from a point in a horizontal plane so as to strike a vertical wall at right angle A.after rebounding from the wall it strikes the horizontal plane once and returns to the point of projection just before second collision at horizontal surface. Find co-efficient of restitution for the two collisions, assuming it to be same for both the collision.
1/2
1/3
1/4
1/5
The correct Answer is:A
h=u2sin2θ2g,x=R2u2sinθcosθg
V1x=eucosθ(←),
V1y=√2gh=usinθ(⏐↓)
V2x=V1x=eucosθ(←),
V2y=eusinθ(↑⏐)
From B→C, equation of trajectory gives,
−h=O−gx212(eV)2⇒x1=eu2sinθcosθgandx2=2V2xV2yg=2e2u2sinθcosθg
∵ x1+x2=x ⇒ e+2e2=1 e=12

Topper's Solved these Questions
Similar Questions
A ball is projected from ground with a speed 70m/s at an angle 45∘ with the vertical so that it strikes a vertical wall at horizontal distance 4903m from the point of projection. If the ball returns back to the poin tof projection without any collision with ground then find the coefficient of restitution between the ball and wall.
View SolutionA ball falls from a height 20m on to a horizontal plane and rebound to a height of 5/64m after 4 collisions.Find the coefficient of restitution.
View Solution
Knowledge Check
- Question 1 - Select One
A ball is projected from a point in a horizontal plane so as to strike a vertical wall at right angle A.after rebounding from the wall it strikes the horizontal plane once and returns to the point of projection just before second collision at horizontal surface. Find co-efficient of restitution for the two collisions, assuming it to be same for both the collision.
A1/2B1/3C1/4D1/5 - Question 2 - Select One or More
A ball is projected from a point in a horizontal plane with a velocity of 20 m/s at 30∘ with the horizontal. Coefficient of restitution is 0.8, then
Aduration for which ball will rebound is 20 sBduration for which ball will rebound is 10 sChorizontal component of velocity of the ball is 17.3 m/s.Dhorizontal distance before the ball ceases to rebound is 173 m - Question 3 - Select One
A ball is projected at an angle 45∘ with horizontal. It passes through a wall of height h at horizontal distance d1 from the point of projection and strikes the ground at a horizontal distance (d1+d2) from the point of projection, then
Ah=2dd_(2)d+d2Bh=d1d2d1+d2Ch=√2d1d2d1+d2Dh=d1d22(d1+d2)
A ball after falling a distance collides an inclined plane of inclination θ. If after the collision , the ball moves horizontally , find the coefficient of restitution.
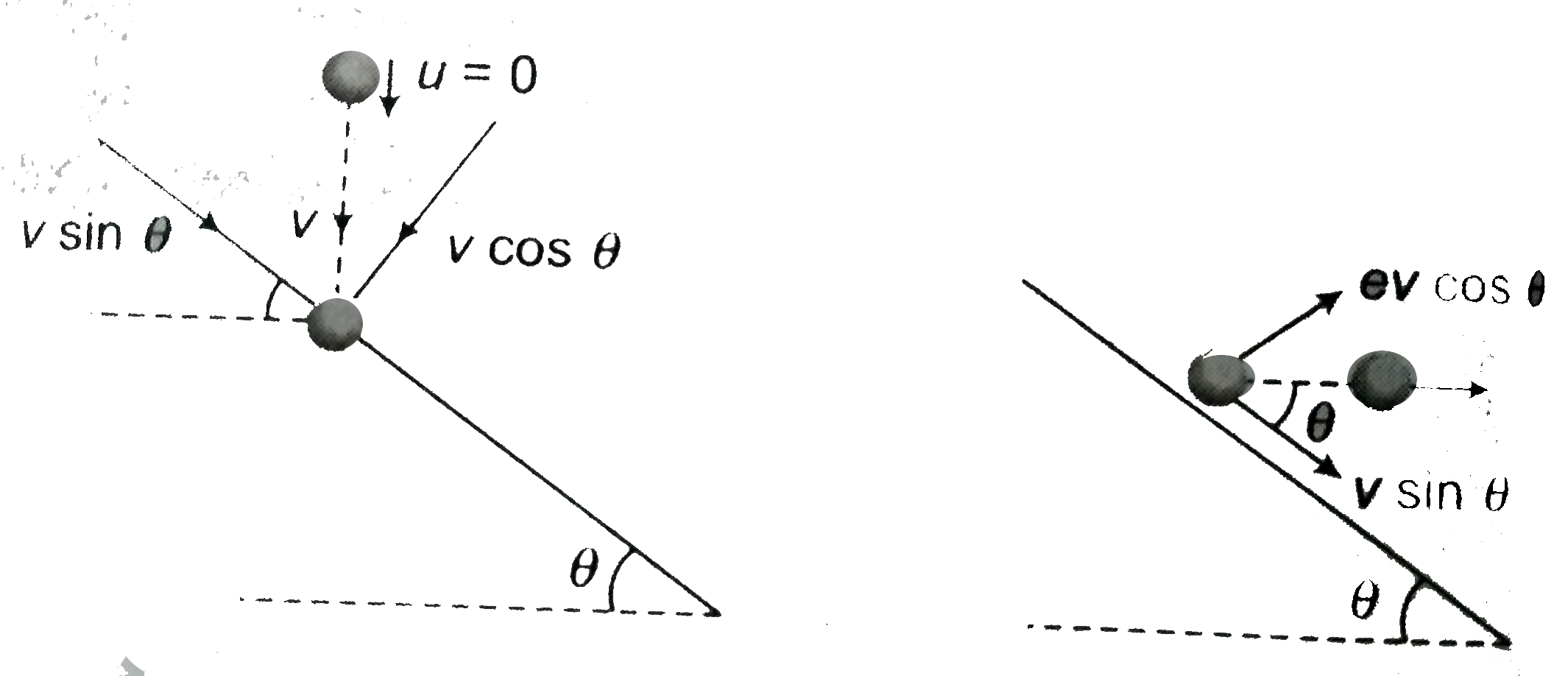 View Solution
View SolutionA small ball is projected with a velocity u at some angle with horizontal towards a smooth vertical wall at a distance d from the point of projection after rebounding from the wall . If e is the coefficient of restitution then find maximum distance d for which ball may return to point of projection .
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at a distance a from the point of projection and returns to its original position. Find the coefficient of restitution between the ball and the wall.
View SolutionA ball falls from height h over an inclined plane of inclination 30∘ as shown. After the collision, the ball moves horizontally, the coefficient of restitution is
_PHY_224_Q01.png) View Solution
View SolutionA particle just clear a wall of height b at a distance a and strikes the ground at a distance from the point of projection, the angle of projection is :
View Solution

