As shown in figure, there is a thick spherical shell with the walls coated with 'lamp black'. A point source which generates thermal energy at a constant rate 'P' is placed at the centre S of the shell. Derive an expression for the temperature T at point M in steady state, where SM = 1.5 R. Your expression would be as follows :
T=(Pσ16πR2)1/4+(P3×πKR)
Here K is the coefficient of thermal conductivity of material of shell and σ is Stefan's constant. Find the value of x.
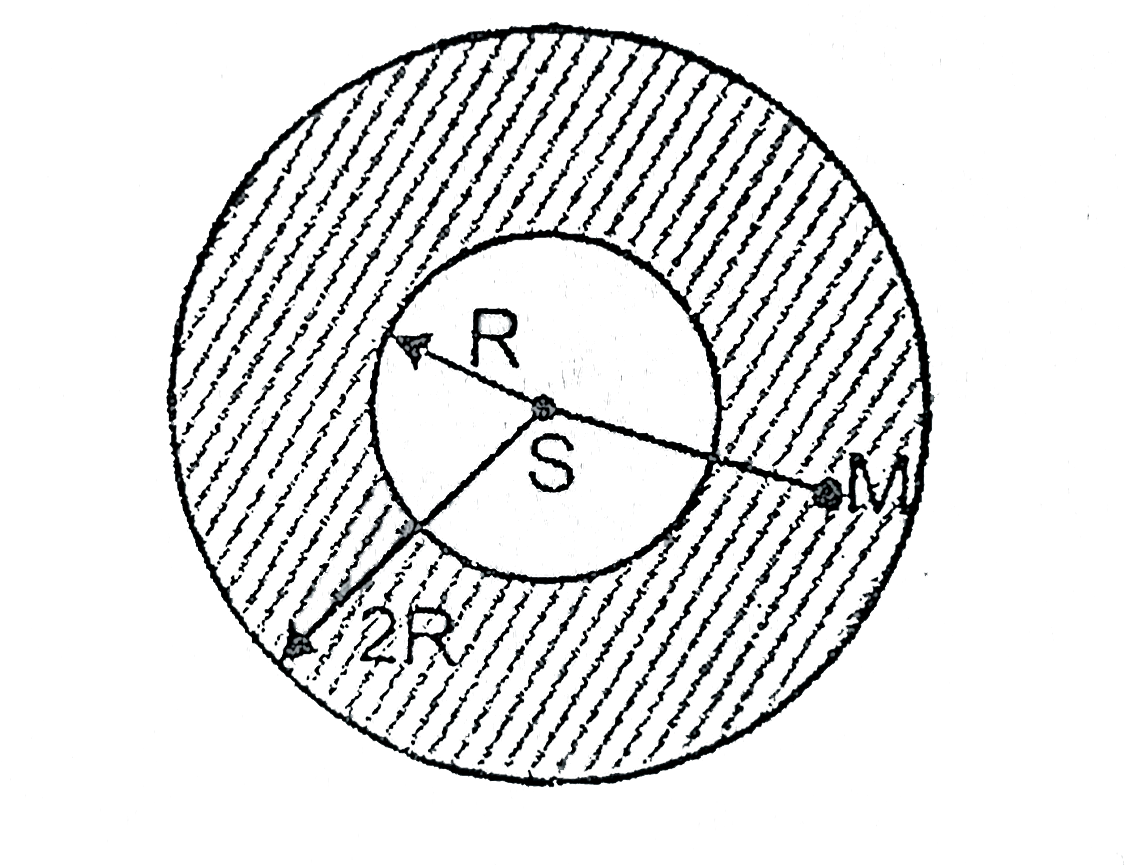
The correct Answer is:8
P=σ4π(2R)2T4S
dR=drk4πr2
Req=14πk2R∫rdrr2, Req=−14πk[1r]2R
Req=−14πk[12R−1r]=14πk(1r−12R)=(2R−r)8πkRr
P=(T−TS)8πkRr(2R−r)=(T−TS)(24Rπk)
T=TS+(P24Rπk)=(P16πσR2)14+(P24Rπk)
x=8
Topper's Solved these Questions
Similar Questions
A thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R/2 from the centre of the shell is
View SolutionA thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
View Solution
Knowledge Check
A thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
A(a) 2Q4πε0RB(b) 2Q4πε0R−2q4πε0RC(c) 2Q4πε0R+q4πε0RD(d) (q+Q)24πε0RA point mass m is placed inside a spherical shell of radius R and mass M at a distance R2 form the centre of the shell. The gravitational force exerted by the shell on the point mass is
AGMmR2B2GMmR2CzeroDGMMR2A point mass m is placed inside a spherical shell of radius R and mass M at a distance R2 form the centre of the shell. The gravitational force exerted by the shell on the point mass is
AGMmR2B2GMmR2CzeroD4MmR2
Derive an expression for electric intensity E due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionA point charge Q is placed inside a conducting spherical shell of inner radius 3R and outer radius 5R at a distance R from the centre of the shell. The electric potential at the centre of the shell will be
View SolutionA point charge Q is placed inside a conducting spherical shell of inner radius 3R and outer radius 5R at a distance R from the centre of the shell. The electric potential at the centre of the shell will be
View SolutionA thin metallic spherical shell contains a charge Q on it. A point charge q is placed at the center of the shell and another charge q1 is placed outside it as shown in figure. All the three charges are positive
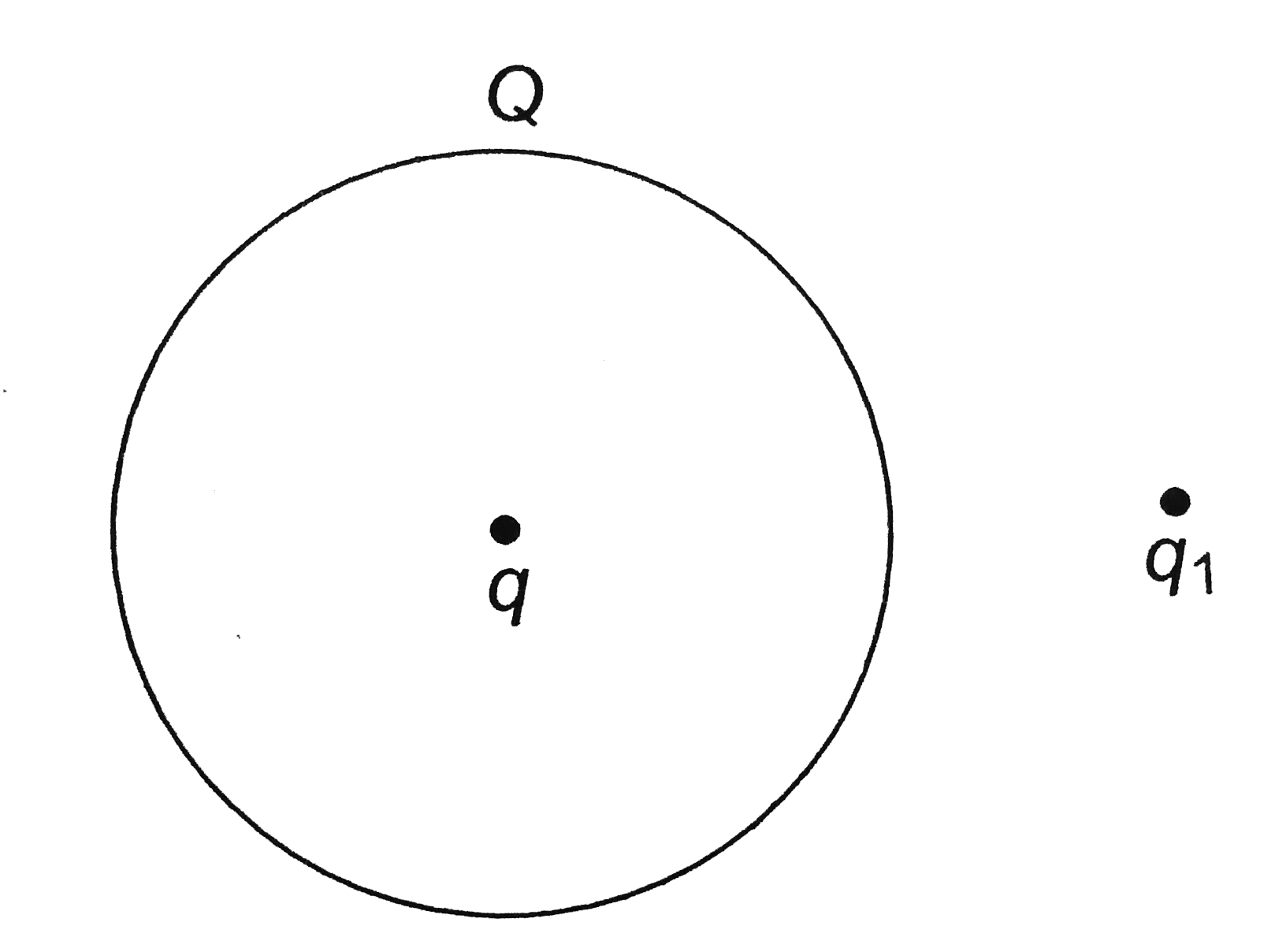
The force on the charge at the centre is
View SolutionP is a point at a distance r from the centre of a spherical shell of mass M and radius a, where r<a. The gravitational potential at P is
View Solution
