The only force acting on a block is along x-axis is given by , When the block moves from x= -2 m to x = 4 m, the change in kinetic energy of block is-
Positive
Negative
Zero
May be positive or negative
The correct Answer is:A
To solve the problem, we need to find the change in kinetic energy of the block as it moves from x=−2m to x=4m under the influence of the force P=−4x2+2N. The change in kinetic energy can be determined by calculating the work done by the force over the specified distance.
1. Identify the Force: The force acting on the block is given by:
P=−4x2+2N
2. Set Up the Work Done Integral: The work done W by the force as the block moves from x=−2m to x=4m is given by the integral of the force over the displacement:
W=∫4−2Pdx=∫4−2−4x2+2dx
3. Calculate the Integral: We can compute the integral:
W=−4∫4−21x2+2dx
The integral ∫1x2+2dx can be solved using the formula:
∫1a2+x2dx=1atan−1(xa)+C
where a=√2. Thus,
∫1x2+2dx=1√2tan−1(x√2)+C
4. Evaluate the Definite Integral:
W=−4(1√2tan−1(x√2))4−2
Now, we need to evaluate this at the limits:
W=−4(1√2tan−1(4√2)−1√2tan−1(−2√2))
5. Calculate the Values:
- For x=4:
tan−1(4√2)=tan−1(2√2)
- For x=−2:
tan−1(−2√2)=tan−1(−√2)
6. Final Calculation: Substitute the values back into the equation for work done:
W=−4(1√2(tan−1(2√2)−tan−1(−√2)))
7. Determine Change in Kinetic Energy: The change in kinetic energy ΔKE is equal to the work done:
ΔKE=W
Conclusion:
Since the force is negative and the integral evaluates to a negative value, the change in kinetic energy will also be negative. Thus, the final answer is that the change in kinetic energy of the block is negative.
P=−4x2+2N
W=∫4−2Pdx=∫4−2−4x2+2dx
W=−4∫4−21x2+2dx
The integral ∫1x2+2dx can be solved using the formula:
∫1a2+x2dx=1atan−1(xa)+C
where a=√2. Thus,
∫1x2+2dx=1√2tan−1(x√2)+C
W=−4(1√2tan−1(x√2))4−2
Now, we need to evaluate this at the limits:
W=−4(1√2tan−1(4√2)−1√2tan−1(−2√2))
- For x=4:
tan−1(4√2)=tan−1(2√2)
- For x=−2:
tan−1(−2√2)=tan−1(−√2)
W=−4(1√2(tan−1(2√2)−tan−1(−√2)))
ΔKE=W
Since the force is negative and the integral evaluates to a negative value, the change in kinetic energy will also be negative. Thus, the final answer is that the change in kinetic energy of the block is negative.
Topper's Solved these Questions
Similar Questions
The potential energy of a particle of mass 2kg moving along the x-axis is given by U(x)=4x2−2x3( where U is in joules and x is in meters). The kinetic energy of the particle is maximum at
View SolutionThe only force acting on a 2.0−kg body as it moves along the x-axis varies as shown in figure. The velocity of the body at x=0 is 4.0ms−1.
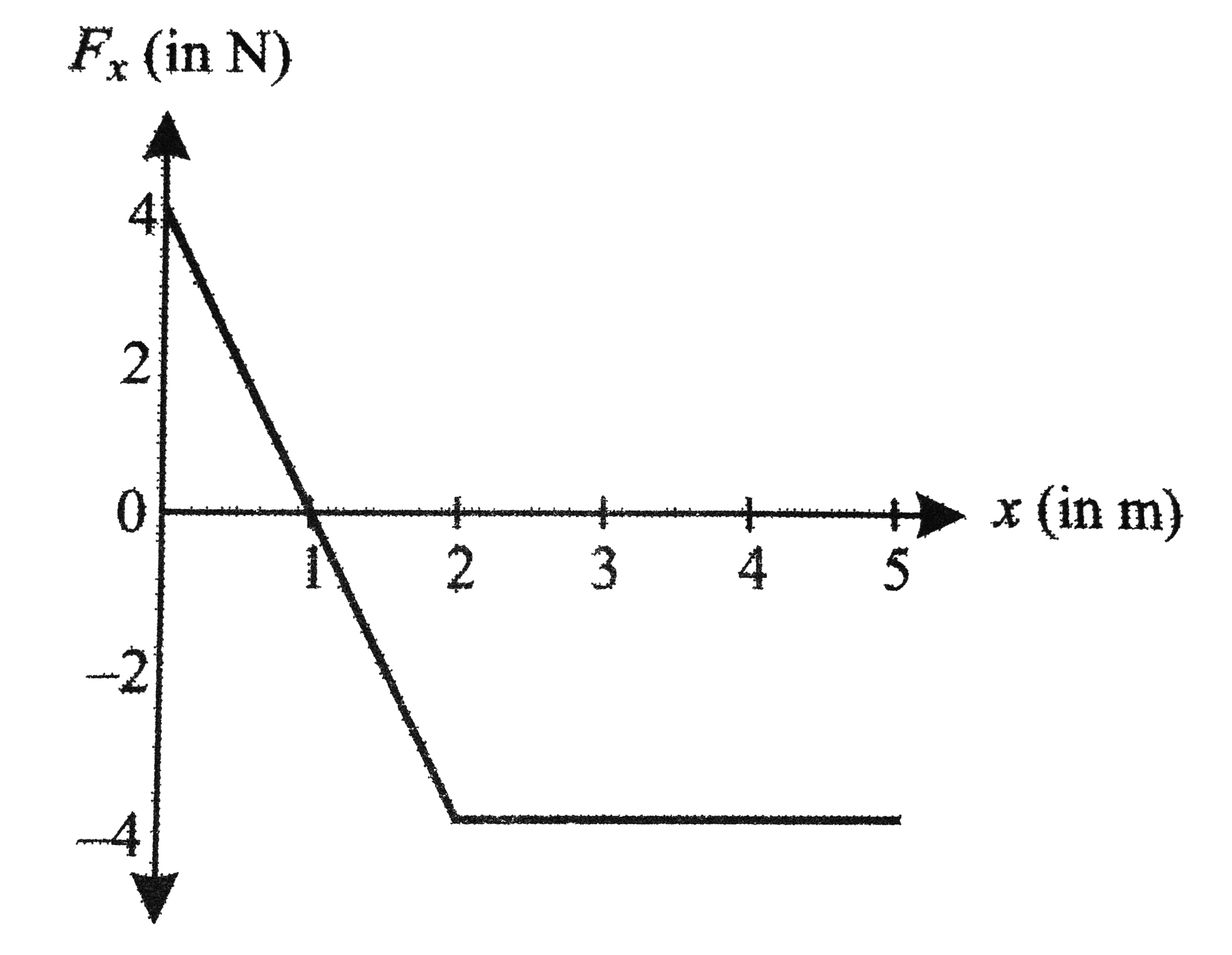
a. What is the kinetic energy of the body at x=3.0m?
b. At what value of x will the body have a kinetic energy of 8.0J?
c. What is the maximum kinetic energy attained by the body between x=0 and x=5.0m?View Solution
Knowledge Check
- Question 1 - Select One
Force acting on a block moving along x-axis is given by
F=−(4x2+2)N
The block is displaced from x=−2m to x=+4m, the work done will beApositiveBnegativeCzeroDmay be positive or negative - Question 2 - Select One
A force of (5 +3x) N acting on a body of mass 20 kg along the x-axis displaces it from x= 2 m to x = 6 m . The work done by the force is
A20 JB48 JC68 JD86 J - Question 3 - Select One
A force of (5 +3x) N acting on a body of mass 20 kg along the x-axis displaces it from x= 2 m to x = 6 m . The work done by the force is
A20 JB48 JC68 JD86 J
A 1.0 kg block is initially at rest on a horizontal frictionless surface when a horizontal force along an x axis is applied to the block. The force is given by →F(x)=(2.5−x2)ˆiN, where x is in meters and the initial position of the block is x=0. (a) What is the kinetic energy of the block as it passes through x=2.0m ? (b) What is the maximum kinetic energy of the block between x=0 and x=2.0m ?
View SolutionA block is initially at rest on a horizontal frictionless surface when a horizontal force in the positive direction of an axis is applied to the block. The force is given by →F=(1−x2)ˆiN, where x is in meters and the initial position of the block is x=0 the maximum kinetic energy of the block is 2nJ in between x=0 ad x=2m find the value of n.
View SolutionIn Fig 15.5, the block has a kinetic energy of 3J and the spring has an elastic potential energy of 2J when the block is at x=+2.0cm. (a) What is the kinetic energy when the block is at x= 0? What is the elastic potential energy when the block is at (b) x=−2.0cm and (c ) x=−xm?
View SolutionA 1.5−kg block is initially at rest on a horizontal frictionless surface when a horizontal force in the positive direction of x-axis is applied to the block. The force is given by →F=(4−x2)→iN, where x is in meter and the initial position of the block is x=0.
The maximum kinetic energy of the block between x=0 and x=2.0m isView SolutionA 1.5kg block is initially at rest on a horizontal frictionless surface. A horizontal force →F=(4−x2)ˆi is applied on the block. Initial position of the block is at x=0. The maximum kinetic enery of the block between x=0 and x=2m is
View Solution

