A ship is moving at a constant speed of 10km/hr in the direction of the unit vector ˆi. Initially. Its position vector, relative to a fixed origin is 10(−ˆi+ˆj) where ˆi & ˆj are perpendicular vectors of length 1 km. Find its position vector relative to the origin at time t hours later. A second ship is moving with constant speed ukm/hr parallele to the vector ˆi+2ˆj and is initially at the origin
(i) If u=10√5km/h. Find the minimum distance between the ships and the corresponding value of t
(ii) Find the value of u for which the shipd are on a collision course and determine the value of t at which the collision would occur if no avoiding action were taken.
Text Solution
The correct Answer is:→s=10(t−1)ˆi+10ˆj(a)t=12h,10km (b) 10√52,t=2hr
It's velocity is 10ˆi
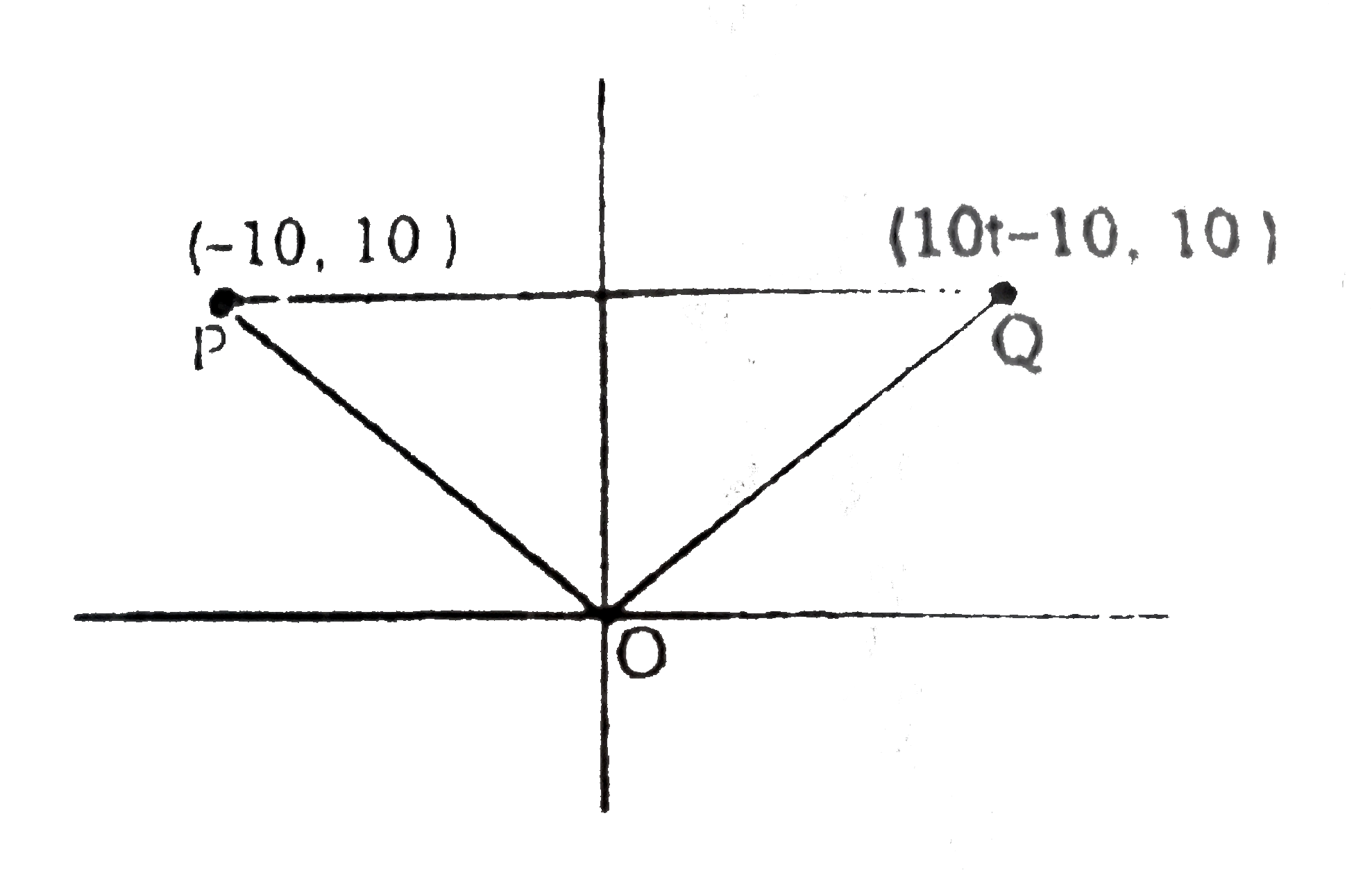
∴ displacement after time 't'=10ˆi×t
Velocity of second ship =u×(ˆi+2ˆj)√5
tanθ=2u/5(10−u√5)=2×10√510√5−10√5
(i) t=1020=120sec, minimum distance = 10 km.
|
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]
11 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - Exercise-05[B]
5 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-04[A]
35 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
Knowledge Check
Unit vector along the vector ˆi+ˆj+ˆk is
A13(ˆi+ˆk+ˆk)B12(ˆi+ˆj+ˆk)C1√3(ˆi+ˆj+ˆk)D1√2(ˆi+ˆj+ˆk)The unit vector along ˆi+ˆj is
AˆkBˆi+ˆjCˆi+ˆj√2Dˆi+ˆj2Unit vector perpendicular to the vectors ˆi−ˆj and ˆi+ˆj is
AˆkB−ˆkCˆi−ˆj√2Dˆi+ˆj√2
Find the projection of the vector ˆi−ˆjon the vector ˆi+ˆj
View SolutionFind the projection of the vector ˆi−ˆjon the vector ˆi+ˆj
View SolutionFind the unit vector in the direction of the vector →a=ˆi+ˆj+2ˆk
View SolutionWrite the projection of the vector ˆi−ˆj on the vector ˆi+ˆj
View SolutionFind the unit vector in the direction of the vector →a=ˆi+ˆj+ 2ˆk .
View Solution
