Equivalent ratios
Equivalent Ratios
In this chapter, we will learn equivalent ratios. We will try to understand as to how to find equivalent ratios. In order to understand more, we will have a look at the equivalent ratios example that would help us in getting the right idea about it.
What is Equivalent Ratio?
A ratio which is obtained by dividing or multiplying the numerator and denominator of a ratio by the same number is known as an equivalent-ratio.
How to find Equivalent Ratios?
In order to find equivalent ratios, we need to make a multiplication or division of both the terms of the given ration which needs to be done by the same non-zero number. It is important to learn as to how to determine the equivalent ratios of a ratio by writing the ratio in the form of fraction. It again needs to be compared by using multiplication and division.
For example if we need to find two equivalent-ratios of 6 : 15.
Then in order to find two equivalent-ratios of 6 : 15.
Here, 6/15 = (6 x 2)/(15 x 2) = 12/30
6/15 = (6 ÷ 3)/(15 ÷ 3) = 2/5
Therefore, 12 : 30 and 2 : 5 are the two equivalent-ratios of 6 : 15.
We will now work on some equivalent ratios examples so that it can help you to get the perfect idea on how to work on it in the best way.
Question: Find the value of ‘a’ and ‘b’ in 12:20
Solution: Let us consider the 1st two ratios,
12/20 = a/5
⇒ 12 x 5 = 20 x a
60 = 20a
∴ a = 60/20
∴ a = 3
In order to find b, consider 1st and 3rd ratio.
Here, 12/20 = 9/b ⇒ 12 x b = 20 x 9
12b = 180
So, b = 180/12
Therefore b = 15.
Related Videos:
Question: Give two equivalent ratios of 8 : 18.
Solution: In order to find equivalent ratios of 8 : 18, it needs to be written as fraction.
= 8/12
= (8 × 2)/(18 × 2)
= 16/36
= 16 : 36 (one equivalent ratio),
Therefore, 16 : 36 is an equivalent ratio of 8 : 18.
Now in order to find another equivalent ratio of 8 : 18 by using division.
We need to express the given ratio as fraction,
= 8/18
= 8/2 ÷ 18/2
= 4/9
= 4 : 9 (another equivalent ratio)
Thus, 4 : 9 is an equivalent ratio of 8 : 18.
So, here the two equivalent ratios of 8 : 18 are 16 : 36 and 4 : 9.
Question: Can you find two equivalent-ratios of 4 : 5.
Solution: In order to find two equivalent ratios of 4 : 5 it is important to apply multiplication method only to get the result in the integer form.
First we need to write the given ratio as fraction,
= 4/5
= (4 × 2)/(5 × 2)
= 8/10
So, 8 : 10 is one equivalent ratio,
In the similar manner, we need to write the given ratio 4 : 5 as fraction to get another equivalent ratio, where we get:
= 4/5
= (4 × 3)/(5 × 3)
= 12/15 is another equivalent ratio
So, the two equivalent ratios of 4 : 5 are 8 : 10 and 12 : 15.
Question: Find three equivalent ratios of 3 : 8?
Solution:
Here 3 : 8 = 3/8 = (3 × 2)/(8 × 2), [Multiplying both numerator and denominator by 2]
= 6/16
So, the first equivalent ratio is 6 : 16
Now 3 : 8 = 3/8 = (3 × 4)/(8 × 4), [Multiplying both numerator and denominator by 4]
= 12/32
So, the second equivalent ratio is 12 : 32
Next 3 : 8 = 3/8 = (3 × 6)/( 8 × 6) [Multiplying both numerator and denominator by 6]
= 18/48
So, the third equivalent ratio is 18 : 48
The three equivalent ratios of 3 : 8 include 6 : 16, 12 : 32, and 18 : 48.
Important Chapters:
Similar Questions
Two equivalent ratio to 3 : 4 are ______.
View SolutionWhich among these is not an equivalent ratio of 4: 9?
View SolutionIntroduction|Ratio|Question Practice|Equivalent Ratio|Proportion|OMR
View SolutionEQUIVALENT RATIOS A ratio obtained by multiplying or dividing the numerator and denominator of a given ratio by the same number is called an equivalent ratio.
View SolutionFill in the following blanks
1518=□6=10□=□30
[Are these equivalents ratio ?]View SolutionEquivalent Fractions
View SolutionWhat is the ratio of equivalent resistance of series combination of n equal resistance to the equivalent resistance in parallel combination of these n resistances ?
View SolutionTwo identical resistors are first connected in series and then in parallel. Find the ratio of equivalent resistances in two cases.
View SolutionThe ratio of equivalent resistance of the combined resistances when the switch is closed to switch is open is 8:x. Find value of x
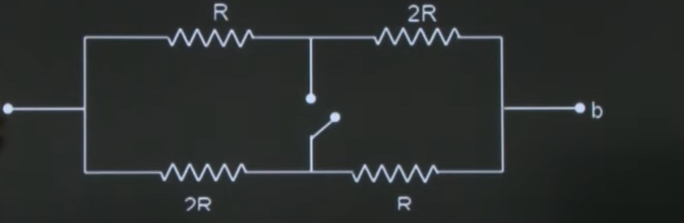 View Solution
View SolutionDerive expression for equivalent resistance of a parallel combination of resistances.
(b) Calculate the ratio of equivalent resistance for a series combination of n number of identical resistors to the parallel combination of the same type of n number of resistors.View Solution

