Sunrays pass through a pinhole in the roof of a hut and produce an elliptical spot on the floor. The minor and major axes of the spot are 6 cm and 12 cm respectively. The angle subtended by the diameter of the sun at our eye is 0.5°. Calculate the height of the roof.
The correct Answer is:3.44cm
Step by step video, text & image solution for Sunrays pass through a pinhole in the roof of a hut and produce an elliptical spot on the floor. The minor and major axes of the spot are 6 cm and 12 cm respectively. The angle subtended by the diameter of the sun at our eye is 0.5°. Calculate the height of the roof. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ARIHANTCHAPTER - GEOMETRICAL OPTICSEXERCISE - Level 3
9 Videos
- GEOMETRICAL OPTICSBOOK - ARIHANTCHAPTER - GEOMETRICAL OPTICSEXERCISE - Level 3
9 Videos
- ELECTROSTATICSBOOK - ARIHANTCHAPTER - ELECTROSTATICSEXERCISE - Level 3
59 Videos
- MAGNETIC EFFECT OF CURRENTBOOK - ARIHANTCHAPTER - MAGNETIC EFFECT OF CURRENTEXERCISE - Magnetic effect of Current
45 Videos
Similar Questions
When Sun's rays pass through small holes in the ventilator of a room, they produce elliptical spots on the floor. The major and minor axes of the ellipses are a=4.9cm and b=4.6cm respectively. What is the height of the room? The angular dimensions of the Sun's disc are β=1100rad.
View SolutionA telescope consists of two lenses of focal lengths 0.3mand3cm respectively. It is fucussed on moon which subtends an angle of 0.5∘ at the obejctive. Calculate the angle subtended at the eye by the final image in normal adjustment of the telescope.
View Solution
Knowledge Check
Two rings are suspended from the points A and B on the ceiling of a room with the help of strings of length 1.2 m each as shown. The points A and B are separated from each other by a distance AB = 1.2 m. A boy standing on the floor throws a small ball so that it passes through the two rings whose diameter is slightly greater than the ball. At the moment when the boy throws the ball, his hand is at a height of 1.0 m above the floor. A stop watch which is switched on at the moment of throwing the ball reads 0.2 s when the ball passes through the first ring and 0.6 s when it passes through the second ring.
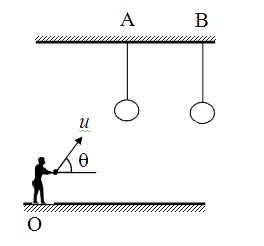
The angle of projection of the ball is:Atan−134Btan−143Ctan−112Dtan−135A rectangular toroid has 1000 turns. The ratio of the outer to inner diameter is 1.6 and its height is 5.0 cm. Find the flux through this toroid, if current passing through it is 1.7 A.
A8μWbB6μWbC8WbD6Wb
A well collimated parallel pencil of cathode rays falls through a potential difference 3kV & enters the spacing between two parallel metallic plates, parallel to their length the spacing between the plates being 0.5cm. The pencil strikes a fluorescent screen, mounted perpendicular to the length of the plates at the farther end of the plates & produces fluorescent spot. if now a potential difference of 3V is applied across the two plates, calculate the linear deflection of the spot on the screen. Given the length of the plates is 10 cm.
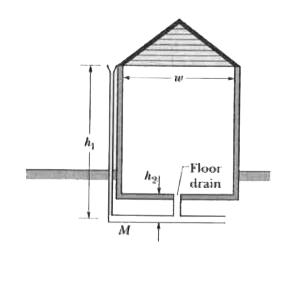
View SolutionA very simplified schematic of the rain drainage system for a home is shown in Fig. Rain falling on the slanted roof runs off into gutters around the roof edge, it then drains through downspouts (only one is shown) into a main drainage pipe M below the basement, which carries the water to an even larger pipe below the street. In Fig. a floor drain in the basement is also connected to drainage pipe M. Suppose the following apply:
(1) the downspouts have height h1 = 11 m,
(2) the floor drain has height h2 = 1.2 m,
(3) pipe M has radius 2.0 cm,
(4) the house has side width w = 40 m and front length L = 70 m,
(5) all the water striking the roof goes through pipe M,
(6) the initial speed of the water in a downspout is negligible, and
(7) the wind speed is negligible (the rain falls vertically).
At what rainfall rate, in centimeters per hour, will water from pipe M reach the height of the floor drain and threaten to flood the basement?
View SolutionA convex lens of focal length 15 cm and a concave mirror of focal length 30 cm are kept with their optic axis PQ and RS parallel but separated in vertical directiion by 0.6 cm as shown . The distance between the lens and mirror is 30 cm . An upright object AB of height 1.2 cm is placed on the optic axis PQ of the lens at a distance of 20 cm from the lens . if A'B' is the image after refraction from the lens and the reflectiion from the mirror , find the distance of A'B' from the pole of the mirror and obtain its magnification . Also locate positions of A' and B' with respect to the optic axis RS.
_E01_247_Q01.png)
View Solution(i) An organ pipe has one end closed and at the other end there is a vibrating diaphragm. The diaphragm is a pressure node. The pipe resonates when the frequency of the diaphragm is 2 KHz. Distance between adjacent nodes is 8.0 cm. When the frequency is slowly reduced, the pipe again resonates at 1.2 KHz.
(a) Find the length of the tube.
(b) Find the next frequency above 2 KHz at which the pipe resonates.
(ii) The figure shows an arrangement for measuring the speed of sound in air. A glass tube is fitted with a movable piston that allows the indicated length L to be adjusted. There is enough gap between the piston and the tube wall to allow the air to pass through it. A speaker is placed near the open end of the tube. A microphone is placed close to the speaker and it is connected to a waveform display. The display is a pure sinusoidal waveform making 750 oscillations in 5 s. Initially, the piston is held at end A and is then slowly pulled back. Loud sound is produced by the tube when L=50cm and L=157cm. Calculate the speed of sound in air.

View SolutionOn seeing with unaided eye, the visual angle of moon at the eye is 0.6∘. The focal lengths of the objective and the eyepiece of a telescope are respectively 200 cm and 5 cm. What will the visual angle on seeing through the telescope?
View SolutionLiquid rises to a height of 5.0 cm in a capillary tube and mercury falls to a depth of 2.0 cm in the same capillary tube. If the density of liquid is 1.2g/cc, of mercury is 13.6g/cc and angles of contact of liquid and mercury with capillary tube are 0∘ and 135∘ respectively. find the ratio of the surface tension for mercury and liquid.
View Solution
