Solve the cryptarithm : ¯¯¯¯¯¯AB+¯¯¯¯¯¯BA=¯¯¯¯¯¯¯¯¯DAD
To solve the cryptarithm ¯¯¯¯¯¯¯¯AB+¯¯¯¯¯¯¯¯BA=¯¯¯¯¯¯¯¯¯¯¯¯¯DAD, we will follow these steps:
Step 1: Understand the notation
The notation ¯¯¯¯¯¯¯¯AB represents a two-digit number where A is the tens digit and B is the units digit. Therefore, we can express it mathematically as:
¯¯¯¯¯¯¯¯AB=10A+B
Similarly, for ¯¯¯¯¯¯¯¯BA:
¯¯¯¯¯¯¯¯BA=10B+A
And for ¯¯¯¯¯¯¯¯¯¯¯¯¯DAD, which is a three-digit number:
¯¯¯¯¯¯¯¯¯¯¯¯¯DAD=100D+10A+D=101D+10A
Step 2: Set up the equation
Now we can set up the equation based on the given cryptarithm:
(10A+B)+(10B+A)=101D+10A
This simplifies to:
11A+11B=101D+10A
Step 3: Rearranging the equation
We can rearrange the equation:
11A+11B−10A=101D
This simplifies to:
A+11B=101D
Step 4: Analyze the maximum values
Since ¯¯¯¯¯¯¯¯AB and ¯¯¯¯¯¯¯¯BA are both two-digit numbers, their maximum value is 99. Therefore, the maximum value of ¯¯¯¯¯¯¯¯¯¯¯¯¯DAD is:
99+99=198
This means DAD must also be less than or equal to 198.
Step 5: Determine the value of D
Since DAD is a three-digit number, D cannot be 0. The maximum value for D must be 1 because if D were 2, DAD would exceed 200. Thus, we conclude:
D=1
Step 6: Substitute D back into the equation
Substituting D=1 into the equation:
A+11B=101×1
This simplifies to:
A+11B=101
Step 7: Find possible values for A and B
Now we need to find values for A and B such that:
- A and B are digits (0-9)
- The equation A+11B=101 holds true.
Step 8: Solve for B
Rearranging gives:
A=101−11B
To find valid values for B:
- If B=9:
A=101−11×9=101−99=2
- If B=8 or lower, A would exceed 9, which is not allowed.
Step 9: Conclusion
Thus, the only valid solution is:
A=2,B=9,D=1
Final Answer
The values are:
- A=2
- B=9
- D=1
The notation ¯¯¯¯¯¯¯¯AB represents a two-digit number where A is the tens digit and B is the units digit. Therefore, we can express it mathematically as:
¯¯¯¯¯¯¯¯AB=10A+B
Similarly, for ¯¯¯¯¯¯¯¯BA:
¯¯¯¯¯¯¯¯BA=10B+A
And for ¯¯¯¯¯¯¯¯¯¯¯¯¯DAD, which is a three-digit number:
¯¯¯¯¯¯¯¯¯¯¯¯¯DAD=100D+10A+D=101D+10A
Now we can set up the equation based on the given cryptarithm:
(10A+B)+(10B+A)=101D+10A
This simplifies to:
11A+11B=101D+10A
We can rearrange the equation:
11A+11B−10A=101D
This simplifies to:
A+11B=101D
Since ¯¯¯¯¯¯¯¯AB and ¯¯¯¯¯¯¯¯BA are both two-digit numbers, their maximum value is 99. Therefore, the maximum value of ¯¯¯¯¯¯¯¯¯¯¯¯¯DAD is:
99+99=198
This means DAD must also be less than or equal to 198.
Since DAD is a three-digit number, D cannot be 0. The maximum value for D must be 1 because if D were 2, DAD would exceed 200. Thus, we conclude:
D=1
Substituting D=1 into the equation:
A+11B=101×1
This simplifies to:
A+11B=101
Now we need to find values for A and B such that:
- A and B are digits (0-9)
- The equation A+11B=101 holds true.
Rearranging gives:
A=101−11B
To find valid values for B:
- If B=9:
A=101−11×9=101−99=2
- If B=8 or lower, A would exceed 9, which is not allowed.
Thus, the only valid solution is:
A=2,B=9,D=1
The values are:
- A=2
- B=9
- D=1
Similar Questions
Knowledge Check
If ABCDEF is a regular hexagon inscribed in a circle with centre O, then ¯¯¯¯¯¯AB+¯¯¯¯¯¯AC+¯¯¯¯¯¯AD+¯¯¯¯¯¯AE+¯¯¯¯¯¯AF=
A¯¯¯¯¯¯AOB5¯¯¯¯¯¯AOC6¯¯¯¯¯¯AOD8¯¯¯¯¯¯AO¯¯¯¯¯¯AB−¯¯¯¯¯¯CB+¯¯¯¯¯¯DA+2¯¯¯¯¯¯CD=
A¯¯¯¯¯¯CBB6¯¯¯¯¯¯OCC¯¯¯¯¯¯CDD¯¯¯¯¯¯ACABCDE is a pentagon. The resultant of the vectors ¯¯¯¯¯¯AB,¯¯¯¯¯¯AE,¯¯¯¯¯¯BC,¯¯¯¯¯¯DC,¯¯¯¯¯¯EDand¯¯¯¯¯¯AC in terms of ¯¯¯¯¯¯AC is
A4¯¯¯¯¯¯ACB2¯¯¯¯¯¯ACC3¯¯¯¯¯¯ACD5¯¯¯¯¯¯AC
If ¯a,¯b,¯c be three vecotrs such that ¯aׯb=¯c,¯bׯc=¯a,¯cׯa=¯b, then
View Solution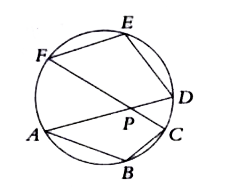
In the above figure (not to scale) ¯¯¯¯¯¯AB,¯¯¯¯¯¯BC,¯¯¯¯¯¯CF,¯¯¯¯¯¯DE,and¯¯¯¯¯¯FE are chords of the circle . If ∠ABC=100∘and∠FED=110∘ , then ∠FPA= _______.
View SolutionIf ABCDE is a pentago, then ¯¯¯¯¯¯AB+¯¯¯¯¯¯BC+¯¯¯¯¯¯CD+¯¯¯¯¯¯DE−¯¯¯¯¯¯AE=
View SolutionIf ABCD is a quadrilateral, then 2¯¯¯¯¯¯AB+3¯¯¯¯¯¯BC+2¯¯¯¯¯¯CD+¯¯¯¯¯¯DA+¯¯¯¯¯¯CA+¯¯¯¯¯¯DB=
View SolutionIf ABCDEF is a regular hexagon, then ¯¯¯¯¯¯AB+¯¯¯¯¯¯AC+¯¯¯¯¯¯AE+¯¯¯¯¯¯AF=
View Solution