If 2x=3y and 4y=5z, then x:z= (a) 4:3 (b) 8:15 (c) 3:4 (d) 15:8
To solve the problem, we need to find the ratio x:z given the equations 2x=3y and 4y=5z.
1. From the first equation 2x=3y:
- We can express x in terms of y:
x=3y2
2. From the second equation 4y=5z:
- We can express z in terms of y:
z=4y5
3. Now, we need to find the ratio x:z:
- This can be expressed as:
xz=3y24y5
4. Simplifying the fraction:
- To simplify 3y24y5, we multiply by the reciprocal of the denominator:
xz=3y2×54y
- The y cancels out:
=3×52×4=158
5. Thus, the ratio x:z is:
x:z=15:8
Final Answer:
The ratio x:z is 15:8, which corresponds to option (d).
- We can express x in terms of y:
x=3y2
- We can express z in terms of y:
z=4y5
- This can be expressed as:
xz=3y24y5
- To simplify 3y24y5, we multiply by the reciprocal of the denominator:
xz=3y2×54y
- The y cancels out:
=3×52×4=158
x:z=15:8
The ratio x:z is 15:8, which corresponds to option (d).
Topper's Solved these Questions
Similar Questions
If 2x=3y and 4y=5z, then x:z= (a) 4:3 (b) 8:15 (c) 3:4 (d) 15:8
View SolutionIf x:y=2:3 andy:z=2:3, then x:z= 2:3 (b) 3:4 (c) 5:7 (d) 4:9
View SolutionIf 2x+y=5 and 3x−4y=2 , then the value of 2xy is (a) 4 (b) 6 (c) 8 (d) 10
View SolutionIf 2x+y=17; y+2z=15 and x+y=9 , then what is the value of 4x+3y+z ? (a) 41 (b) 43 (c) 45 (d) 55 (e) None of these
View SolutionIf 3x−4y+z=7; 2x−z+3y=19; x+2y+2z=24 , then what is the value of z ? (a) 4 (b) 5 (c) 6 (d) 8
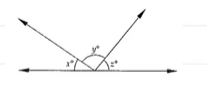
View SolutionIn Figure, if yx=5 and zx=4, then the value of x is (a) 80 (b) 180 (c) 120 (d) 150
View SolutionIf x(2x+y+z)=y(x+2y+z)=z(x+y+2z)=a , then find a if x+y+z≠0 . (a)13 (b) 14 (c) 12 (d) 18
View SolutionIf 2x+3y=31, y−z=4 and x+2z=11 , then what is the value of x+y+z ? (a) 12 (b) 13 (c) 15 (d) 16
View SolutionThe number of solutions of the system of equations: 2x+y−z=7, x−3y+2z=1, x+4y−3z=5 is (a) 3 (b) 2 (c) 1 (d) 0
View SolutionIf 32x+43y=35z, then z will be
View Solution
