If both the angles are acute and sin(3x+20∘)=cos(2x−40∘), find x
To solve the equation sin(3x+20∘)=cos(2x−40∘), we can use the trigonometric identity that relates sine and cosine:
Step 1: Use the identity
We know that:
sinA=cos(90∘−A)
Thus, we can rewrite the cosine term:
cos(2x−40∘)=sin(90∘−(2x−40∘))=sin(130∘−2x)
Step 2: Set the sine terms equal
Now we can set the two sine terms equal:
sin(3x+20∘)=sin(130∘−2x)
Step 3: Compare angles
Since both angles are equal, we can set up the equation:
3x+20∘=130∘−2x
Step 4: Solve for x
Now, we will solve for x:
1. Add 2x to both sides:
3x+2x+20∘=130∘
This simplifies to:
5x+20∘=130∘
2. Subtract 20∘ from both sides:
5x=130∘−20∘
This simplifies to:
5x=110∘
3. Divide by 5:
x=110∘5=22∘
Final Answer
Thus, the value of x is:
22∘
We know that:
sinA=cos(90∘−A)
Thus, we can rewrite the cosine term:
cos(2x−40∘)=sin(90∘−(2x−40∘))=sin(130∘−2x)
Now we can set the two sine terms equal:
sin(3x+20∘)=sin(130∘−2x)
Since both angles are equal, we can set up the equation:
3x+20∘=130∘−2x
Now, we will solve for x:
1. Add 2x to both sides:
3x+2x+20∘=130∘
This simplifies to:
5x+20∘=130∘
5x=130∘−20∘
This simplifies to:
5x=110∘
x=110∘5=22∘
Thus, the value of x is:
22∘
Topper's Solved these Questions
Similar Questions
Knowledge Check
In a right triangle, one of the acute angles is cos(π3),anccos(π3)=sinx. What is the measure of x ?
Aπ12Bπ6Cπ3D2π3Angle x is one of the acute angles in a right triangle. If the measure of angles is 30∘, what is the value of (sinx)2+(cosx)2 ?
A14B12C1D2
If the angle θ is acute, then the acute angle between x2(cosθ−sinθ)+2xycosθ+y2(cosθ+sinθ)=0, is
View SolutionEvaluate : ∫excos2x(cosx−3sinx)dx
View SolutionIf 4cos2x=3andx is an acute angle find the value of :
(i) x
(ii) cos2x+cot2x
(iii) cos 3x
(iv) sin 2x
View SolutionIn the given figure ∠B=90∘and∠ADB=x∘, Find :
(i) sin∠CAB
(ii)cos2C∘+sin2C∘
(iii) tanx∘−cosx∘+3sinx∘
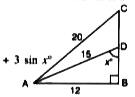
View SolutionIf x= 20∘ Evaluate :
12sin3x2cos3x+5tan(2x+5∘)−3cot23x.
View SolutionFind the number of solutions of the equations
(sinx−1)3+(cosx−1)3+(sinx)3=(2sinx+cosx−2)3 in (0,2π).
View Solution
