For all θ,tanθ+cosθ+tan(−θ)+cos(−θ)=
0
2tanθ
2cosθ
2(tanθ+cosθ)
The correct Answer is:C
To solve the expression tanθ+cosθ+tan(−θ)+cos(−θ), we can follow these steps:
Step 1: Identify the properties of trigonometric functions
We know that:
- tan(−θ)=−tan(θ) (odd function)
- cos(−θ)=cos(θ) (even function)
Step 2: Substitute the properties into the expression
Using these properties, we can rewrite the expression:
tanθ+cosθ+tan(−θ)+cos(−θ)=tanθ+cosθ+(−tanθ)+cosθ
Step 3: Simplify the expression
Now, let's simplify the expression:
tanθ−tanθ+cosθ+cosθ=0+2cosθ=2cosθ
Final Answer
Thus, the expression simplifies to:
tanθ+cosθ+tan(−θ)+cos(−θ)=2cosθ
We know that:
- tan(−θ)=−tan(θ) (odd function)
- cos(−θ)=cos(θ) (even function)
Using these properties, we can rewrite the expression:
tanθ+cosθ+tan(−θ)+cos(−θ)=tanθ+cosθ+(−tanθ)+cosθ
Now, let's simplify the expression:
tanθ−tanθ+cosθ+cosθ=0+2cosθ=2cosθ
Thus, the expression simplifies to:
tanθ+cosθ+tan(−θ)+cos(−θ)=2cosθ
Topper's Solved these Questions
Similar Questions
If cosθ=0.75,tanθ=
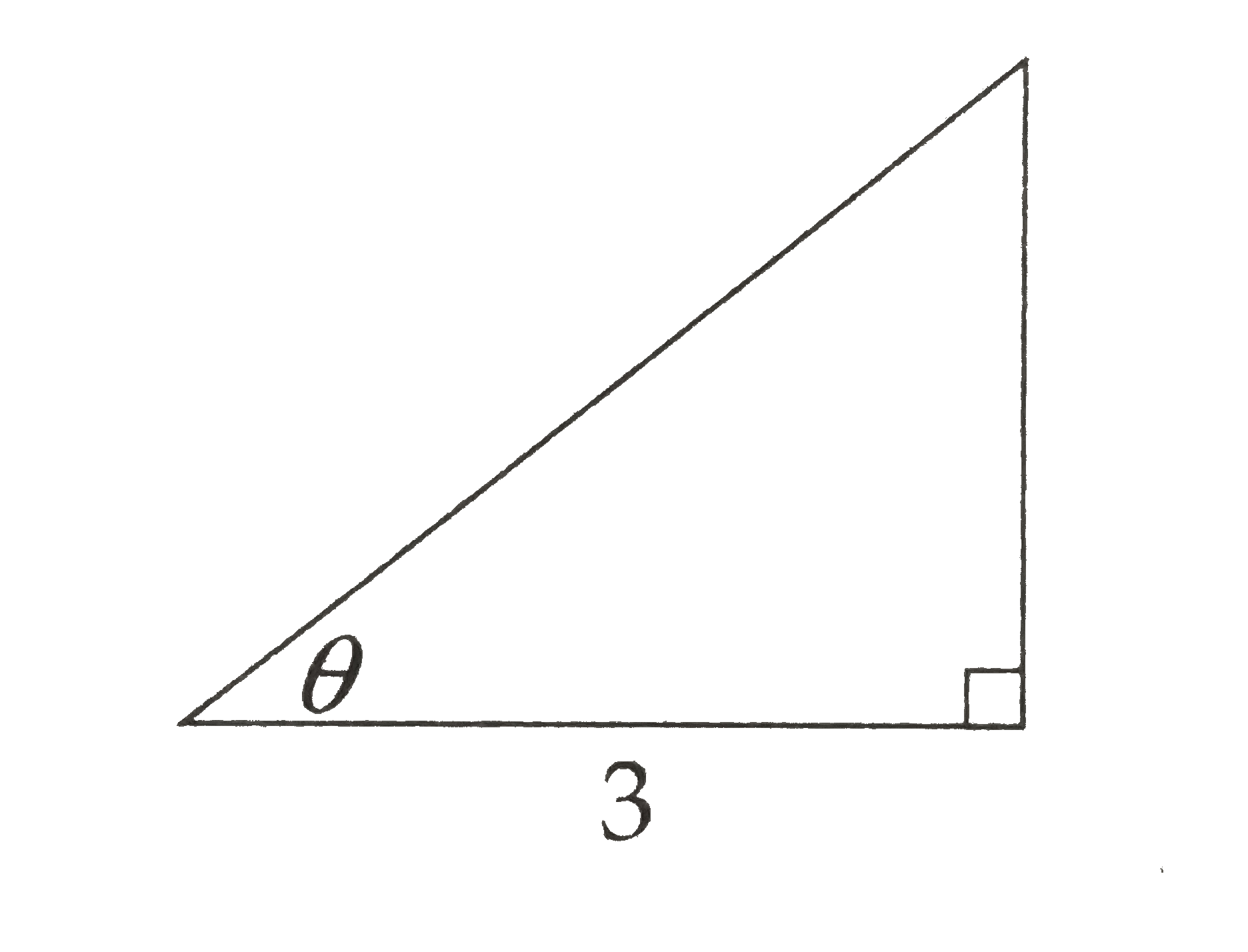
View SolutionIf θ lies in the first quadrant and 5tanθ=4, then 5sinθ−3cosθsinθ+2cosθ=
View SolutionSolve tanθ+tan2θ+tanθ⋅tan2θ⋅tan3θ=1
View SolutionIf tanθ=pq then psinθ−qcosθpsinθ+qcosθ=
View SolutionIf tanθ=ab then bcos2θ+asin2θ=
View SolutionProve that: tan8θ−tan6θ−tan2θ=tan8θ tan6θ tan2θ.
View SolutionProve that : 1+sinθ−cosθ1+sinθ+cosθ=tan(θ2)
View SolutionIf 5tanθ=4 , show that 5sinθ−3cosθ5sinθ+3cosθ=17
View Solutionθ1,θ2,θ3 are angles of 1st quadrant if tanθ1=cosθ1,tanθ2=cosecθ2,cosθ3=θ3. Which of the following is not true ?
View SolutionGeneral solution of the equation
tanθ+tan4θ+tan7θ=tanθ⋅tan4θ⋅tan7θisθ=
View Solution
