Triangle ABC is right angled at A.AD is perpendicular to BC. If AB=5 cm, BC=13 cm and AC=12 cm. Find the area of ABC. Also, find the length of AD.
To solve the problem, we will follow these steps:
Step 1: Find the Area of Triangle ABC
The area of a triangle can be calculated using the formula:
Area=12×base×height
In triangle ABC, we can take AB as the base and AC as the height.
Given:
- AB=5cm
- AC=12cm
Substituting the values into the formula:
Area=12×5cm×12cm=12×60cm2=30cm2
Step 2: Find the Length of AD
Since AD is perpendicular to BC, we can use the Pythagorean theorem in two right triangles: ABD and ACD.
Let:
- AD=L
- DC=H
- BD=BC−DC=13cm−H
Using the Pythagorean theorem in triangle ACD:
L2+H2=AC2
Substituting the known values:
L2+H2=122=144(1)
Using the Pythagorean theorem in triangle ABD:
L2+(13−H)2=AB2
Substituting the known values:
L2+(13−H)2=52=25(2)
Step 3: Expand Equation (2)
Expanding equation (2):
L2+(169−26H+H2)=25
Rearranging gives:
L2+H2−26H+169−25=0
L2+H2−26H+144=0(3)
Step 4: Set Equations (1) and (3) Equal
From equations (1) and (3):
144−H2=25−169+26H−H2
This simplifies to:
144=−144+26H
Adding 144 to both sides:
288=26H
Dividing by 26:
H=28826≈11.07cm
Step 5: Substitute H back to find L
Substituting H back into equation (1):
L2=144−H2
Calculating H2:
H2≈(11.07)2≈122.48
Now substituting:
L2=144−122.48≈21.52
Taking the square root:
L≈√21.52≈4.64cm
Final Answers
- The area of triangle ABC is 30cm2.
- The length of AD is approximately 4.64cm.
The area of a triangle can be calculated using the formula:
Area=12×base×height
In triangle ABC, we can take AB as the base and AC as the height.
- AB=5cm
- AC=12cm
Area=12×5cm×12cm=12×60cm2=30cm2
Since AD is perpendicular to BC, we can use the Pythagorean theorem in two right triangles: ABD and ACD.
- AD=L
- DC=H
- BD=BC−DC=13cm−H
L2+H2=AC2
Substituting the known values:
L2+H2=122=144(1)
L2+(13−H)2=AB2
Substituting the known values:
L2+(13−H)2=52=25(2)
Expanding equation (2):
L2+(169−26H+H2)=25
Rearranging gives:
L2+H2−26H+169−25=0
L2+H2−26H+144=0(3)
From equations (1) and (3):
144−H2=25−169+26H−H2
This simplifies to:
144=−144+26H
Adding 144 to both sides:
288=26H
Dividing by 26:
H=28826≈11.07cm
Substituting H back into equation (1):
L2=144−H2
Calculating H2:
H2≈(11.07)2≈122.48
Now substituting:
L2=144−122.48≈21.52
Taking the square root:
L≈√21.52≈4.64cm
- The area of triangle ABC is 30cm2.
- The length of AD is approximately 4.64cm.
Topper's Solved these Questions
Similar Questions
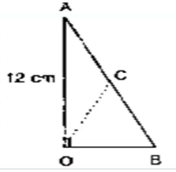
In Figure, ∠AOB=900, AC=BC, OA=12 cm and OC=6.5cm. Find the area of AOB.
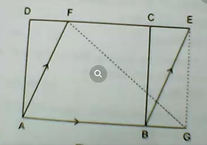
View SolutionIn Figure, ABCD is a rectangle with sides AB=10cmandAD=5cm Find the area of EFG
View SolutionIn Fig.20, ABCD is a parallelogram, DL⊥AB and DM⊥BC. If AB=18 cm , BC=12 cm and DM=9.3 cm, find DL.
View SolutionIn Figure, triangle ABC is right-angled at B. Give that AB=9CM, AC=15CM,andD,E are the mid-points of the sides ABandAC respectively, calculate
The length of BC
(ii) The area of ADE
View SolutionIn Figure, ABCD is a quadrilateral in which diagonal AC=84 cm ; DL⊥AC, BM⊥AC, DL=16.5 cm and BM=12 cm . Find the area of quadrilateral ABCD.
View SolutionIn Fig. 21, ABCD is a parallelogram, DL⊥AB. If AB=20 cm , AD=13 cm and area of the parallelogram is 100 cm2 find AL.
View SolutionABC is a triangle and PQ is a straight line meeting AB in P and AC in Q . If AP=1cm , PB=3cm , AQ=1.5cm , QC=4.5m , prove that area of APQ is one-sixteenth of the area of ABC .
View SolutionIf triangle ABC is similar to triangle DEF such that BC=3CM,EF=4cm and area of triangle ABC=54cm2 . Determine the area of triangle DEF.
View SolutionIf A : B = 3 : 4 and B : C = 12 : 13, then find A : C.
View SolutionIn ΔABC, if angle C=3 angle A, B C=27 and A B=48 , then find the length of the side A C .
View Solution
