Question

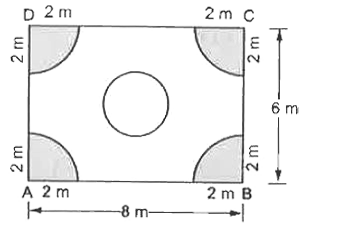
In Fig. 19, the radius of quarter circular
plot taken is 2 m and radius of the flower bed is 2 m. Find the area of the
remaining field.

Explanation

To solve the problem, we first need to find the area of the quarter circular plot and the area of the flower bed, and then we will subtract the area of the flower bed from the area of the quarter circular plot to find the area of the remaining field.

1. Identify the radius of the quarter circular plot and the flower bed:
- Given radius of the quarter circular plot (R) = 2 m
- Given radius of the flower bed (r) = 2 m

2. Calculate the area of the quarter circular plot:
- The formula for the area of a full circle is \( A = \pi R^2 \).
- Since we only need the area of a quarter circle, we will divide the area of the full circle by 4.
- Area of the quarter circular plot = \( \frac{1}{4} \times \pi R^2 = \frac{1}{4} \times \pi (2)^2 = \frac{1}{4} \times \pi \times 4 = \pi \) m².

3. Calculate the area of the flower bed:
- The area of the flower bed is also a circle, so we will use the same formula.
- Area of the flower bed = \( \pi r^2 = \pi (2)^2 = \pi \times 4 = 4\pi \) m².

4. Calculate the area of the remaining field:
- To find the area of the remaining field, subtract the area of the flower bed from the area of the quarter circular plot.
- Area of the remaining field = Area of the quarter circular plot - Area of the flower bed
- Area of the remaining field = \( \pi - 4\pi = -3\pi \) m².

5. Interpret the result:
- Since the area of the remaining field cannot be negative, it indicates that the flower bed occupies more area than the quarter circular plot. Therefore, the remaining area is effectively zero in this context.

Final Answer:
The area of the remaining field is effectively 0 m², as the flower bed occupies the entire area of the quarter circular plot.