The runs scored in a cricket match by 11 players are as follows:6,15,120,50,100,80,10,15,8,10,10 Find the mean, mode and median of this data.
To find the mean, mode, and median of the given data set of runs scored by 11 players, we will follow these steps:
Given Data:
The runs scored by the players are:
6, 15, 120, 50, 100, 80, 10, 15, 8, 10, 10
Step 1: Arrange the Data in Ascending Order
First, we need to arrange the data in increasing order:
Ordered Data:
6, 8, 10, 10, 10, 15, 15, 50, 80, 100, 120
Step 2: Calculate the Mean
The mean is calculated by taking the sum of all observations and dividing it by the number of observations.
Sum of Observations:
6 + 8 + 10 + 10 + 10 + 15 + 15 + 50 + 80 + 100 + 120 = 424
Number of Observations:
There are 11 players.
Mean Calculation:
Mean = Sum of Observations / Number of Observations
Mean = 424 / 11 = 38.54545454545454 (approximately 38.55)
Step 3: Calculate the Median
The median is the middle value of the ordered data. Since we have an odd number of observations (11), the median will be the value at the (n + 1)/2 position.
Position of Median:
(n + 1) / 2 = (11 + 1) / 2 = 12 / 2 = 6
Median Value:
The 6th value in the ordered list is 15.
Step 4: Calculate the Mode
The mode is the value that appears most frequently in the data set.
Frequency of Each Value:
- 6: 1 time
- 8: 1 time
- 10: 3 times
- 15: 2 times
- 50: 1 time
- 80: 1 time
- 100: 1 time
- 120: 1 time
Mode Value:
The number that appears most frequently is 10 (which appears 3 times).
Final Results:
- Mean: 38.55
- Median: 15
- Mode: 10
The runs scored by the players are:
6, 15, 120, 50, 100, 80, 10, 15, 8, 10, 10
First, we need to arrange the data in increasing order:
6, 8, 10, 10, 10, 15, 15, 50, 80, 100, 120
The mean is calculated by taking the sum of all observations and dividing it by the number of observations.
6 + 8 + 10 + 10 + 10 + 15 + 15 + 50 + 80 + 100 + 120 = 424
There are 11 players.
Mean = Sum of Observations / Number of Observations
Mean = 424 / 11 = 38.54545454545454 (approximately 38.55)
The median is the middle value of the ordered data. Since we have an odd number of observations (11), the median will be the value at the (n + 1)/2 position.
(n + 1) / 2 = (11 + 1) / 2 = 12 / 2 = 6
The 6th value in the ordered list is 15.
The mode is the value that appears most frequently in the data set.
- 6: 1 time
- 8: 1 time
- 10: 3 times
- 15: 2 times
- 50: 1 time
- 80: 1 time
- 100: 1 time
- 120: 1 time
The number that appears most frequently is 10 (which appears 3 times).
- Mean: 38.55
- Median: 15
- Mode: 10
Topper's Solved these Questions
Similar Questions
Knowledge Check
The runs scored in a cricket match by 11 players is as follows:6, 15, 120, 50, 100, 80, 10, 15, 8, 10, 15Find the mean, mode and median of this data. Are the three same?
A mean = 39, median = 15, mode = 15B mean = 40, median = 16, mode = 16C mean = 38, median = 11, mode = 11D mean = 33, median = 12, mode = 12
The runs scored in a cricket match by 11 players are as follows :
9, 15, 121, 51, 101, 81, 50, 16, 82, 11, 11
Find the mean, mode and median respectively of this data
View SolutionFind the mean, median and mode of the following data.
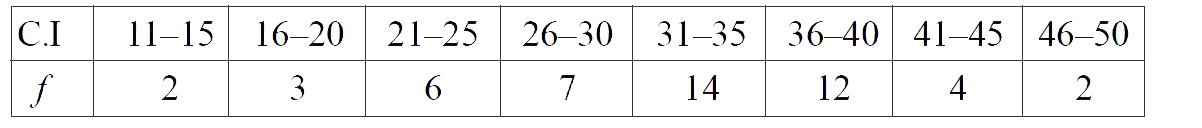
View SolutionFind the mean, median and mode of the following data
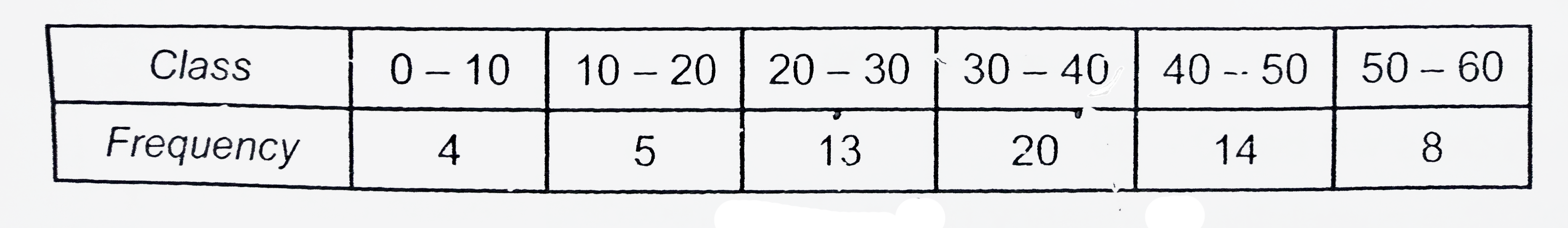
View SolutionFind the mean, median and mode of the following data:
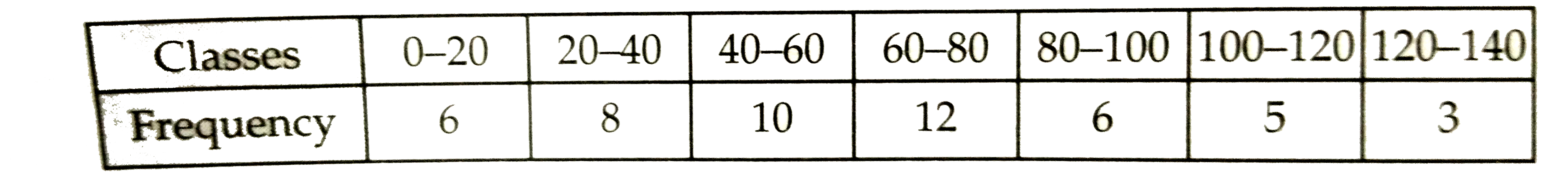
View SolutionFind the mean, median and mode of the following data :
View SolutionFind the mean, median and mode of the following data :

View SolutionFind the mean, median, and mode for the follow ing data.

View Solution

