In a parallelogram the sum of any two adjacent angles is
To prove that in a parallelogram the sum of any two adjacent angles is 180∘, we can follow these steps:
1. Identify the Parallelogram:
Let’s consider a parallelogram ABCD, where AD is parallel to BC and AB is parallel to CD.
2. Understand the Properties of Parallel Lines:
Since AD is parallel to BC, and AB is a transversal line that intersects both AD and BC, we can use the properties of angles formed by a transversal.
3. Apply the Consecutive Interior Angles Theorem:
According to the Consecutive Interior Angles Theorem, when a transversal intersects two parallel lines, the sum of the interior angles on the same side of the transversal is 180∘.
4. Calculate Angle A and Angle B:
Since AB is a transversal, we have:
Angle A+Angle B=180∘
This is because they are consecutive interior angles.
5. Calculate Angle B and Angle C:
Similarly, since CD is also parallel to AB and BC is the transversal:
Angle B+Angle C=180∘
6. Calculate Angle C and Angle D:
Now, considering the transversal AD:
Angle C+Angle D=180∘
7. Calculate Angle D and Angle A:
Finally, using the transversal BC:
Angle D+Angle A=180∘
8. Conclusion:
Thus, we have shown that the sum of any two adjacent angles in a parallelogram is 180∘:
- Angle A + Angle B = 180∘
- Angle B + Angle C = 180∘
- Angle C + Angle D = 180∘
- Angle D + Angle A = 180∘
Let’s consider a parallelogram ABCD, where AD is parallel to BC and AB is parallel to CD.
Since AD is parallel to BC, and AB is a transversal line that intersects both AD and BC, we can use the properties of angles formed by a transversal.
According to the Consecutive Interior Angles Theorem, when a transversal intersects two parallel lines, the sum of the interior angles on the same side of the transversal is 180∘.
Since AB is a transversal, we have:
Angle A+Angle B=180∘
This is because they are consecutive interior angles.
Similarly, since CD is also parallel to AB and BC is the transversal:
Angle B+Angle C=180∘
Now, considering the transversal AD:
Angle C+Angle D=180∘
Finally, using the transversal BC:
Angle D+Angle A=180∘
Thus, we have shown that the sum of any two adjacent angles in a parallelogram is 180∘:
- Angle A + Angle B = 180∘
- Angle B + Angle C = 180∘
- Angle C + Angle D = 180∘
- Angle D + Angle A = 180∘
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
In a parallelogram ABCD, the sum of any two consecutive angles is 180∘ and opposite angles are equal.
In a parallelogram ABCD,∠D=115∘ , determine the measure of ∠Aand∠BA∠A=85∘,∠B=115∘B∠A=65∘,∠B=65∘C∠A=65∘,∠B=115∘D∠A=75∘,∠B=105∘ - Question 2 - Select One
In a parallelogram ABCD, the sum of any two consecutive angles is 180degree and opposite angles are equal.
In the given figure, find ∠A in the parallelogram .
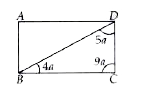 A90∘B60∘C30∘D110∘
A90∘B60∘C30∘D110∘ - Question 3 - Select One
In a parallelogram ABCD, the sum of any two consecutive angles is 180∘ and opposite angles are equal.
Find the value of ∠Qand∠P , if ∠P=10aand∠R=50∘ in a parallelogram PQRS.A∠=50∘,∠P=130∘B∠Q=130∘,∠P=50∘C∠Q=100∘,∠P=120∘D∠Q=50∘+∠P=100∘
In a parallelogram ABCD , prove that sum of any two consecutive angles is 180∘
View SolutionIn a parallelogram, the bisectors of any two consecutive angles intersect at right angle.
View SolutionIn a parallelogram, the bisectors of any two consecutive angles intersect at right angle.
View SolutionIn a parallelogram, two adjacent angles are supplementary.
View SolutionOne angle of a parallelogram is 30∘ more than twice its adjacent angles. Find the measure of its adjacent angle.
View Solution

