Construct a quadrilateral ABCD in which BC=4cm, CA=5.6cm, AD=4.5cm, CD=5cm and BD=6.5cm.
To construct the quadrilateral ABCD with the given dimensions, follow these steps:
Step-by-Step Construction:
1. Draw Line Segment BC:
- Using a ruler, draw a line segment BC that measures 4 cm. Label the endpoints as B and C.
Hint: Ensure you measure accurately using the ruler for precise length.
2. Draw Circle with Center B:
- Using a compass, place the pointer at point B and draw a circle with a radius of 6.5 cm (the length of BD). This circle will help locate point D.
Hint: Keep the compass steady while drawing the circle to maintain a perfect round shape.
3. Draw Circle with Center C:
- Now, place the compass pointer at point C and draw another circle with a radius of 5 cm (the length of CD). This circle will help locate point D as well.
Hint: Make sure the compass width remains unchanged while drawing the second circle.
4. Locate Point D:
- The intersection of the two circles (from steps 2 and 3) gives you point D. Mark the intersection point as D.
Hint: There may be two intersection points; choose the one that allows you to construct the quadrilateral correctly.
5. Draw Line Segment AD:
- Using a ruler, draw a line segment AD that measures 4.5 cm. Start from point A (which will be determined next) and end at point D.
Hint: Measure carefully to ensure that the length is exactly 4.5 cm.
6. Draw Circle with Center A:
- Place the compass pointer at point A and draw a circle with a radius of 5.6 cm (the length of AC). This will help locate point C.
Hint: Ensure the compass is set to the correct measurement before drawing.
7. Locate Point C:
- The intersection of the circle drawn from point A and the line segment BC will give you point C. Mark this intersection as C.
Hint: If the intersection is not clear, double-check your measurements and the position of point A.
8. Draw Line Segment AC:
- Using a ruler, connect point A to point C to complete the quadrilateral.
Hint: Ensure that the line is straight and connects both points clearly.
9. Draw Line Segment BD:
- Finally, connect point B to point D with a straight line.
Hint: Use a ruler for a straight connection to maintain the shape of the quadrilateral.
Final Check:
- Ensure that all sides are correctly measured as per the question: BC = 4 cm, CA = 5.6 cm, AD = 4.5 cm, CD = 5 cm, and BD = 6.5 cm.
- Using a ruler, draw a line segment BC that measures 4 cm. Label the endpoints as B and C.
- Using a compass, place the pointer at point B and draw a circle with a radius of 6.5 cm (the length of BD). This circle will help locate point D.
- Now, place the compass pointer at point C and draw another circle with a radius of 5 cm (the length of CD). This circle will help locate point D as well.
- The intersection of the two circles (from steps 2 and 3) gives you point D. Mark the intersection point as D.
- Using a ruler, draw a line segment AD that measures 4.5 cm. Start from point A (which will be determined next) and end at point D.
- Place the compass pointer at point A and draw a circle with a radius of 5.6 cm (the length of AC). This will help locate point C.
- The intersection of the circle drawn from point A and the line segment BC will give you point C. Mark this intersection as C.
- Using a ruler, connect point A to point C to complete the quadrilateral.
- Finally, connect point B to point D with a straight line.
- Ensure that all sides are correctly measured as per the question: BC = 4 cm, CA = 5.6 cm, AD = 4.5 cm, CD = 5 cm, and BD = 6.5 cm.
Topper's Solved these Questions
Similar Questions
In Fig. 10.57, a circle is inscribed in a quadrilateral ABCD in which ∠B=90o . If AD=23cm , AB=29cm and DS=5cm , find the radius r of the circle. (FIGURE)
View SolutionWhich of the following pairs of triangles are congruent? If they are congruent, write out the pairs of equal angles. ABC:AB=3cm, BC=4cm, CA=2 cm DEF:DE=2 cm, EF=3 cm and FD=4 cm PQR:PQ=17 cm, QR=15 cm, PR=18 cm DEF:DE=18 cm, EF=17 cm, DF=15 cm .
View SolutionIn Figure, ABCD is a quadrilateral in which diagonal AC=84 cm ; DL⊥AC, BM⊥AC, DL=16.5 cm and BM=12 cm . Find the area of quadrilateral ABCD.
View SolutionCalculate the area of the quadrilateral ABCD as shown in Fig.33, given that BD=42 cm , AC=28 cm , OD=12 cm and AC⊥BD .
View SolutionIn a ABC, D and E are points on the sides AB and AC respectively. For each of the following cases show that DEBC : AB=10.8cm, BD=4.5 cm, AC=4.8cm and AE=2.8cm (ii) AD=5.7cm, BD=9.5cm, AE=3.3cm and EC=5.5cm .
View SolutionIn a ABC , D and E are points on AB and AC respectively such that DE/BC . If AD=2.4cm, AE=3.2cm, DE=2cm and BC=5cm , find BD and CE .
View SolutionFind the area of the following polygon, if AL=10cm, AM=20cm, AN=50cm, AO=60cm and AD=90cm.
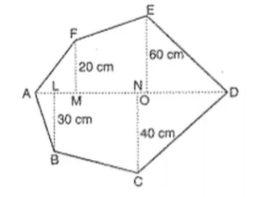 View Solution
View SolutionIn Figure, ABCD is a trapezium in which AB=7cm, AD=BC=5cm, DC=x cm, and distance between AB and DC is 4cm . Find the value of x and area of trapezium ABCD
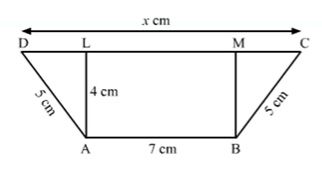 View Solution
View SolutionIn a ABC, D and E are points on the sides AB and AC respectively. For each of the following cases show that DEBC : AB=12 cm, AD=8 cm, AE=12 cm and AC=18 cm . (ii) AB=5.6 cm, AD=1.4 cm, AC=7.2 cm and AE=1.8 cm
View SolutionIn a parallelogram ABCD diagonals AC and BD intersect at O and AC=6.8 cm and BD=5.6cm . Find the measures of OC and OD
View Solution

