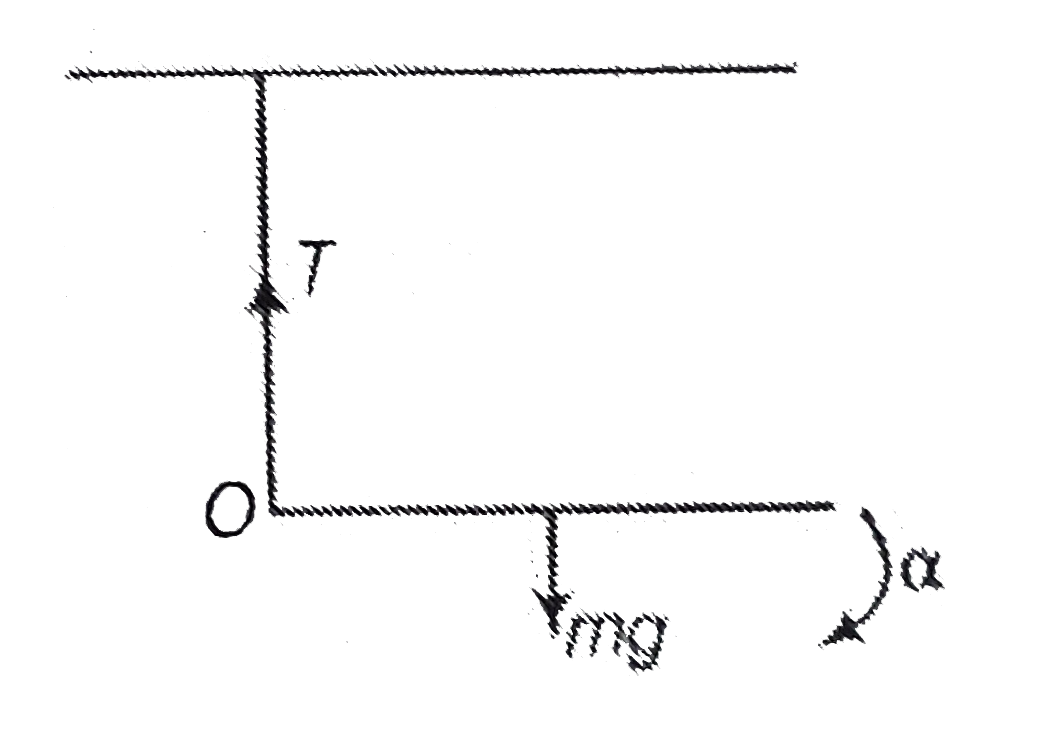
A horizontal weightless rod of length 3l is suspended on two vertical strings. Two loads of mass and are in equilibrium at equal distance from each other and from the ends of the strings (Fig). Determie the tension T of the left string at the instant when the right string snaps.

Step by step video & image solution for A horizontal weightless rod of length 3l is suspended on two vertical strings. Two loads of mass m_(1) and m_(2) are in equilibrium at equal distance from each other and from the ends of the strings (Fig). Determie the tension T of the left string at the instant when the right string snaps. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
A uniform rod of length 1m and mass 2kg is suspended. Calculate tension T (in N) in the string at the instant when the right string snaps (g=10m/s2).
 View Solution
View SolutionA uniform rod of mass m and length L is suspended through two vertical strings of equal lengths fixed at ends. A small object of mass 2m is placed at distance L/4 from the left end. Find the tensions in the two strings.
View Solution
Knowledge Check
- Question 1 - Select One
A uniform rod of length l and mass M is suspended on two vertical inextensible string as shown in figure. Then tension T in the left string at the instant, when right string snaps is?
 AT=MgBT=Mg2CT=Mg4DT=zero
AT=MgBT=Mg2CT=Mg4DT=zero - Question 2 - Select One
A horizontal rod of length 5m weight 4 kg is suspended at the ends by two strings. The weights 8 kg, 12 kg, 16 kg and 20 kg are placed on the rod at distance 1 m, 2 m, 3 m and 4 m from one end of the rod. Then the tensions in the string are
A26, 34 kg. wt.B20, 30 kg. wtC10, 40 kg. wt.DNone of these - Question 3 - Select One
A stone of mass m tied to a string of length l is rotated in a circle with the other end of the string as the centre. The speed of the stone is v. If the string breaks, the stone will move
Amove towards the centreBmove away from the centreCmove along a tangentDstop

A uniform rod of mass m and length l is held horizontally by two vertical strings of negligible mass, as shown in the figure.
(a). Immediately after the right string is cut, what is the linear acceleration of the end of the rod?
(b). Of the middle of the rod?
(c). Determine the tension in the left string immediately after the right string is cut.View SolutionA uniform rod of length l and mass m is suspended by two vertical inextensible strings as shwon in figure. Then, tension in the left string when right string snape, si
 View Solution
View SolutionTwo masses ,each equal to m ,are arrached to one another by a massless string passing over a smooth pulley the tension in the string is :
View SolutionA uniform rod of mass m and length l is hanged by two stirngs as shown in figure. Suddenly one string is cut. The tension in other string at this instant is
 View Solution
View Solution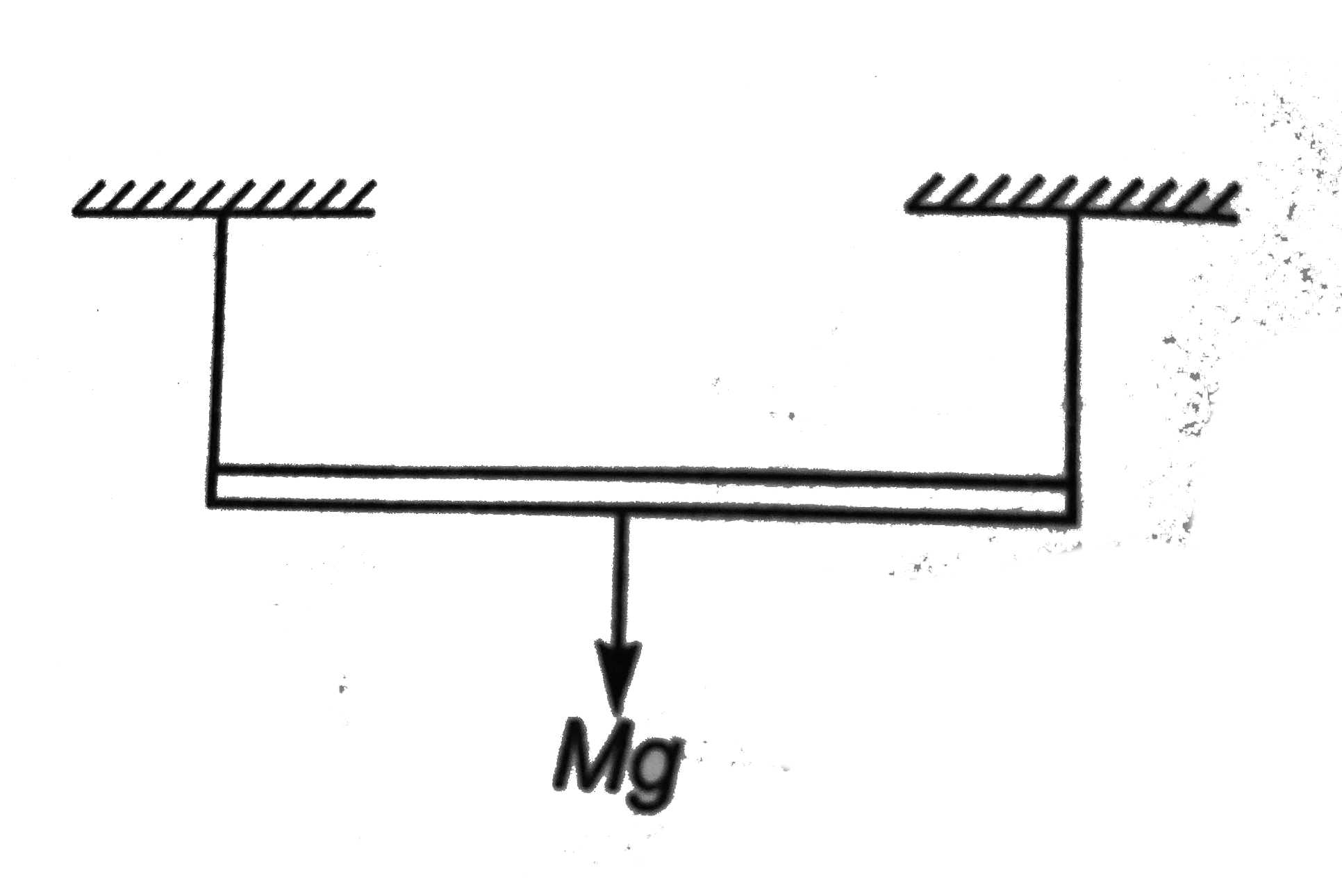
A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.View Solution

