Find the Area of Shaded Region bounded between two semicircles drawn on side of length 28cm of a rectangle as diameter. The other side of the rectangle is 14 cm long. Semi-Circles Rectangle Shaded Area
area of sector=θ360o⋅πr2=16πr2
area of segment(1)= area of sector - area of triangle
(1)=πr26+πr26+√34r2
(1)=πr23−√34r2
(1)+(2)=14πr2
(2)=πr24−πr23+√34r2
(2)=√34r2−πr212
(1)+(2)+(2)+(3)=r2
(3)=r2−14πr2−√34r2+πr212
(3)=r2(1−√34−π6)
shaded region
=2r2(0.044)
=2(14)2(o.044)
=17.248cm2
Similar Questions
Knowledge Check
A rectangle with one side of length 4 cm. is inscribed in a circle of diameter 5 cm. Find, the area of the rectangle.
A21cm2B12cm2C4cm2D3cm2A rectangle of maximum area is drawn inside a circle of diameter 5 cm. What is the maximum area of such a rectangle?
A25cm2B12cm2C12.5cm2DNone of these
one side of a rectangle is 12 cm long and its diagonal measures 37 cm Find the other side and the area of the rectangle .
View SolutionPerimeter of a square and a rectangle is same. If a side of the square is 15cm one side of the rectangle is 18cm, find the area of the rectangle.
View SolutionABCD is a square of side 6 cm. Find the area of the shaded region bounded between square and the two quadrants
View SolutionIn the figure, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
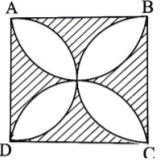
View SolutionIn the figure given below, PQRS is a square of side 14 cm and two semicircles are drawn inside of it with PQ and SR as diameters. Find the area of the shaded region in the figure.

View SolutionThe given figure shows a rectangle. Find the area of the shaded region within the rectangle.
View Solution
