A light rigid sheet of triangular shape has a curved portion cut from it as shown in figure it floats on the surface of water some soap solution is dropped over dotted region surface tension of water and soap film are T1 & T2 respectively T1=1.5T2 Mark correct option

The frame experiences a net force F=(T1−T2)R in y≤0 direction
The frame experiences force F=(T1−T2)R in y≤0 direction
The frame experiences force (T1−T2)(2−π)R in y≥0 direction
Resultant force on wire frame is zero
The correct Answer is:B
Fy=T× projected length in y direction
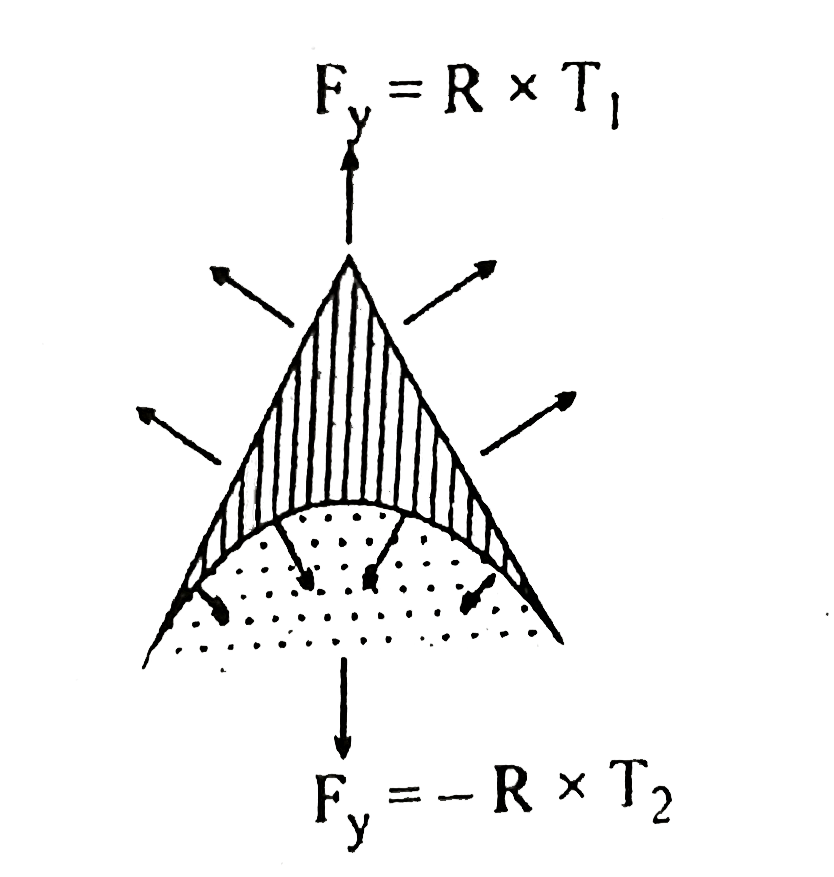
F=(T1−T2)R
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Comphrehension
134 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
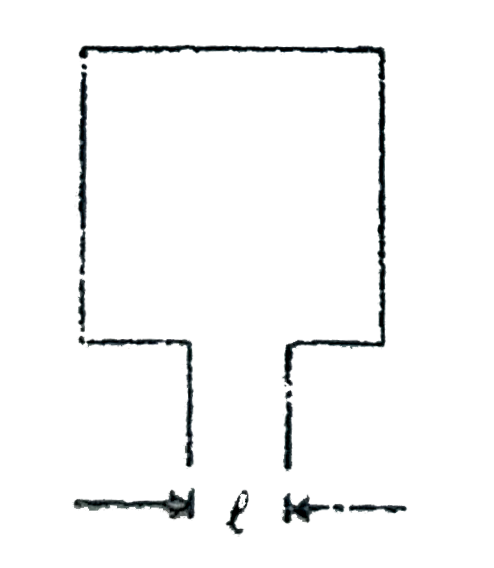
A light open rigid paper frame as shown in (Fig.) floats on the surface of water. What will happen to the frame if some soap solution is dropped inside it ? What force will acts on the frame and in what direction will it act ?
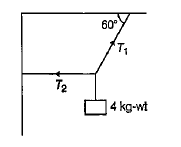
View SolutionDetermine the tensions T1 and T2 in the strings as shown in figure.
View Solution
Knowledge Check
Surface tension of water is T1. When oil spreads on water surface tension becomes T2, then
AT1>T2BT1=T2CT1<T2DT1=T22The addition of soap changes the surface tension of water to T1 and that of salt solution changes to T2. Then
AT1=T2BT1>T2CT1<T2DT1≥T2If T is the surface tension of soap solution, the amount of work done in blowing a soap bubble from a diameter D to 2D is
A2πD2TB4πD2TC6πD2TD8πD2T
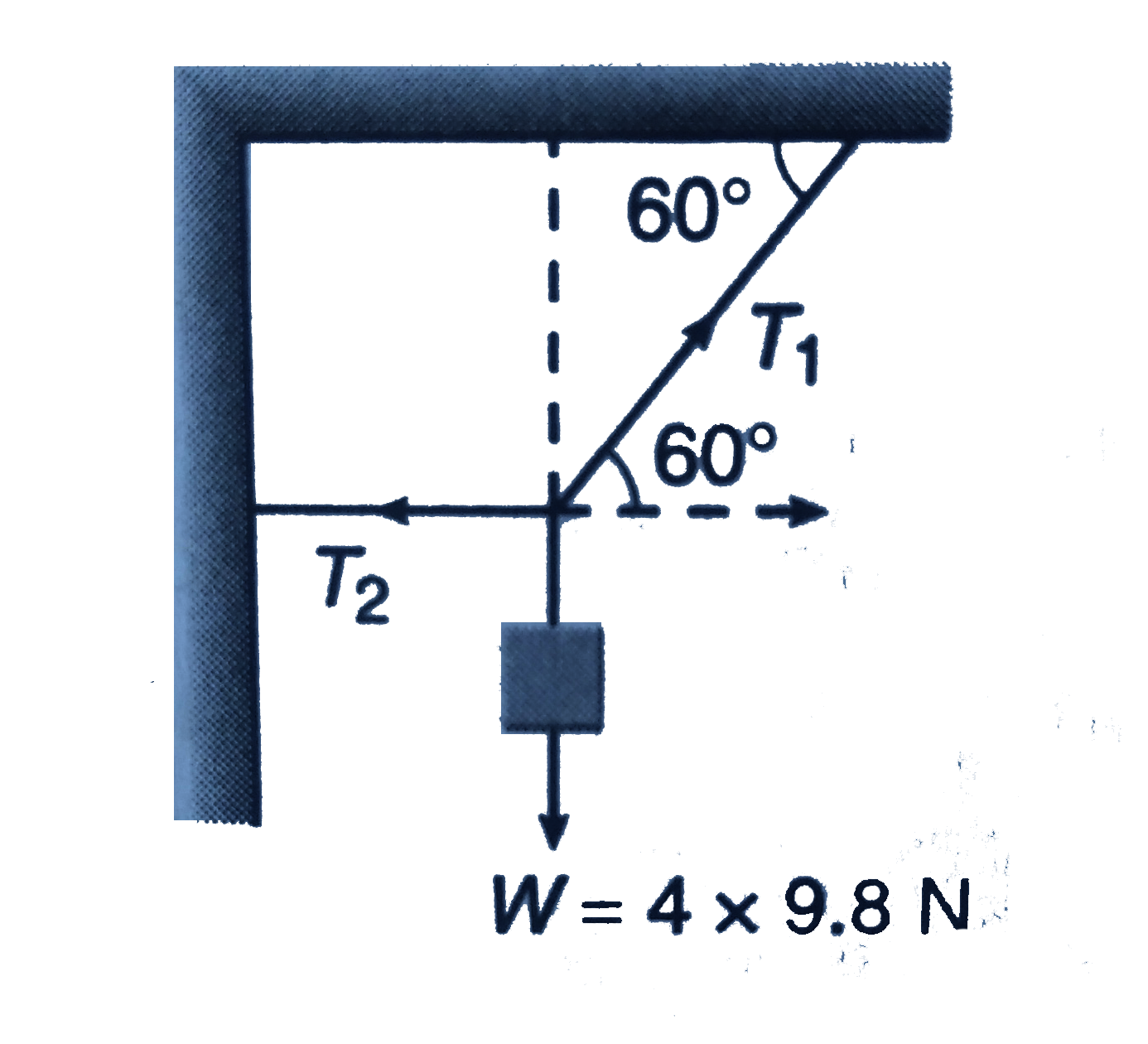
Determine the tensions T1 and T2 in the string as shown in figure.
View SolutionWhat will be the work done in increasing the radius of soap bubble from r2 to 2r, if surface tension of soap solution is T?
View SolutionAn isolated and charged spherical soap bubble has a radius 'r' and the pressure inside is atmospheric. If 'T' is the surface tension of soap solution, then charge on drop is:
View SolutionFor an ideal gas V−T curves at constant pressure P1 & P2 are shown in figure, from the figure
_E01_061_Q01.png)
View SolutionAn isolated and charged spherical soap bubble has a radius r and the pressure inside is atmospheric. IfT is the surface tension of soap solution, then charge on drop isXπ√2rTε0 then find the value of X.
View Solution
