A solid floats with 2/3 of its volume immersed in a liquid and with 3/4 of its volume immersed in another liquid what fraction of its volume will be immersed of it floats in a homogenous mixture formed of equal volumes of the liquids?
6/7
8/11
11/16
12/17
The correct Answer is:D
To solve the problem, we need to find the fraction of the volume of a solid that will be immersed when it floats in a homogeneous mixture formed of equal volumes of two different liquids. Let's break down the solution step by step.
Step 1: Understanding the Given Information
We know that:
- In the first liquid, the solid is floating with 23 of its volume immersed.
- In the second liquid, the solid is floating with 34 of its volume immersed.
Let V be the total volume of the solid, ρs be the density of the solid, ρ1 be the density of the first liquid, and ρ2 be the density of the second liquid.
Step 2: Applying the Principle of Buoyancy
According to Archimedes' principle, the weight of the solid is equal to the weight of the liquid displaced by the immersed volume of the solid.
1. For the first liquid:
Weight of solid=V⋅ρs⋅g
Weight of liquid displaced=(23V)⋅ρ1⋅g
Setting these equal gives:
V⋅ρs⋅g=(23V)⋅ρ1⋅g
Cancelling V and g from both sides:
ρs=23ρ1
2. For the second liquid:
Weight of liquid displaced=(34V)⋅ρ2⋅g
Setting the weights equal gives:
V⋅ρs⋅g=(34V)⋅ρ2⋅g
Cancelling V and g:
ρs=34ρ2
Step 3: Relating the Densities
From the equations we derived:
1. ρ1=32ρs
2. ρ2=43ρs
Step 4: Finding the Density of the Mixture
When equal volumes of the two liquids are mixed, the density of the mixture ρm can be calculated as:
ρm=ρ1+ρ22
Substituting the values of ρ1 and ρ2:
ρm=32ρs+43ρs2
Finding a common denominator (which is 6):
ρm=96ρs+86ρs2=176ρs2=1712ρs
Step 5: Finding the Immersed Volume in the Mixture
Let V′ be the volume of the solid immersed in the mixture. Using the principle of buoyancy again:
V′⋅ρm⋅g=V⋅ρs⋅g
Cancelling g and rearranging gives:
V′⋅1712ρs=V⋅ρs
Cancelling ρs from both sides:
V′⋅1712=V
Thus,
V′=V⋅1217
Step 6: Finding the Fraction of Volume Immersed
The fraction of the volume of the solid that is immersed in the mixture is:
Fraction immersed=V′V=1217
Final Answer
The fraction of the volume of the solid that will be immersed when it floats in the homogeneous mixture is 1217.
---
We know that:
- In the first liquid, the solid is floating with 23 of its volume immersed.
- In the second liquid, the solid is floating with 34 of its volume immersed.
According to Archimedes' principle, the weight of the solid is equal to the weight of the liquid displaced by the immersed volume of the solid.
Weight of solid=V⋅ρs⋅g
Weight of liquid displaced=(23V)⋅ρ1⋅g
Setting these equal gives:
V⋅ρs⋅g=(23V)⋅ρ1⋅g
Cancelling V and g from both sides:
ρs=23ρ1
Weight of liquid displaced=(34V)⋅ρ2⋅g
Setting the weights equal gives:
V⋅ρs⋅g=(34V)⋅ρ2⋅g
Cancelling V and g:
ρs=34ρ2
From the equations we derived:
1. ρ1=32ρs
2. ρ2=43ρs
When equal volumes of the two liquids are mixed, the density of the mixture ρm can be calculated as:
ρm=ρ1+ρ22
Substituting the values of ρ1 and ρ2:
ρm=32ρs+43ρs2
Finding a common denominator (which is 6):
ρm=96ρs+86ρs2=176ρs2=1712ρs
Let V′ be the volume of the solid immersed in the mixture. Using the principle of buoyancy again:
V′⋅ρm⋅g=V⋅ρs⋅g
Cancelling g and rearranging gives:
V′⋅1712ρs=V⋅ρs
Cancelling ρs from both sides:
V′⋅1712=V
Thus,
V′=V⋅1217
The fraction of the volume of the solid that is immersed in the mixture is:
Fraction immersed=V′V=1217
The fraction of the volume of the solid that will be immersed when it floats in the homogeneous mixture is 1217.
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Comphrehension134videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning75videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE10videos
FLUID MECHANICS
- BOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question32videos
SEMICONDUCTORS
Similar Questions
A solid body floating in water has 15th of its volume immersed in it. What fraction of its volume will be immersed, if it floats in a liquid of specific gravity 1.2 ?
View SolutionA uniform cylindrical body when placed in liquid a floats with one third of its length outside the liquid.
When placed in liquid B, it floats with one third of its length immersed in the liquid. When the body is made to float vertically in a homogeneous mixture of equal volumes of the two liquids, 25 cm of its length is seen in air. Find the length of the body.View Solution
Knowledge Check
- Question 1 - Select One
A body floats with one-third of its volume outside water and 3/4 of its volume outside another liquid. The density of another liquid is
A94g/ccB49g/ccC83g/ccD38g/cc - Question 2 - Select One
A body floats with one-third of its volume outside water and 3/4 of its volume outside another liquid. The density of another liquid is :
A94g/ccB49g/ccC83g/ccD38g/cc - Question 3 - Select One
A block of density ρ floats in a liquid with its one third volume immersed. The density of the liquid is
AρBρ3Cρ2D3ρ
A solid body is floating in water with 5/6th of its volume inside the water. What fraction of its volume will be outside if it ftoats in a liquid of specific gravity 1.6?
View SolutionA plank is floating in a liquid as shown, Fraction f of its volume is immersed. Choose the correct options
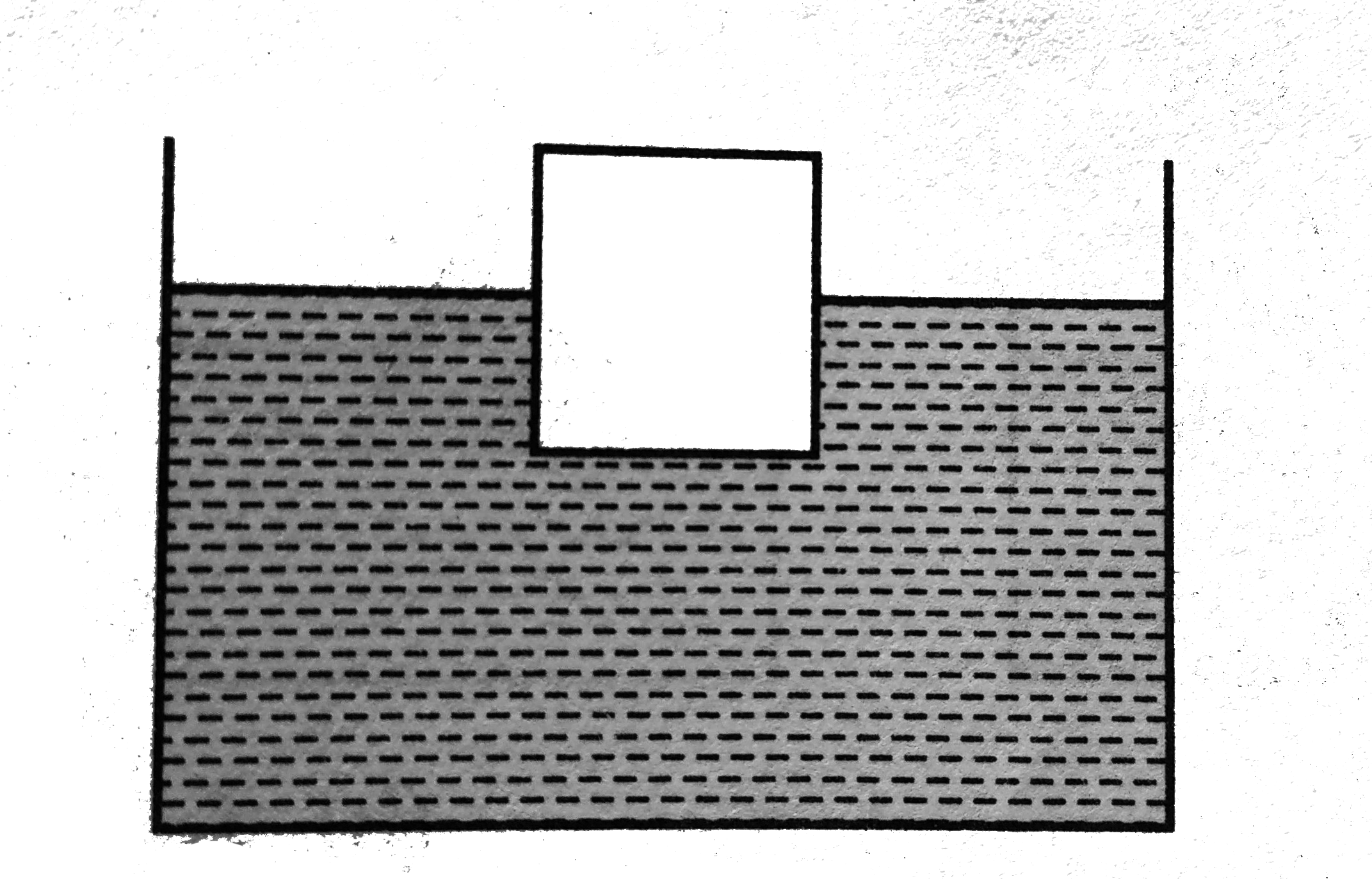 View Solution
View SolutionA body of density d and volume V floats with volumes V of its total volume V immersed in a liquid of density d and the rest of the volume V2 immersed in another liquid of density d2(<d1). The volume V1 immersed in liquid of density d1 is
View SolutionA non-conducting body floats in a liquid at 20∘C. with 2/3 of its volume immersed in the liquid When liquid temperature is increased to 100∘C,3/4 of body volume is immersed in the liquid. Then the coefficient of real expansion of the liquid is... (neglecting the expansion of container of the liquid)
View SolutionTwo solids A and B floats in water. It is observed that A floats with half of its volume immersed and B Floats with 2/3 of its volume immersed. The ration of densities of A and B is
View Solution

