In the front of the upper slit of YDSE apparatus, a thin film of a liquid of refractive index 1.40 is placed. It is a hot day and the liquid starts evaporating from the surface. A beam of light at wavelength 560 nm is incident onto the YDSE apparatus and the intensity I at the centre of the screen is monitored. Figure gives intensity I as a function of time t. The intensity changes because of evaporation from the two sides of the film. Assume that the film is flat and has parallel sides. Also assume that the film's thickness decreases at a constant rate
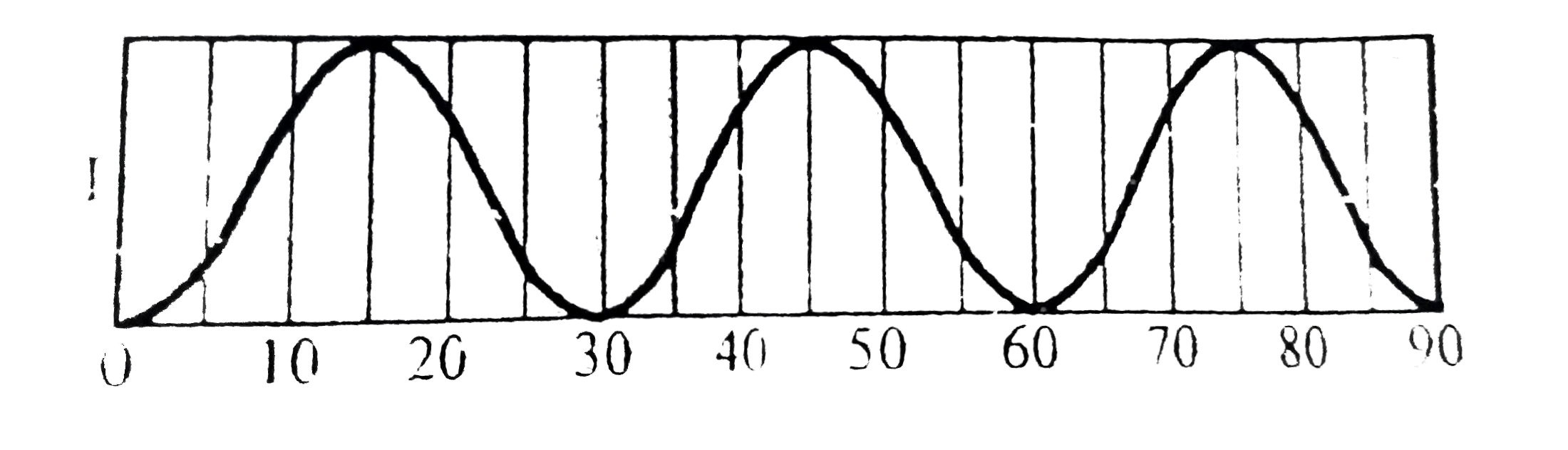
If the maximum intensity is I0 then
Intensity at t=0secisI0√2
Intensity at t=10secis3I04
Intensity at t=5secisI04
Intensity at t=5sec,I02√2
The correct Answer is:B, C
I=I0cos2ϕ2
⇒int=10sec,Δϕ=π15×10=2π3
⇒ϕ=p+2π3
I=I0(cos(π2+π3))2=I0sin2π3=34I0
int=5sec,Δϕ=π15×5=π3
ϕ=π+π3
I=10(cos(π2+π6))2=I0sin2π6=I04
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Multiple objective
131 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic
70 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
Statement-1: The numerical value (n)of a physical quantity is inversely proportional to size of unit (u) being used to measure it.
Statement-2:The magnitude of physical quantity does not change by changing the units.
View SolutionThe quantity// quantities that does //do not have mass in its// their dimentions (when we take standard 7 quantities as fundmental) is//are:
View SolutionThe manifestation of band structure in solids is due to
View SolutionA cylinder of height 20m is completely filled with water. The velocity of effux of water (∈ms−1) through a small hole on the side wall of the cylinder near its bottom is
View SolutionHow does the width of the depletion layer of a p-n junction diode change with decrease in reverse bias?
View SolutionIn YDSE, both slits produce equal intensities on the screen. A 100% transparent thin film is placed in front of one of the slits. Now, the intensity on the centre becomes 75% of the previous intensity. The wavelength of light is 6000Å and refractive index of glass is 1.5. Thus, minimum thickness of the glass slab is
View SolutionA YDSE is performed in a medium of refractive index 4 // 3 , A light of 600 nm wavelength is falling on the slits having 0.45 nm separation . The lower slit S_(2) is covered b a thin glass plate of thickness 10.4 mm and refractive index 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in figure. (All the wavelengths in this problem are for the given medium of refractive index 4 // 3 , ignore absorption.) Find the light intensity at point O relative t maximum fringe intensity.
View Solution_E01_102_Q01.png)
In a YDSE a parallel beam of light of wavelength 6000Å is incident on slits at angle of incidence 30∘. A and B are two thin transparent films each of refractive index 1.5. thickness of A is 20.4μm. Light coming through A and B have intensities I and 4I resepctively on the screen. Intensity at point O which is symmetric relative to the slits is 3I. The central maxima is above O.
(i). what is the maximum thickness of B to do so. Assuming thickness of B to be that found in part (i) answer the following parts.
(ii). Find fringe width, maximum intensity and minimum intensity on screen.
(iii). Dinstance of nearest minima from O
(iv) intensity at 5 cm on either side of O.
View SolutionIn the front of the upper slit of YDSE apparatus, a thin film of a liquid of refractive index 1.40 is placed. It is a hot day and the liquid starts evaporating from the surface. A beam of light at wavelength 560 nm is incident onto the YDSE apparatus and the intensity I at the centre of the screen is monitored. Figure gives intensity I as a function of time t. The intensity changes because of evaporation from the two sides of the film. Assume that the film is flat and has parallel sides. Also assume that the film's thickness decreases at a constant rate
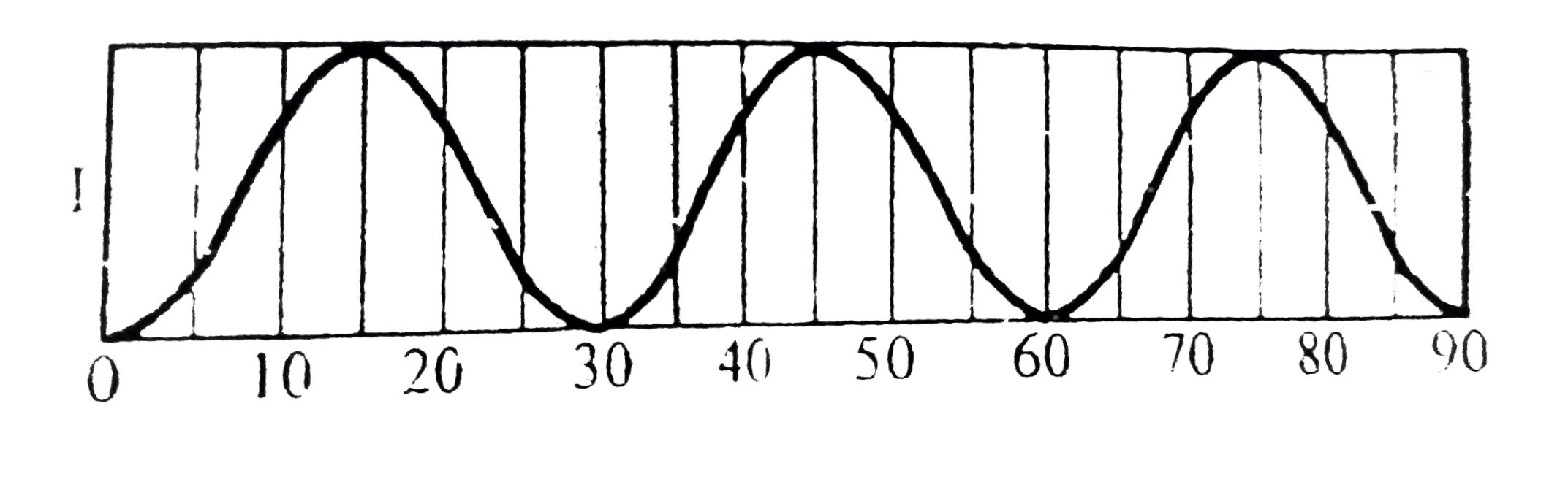
What can be the initial thickness of the film?
View SolutionIn the front of the upper slit of YDSE apparatus, a thin film of a liquid of refractive index 1.40 is placed. It is a hot day and the liquid starts evaporating from the surface. A beam of light at wavelength 560 nm is incident onto the YDSE apparatus and the intensity I at the centre of the screen is monitored. Figure gives intensity I as a function of time t. The intensity changes because of evaporation from the two sides of the film. Assume that the film is flat and has parallel sides. Also assume that the film's thickness decreases at a constant rate The rate of change of thickness can be
View SolutionIn YDSE experiment a uniform intensity light beam is incident on slit plane which has two slits having width ratio 9:4. Find the ratio of intensities of bright and dark fringes on screen.
[25]
View SolutionThe distance between two slits in a YDSE apparatus is 3mm. The distance of the screen from the slits is 1m. Microwaves of wavelength 1 mm are incident on the plane of the slits normally. Find the distance of the first maxima on the screen from the central maxima.
View SolutionThe distance between two slits in a YDSE apparatus is 3mm. The distance of the screen from the slits is 1m. Microwaves of wavelength 1 mm are incident on the plane of the slits normally. Find the distance of the first maxima on the screen from the central maxima.
View SolutionIn YDSE the distance between the slits is 1mm and screen is 25nm away from intensities IF the wavelength of light is 6000A the fringe width on the screen is
View SolutionA:When the apparatus of YDSE is brought in a liquid from air, the fringe width decreases.
R: The wavelength of light decreases in the liquid.
View Solution
