Find the total number of fringes formed on screen in the L loyd's mirror arrangement shown.

The correct Answer is:0012
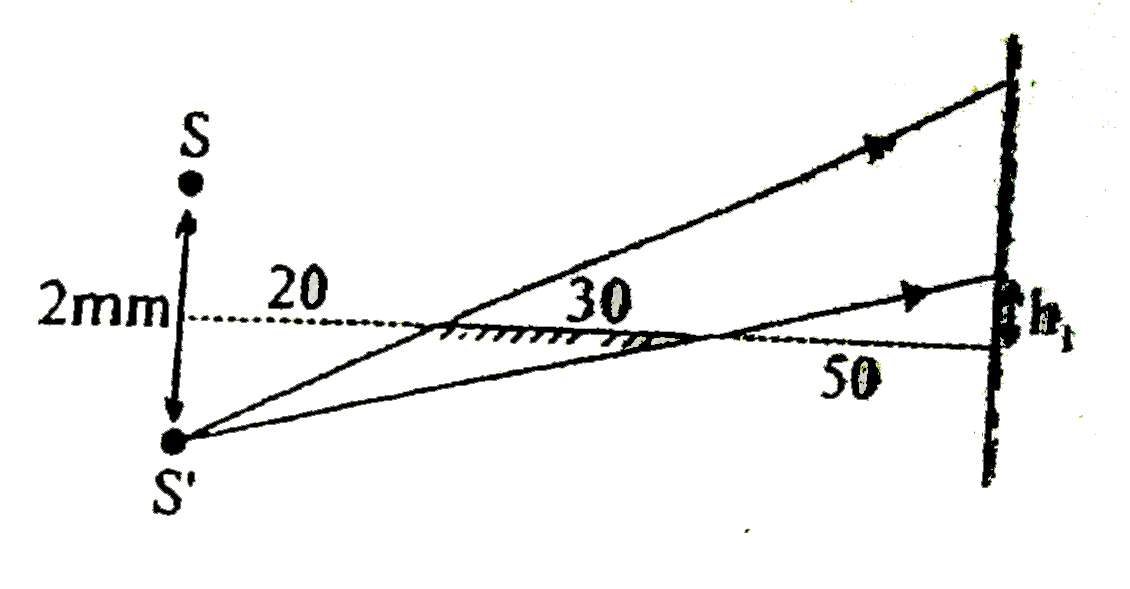
0.1h1=5050⇒h1=1mm
L+h10.1=8020⇒L+h1=4mm⇒L=3mm
B=λDd=5×10−7×12×10−3=2.5×10−4 m
N=LB=3×10−32.5×10−4=30025=12
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic
70 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Multiple objective
131 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
Knowledge Check
Find the number of all whole numbers formed on the screen of a calculator which can be recognised as numbers with (unique) correct digits when they are read inverted. The greatest number formed onn its screen is 999999.
A98970B89912C110050D100843Find the number of all whole numbers formed on the screen of a calculator which can be recognised as numbers with (unique) correct digits when they are read inverted. The greatest number formed onn its screen is 999999.
A98970B89912C110050D100843
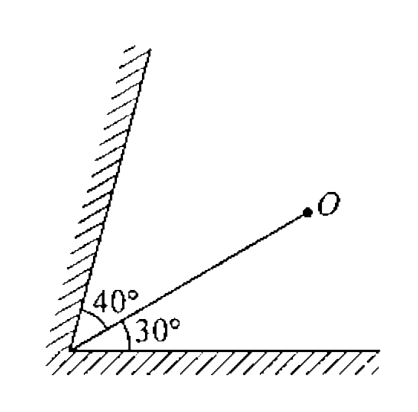
Two plane mirrors are inclined at an angle of 75∘ to each other. Find the total number of images formed when an object is placed as shown in figure

View SolutionTwo plane mirrors are inclined at an angle of 70∘ to each other. Find the total number of images formed when object is placed as shown. In figure-. Total images = 5
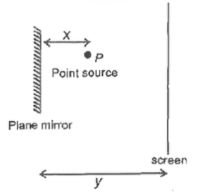
View SolutionShape of interference fringes formed on the screen due to point source P, in the case shown here
View SolutionFind the number of all whole numbers formed on the screen of a calculator which can be recognised as numbers with (unique) correct digits when they are read inverted. The greatest number formed onn its screen is 999999.
View SolutionFind the number of all whole numbers formed on the screen of a calculator which can be recognised as numbers with (unique) correct digits when they are read inverted. The greatest number formed onn its screen is 999999.
View SolutionFind the number of whole numbers formed on the screen of a calculator which can read upside done (i.e can be recognized as numbers with unique( correct) digits). It is given that greatest numbers that can be formed on the screen of the calculator is 99999999.
View Solution

