Pressure over ideal binary liquid mixture containing 10 moles each of liquid A and B is gradually decreased isothermally. If P∘A=200mm Hg and P∘B=100mmHg. Find the pressure at which half of the liquid is converted into vapour.
150 mm Hg
166.5 mmHg
133 mmHg
141.4 mmHg
The correct Answer is:D
To solve the problem of finding the pressure at which half of the liquid is converted into vapor in an ideal binary liquid mixture of liquids A and B, we can follow these steps:
Step 1: Understand the Given Data
- Moles of liquid A (nA) = 10
- Moles of liquid B (nB) = 10
- Total moles (n_total) = nA + nB = 20
- Vapor pressure of pure A (P_A^0) = 200 mm Hg
- Vapor pressure of pure B (P_B^0) = 100 mm Hg
Step 2: Define the Situation
When half of the liquid is converted into vapor, we have:
- Moles of liquid A remaining = nA - yA
- Moles of liquid B remaining = nB - yB
- Moles of vapor A = yA
- Moles of vapor B = yB
Given that half of the liquid is converted into vapor, we have:
- yA + yB = 10 (since half of the total moles of liquid, which is 20, is 10)
Step 3: Write the Relationships
Using Raoult's Law, we can express the partial pressures of A and B:
- Partial pressure of A (P_A) = x_A * P_A^0
- Partial pressure of B (P_B) = x_B * P_B^0
Where:
- x_A = mole fraction of A in the liquid phase
- x_B = mole fraction of B in the liquid phase
Step 4: Calculate Mole Fractions
The mole fractions can be expressed as:
- x_A = (nA - yA) / (n_total - (yA + yB)) = (10 - yA) / (20 - 10) = (10 - yA) / 10
- x_B = (nB - yB) / (n_total - (yA + yB)) = (10 - yB) / (20 - 10) = (10 - yB) / 10
Step 5: Substitute into Raoult's Law
Using the mole fractions in Raoult's Law:
- P_A = (10 - yA) / 10 * 200
- P_B = (10 - yB) / 10 * 100
Step 6: Total Pressure
The total pressure (P_total) is given by:
Ptotal=PA+PB
Substituting the expressions from Raoult's Law:
Ptotal=(10−yA10⋅200)+(10−yB10⋅100)
Step 7: Substitute yB
Since yB = 10 - yA, we can substitute this into the equation:
Ptotal=(10−yA10⋅200)+(10−(10−yA)10⋅100)
Ptotal=(10−yA10⋅200)+(yA10⋅100)
Step 8: Simplify the Equation
Now, simplify the equation:
Ptotal=2000−200yA+100yA10
Ptotal=2000−100yA10
Ptotal=200−10yA
Step 9: Solve for yA
To find the pressure at which half of the liquid is converted into vapor, we need to find yA when yA = 5 (since half of 10 moles is 5 moles):
Ptotal=200−10(5)
Ptotal=200−50
Ptotal=150 mm Hg
Conclusion
The pressure at which half of the liquid is converted into vapor is 150 mm Hg.
- Moles of liquid A (nA) = 10
- Moles of liquid B (nB) = 10
- Total moles (n_total) = nA + nB = 20
- Vapor pressure of pure A (P_A^0) = 200 mm Hg
- Vapor pressure of pure B (P_B^0) = 100 mm Hg
When half of the liquid is converted into vapor, we have:
- Moles of liquid A remaining = nA - yA
- Moles of liquid B remaining = nB - yB
- Moles of vapor A = yA
- Moles of vapor B = yB
- yA + yB = 10 (since half of the total moles of liquid, which is 20, is 10)
Using Raoult's Law, we can express the partial pressures of A and B:
- Partial pressure of A (P_A) = x_A * P_A^0
- Partial pressure of B (P_B) = x_B * P_B^0
- x_A = mole fraction of A in the liquid phase
- x_B = mole fraction of B in the liquid phase
The mole fractions can be expressed as:
- x_A = (nA - yA) / (n_total - (yA + yB)) = (10 - yA) / (20 - 10) = (10 - yA) / 10
- x_B = (nB - yB) / (n_total - (yA + yB)) = (10 - yB) / (20 - 10) = (10 - yB) / 10
Using the mole fractions in Raoult's Law:
- P_A = (10 - yA) / 10 * 200
- P_B = (10 - yB) / 10 * 100
The total pressure (P_total) is given by:
Ptotal=PA+PB
Ptotal=(10−yA10⋅200)+(10−yB10⋅100)
Since yB = 10 - yA, we can substitute this into the equation:
Ptotal=(10−yA10⋅200)+(10−(10−yA)10⋅100)
Ptotal=(10−yA10⋅200)+(yA10⋅100)
Now, simplify the equation:
Ptotal=2000−200yA+100yA10
Ptotal=2000−100yA10
Ptotal=200−10yA
To find the pressure at which half of the liquid is converted into vapor, we need to find yA when yA = 5 (since half of 10 moles is 5 moles):
Ptotal=200−10(5)
Ptotal=200−50
Ptotal=150 mm Hg
The pressure at which half of the liquid is converted into vapor is 150 mm Hg.
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - SOLUTIONSEXERCISE - Exercise 124videos
SOLUTIONS
- BOOK - BANSALCHAPTER - SOLUTIONSEXERCISE - Exercise 214videos
SOLUTIONS
- BOOK - BANSALCHAPTER - SOLUTIONSEXERCISE - Solved Examples28videos
SOLUTIONS
- BOOK - BANSALCHAPTER - RADIO ACTIVITYEXERCISE - Exercise-412videos
RADIO ACTIVITY
- BOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS1videos
TEST PAPERS
Similar Questions
1 mole of liquid A and 9 moles of liquid B are mixed to form a solution. If P∘B=400mm of Hg and P∘A=20mm of Hg at a temperature 'T' and normal boiling point of liquid B is 300K then answer the questions that follow.
Given data: Kb=2.7K kg mol−1, Molar mass of B=100
If 'A' is perfectly non volatile and it dimerises to and extent of 60% then what will be the vapour pressure of the solution.View SolutionA mixture contains 1 mole of volatile liquid A (P0A=80mm Hg). and 3 moles of volatile liquid B (P0A=80mm Hg). If solution behaves ideally, the total vapour pressure of the distillate is
View Solution
Knowledge Check
- Question 1 - Select One
A mixture contains 1 mole of volatile liquid A (P0A=80mm Hg). and 3 moles of volatile liquid B (P0A=80mm Hg). If solution behaves ideally, the total vapour pressure of the distillate is
A85 mm HgB85.88 mm HgC90 mmHgD92 mm Hg - Question 2 - Select One
Molal fraction of A vapours above te solution in mixture of A and B [MA5]A3 will be
[Given :P∘A=100mm Hg and P∘B=200mm Hg]A0.4B0.8C0.25DNone of these - Question 3 - Select One
1 mole of liquid A and 9 moles of liquid B are mixed to form a solution. If P∘B=400mm of Hg and P∘A=20mm of Hg at a temperature 'T' and normal boiling point of liquid B is 300K then answer the questions that follow.
Given data: Kb=2.7K kg mol−1, Molar mass of B=100
It is observed that pressure of vapour above the solution at 'T' Kelvin is 350 mm Hg. The true statement is :AThe liquids form ideal solution.BThe solute- solvent interactions are weaker than solvent- solvent or solute- solute interactions.CThe volume of final solution will be lower than sum of individual volumesDThe enthalpy change due to mixing will be zero.
If same amount of gas is trapped over liquid (a) and liquid (b) in following containers. Assuming temperature and cross-sectional area of container are same. Patm=760mmHg,dHg=13.6g/mL
Find the pressure (in cm Hg) of gas over the liquid (b).
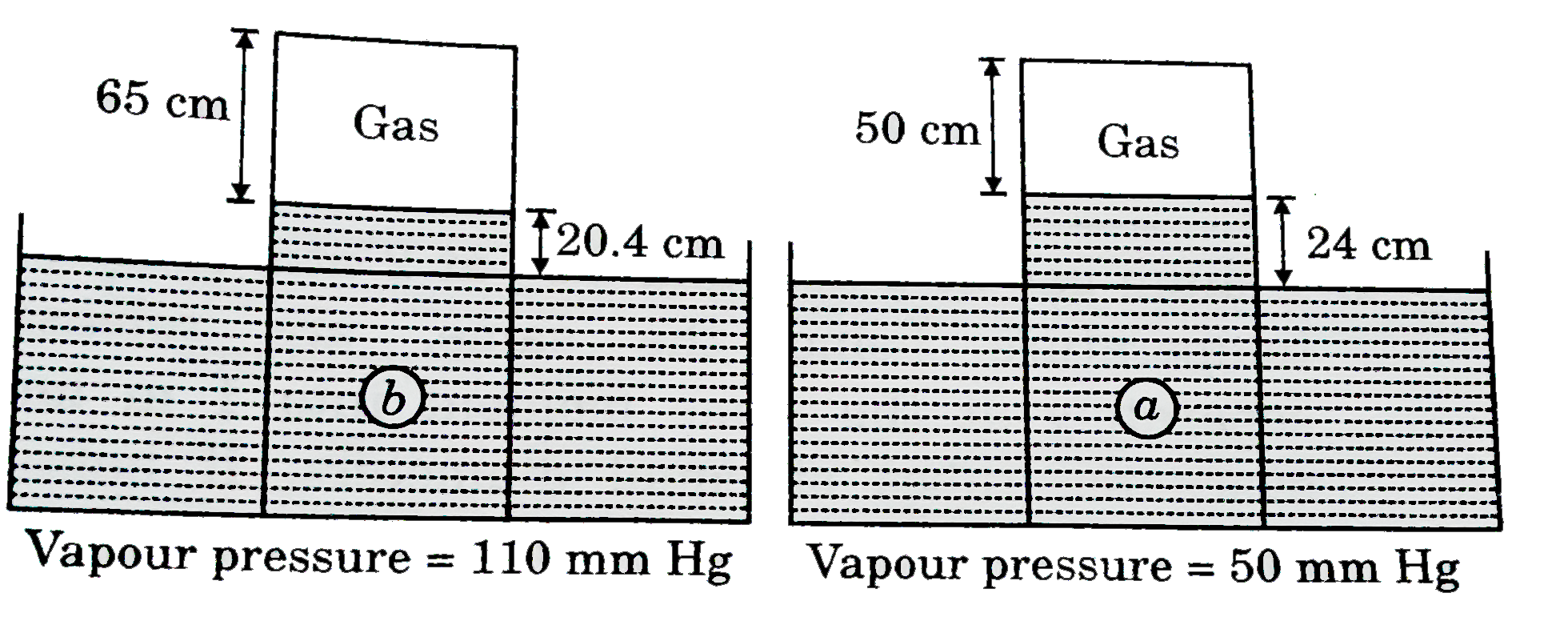 View Solution
View SolutionAt 300 K the vapour pressure of an ideal solution containing 1 mole of liquid A and 2 moles of liquid B is 500 mm of Hg. The vapour pressure of the solution increases by 25 mm of Hg, if one more mole of B is added to the above ideal solution at 300K. Then the vapour pressure of A in its pure state is
View SolutionTotal vapour pressure of mixture of 1 mole of volatile component A (P∘A=100mmHg) and 3 mole of volatile component B(P∘B=80mmHg) is 90mmHg. For such case :
View SolutionIf same amount of gas is trapped over liqquid (a) and liquid (b) in following container4s. Assuming temperature and cross-sectional area of container are same.
Patm=760mmHg,dHg=13.6g/mL
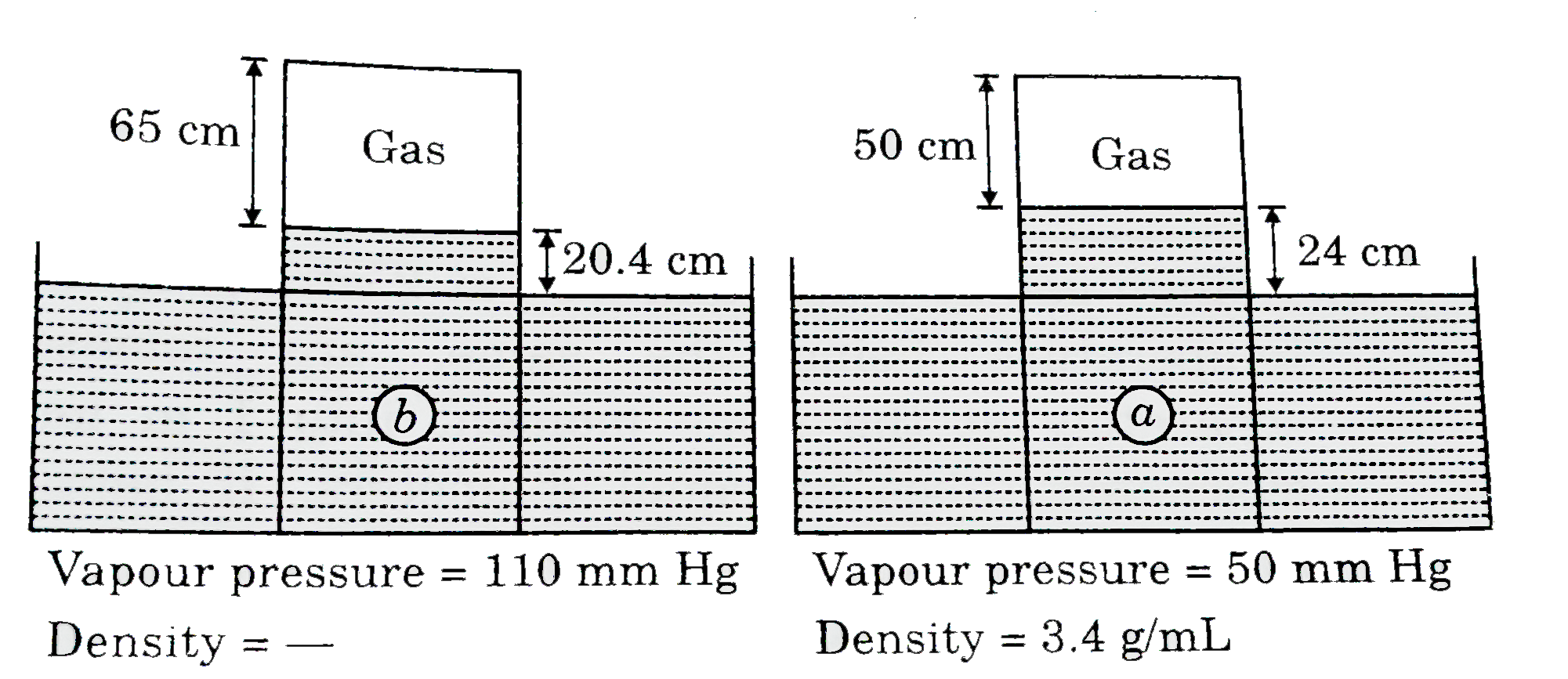
If same amount of gas is trapped over liquid (a) and liquid (b) in following containers. Assuming temperature and cross-sectional area of container are same. Patm=760mmHg,dHg=13.6g/mL
Find the pressure (in cm Hg) of gas over liquid (a)View SolutionThe vapour pressure of a solution of the liquids A (p∘=80mmHgandXA=0.4)and(p∘=120mnHgandXB=0.6) is found to be 100 mm Hg. It shows that the solution exhibits
View Solution

