Question

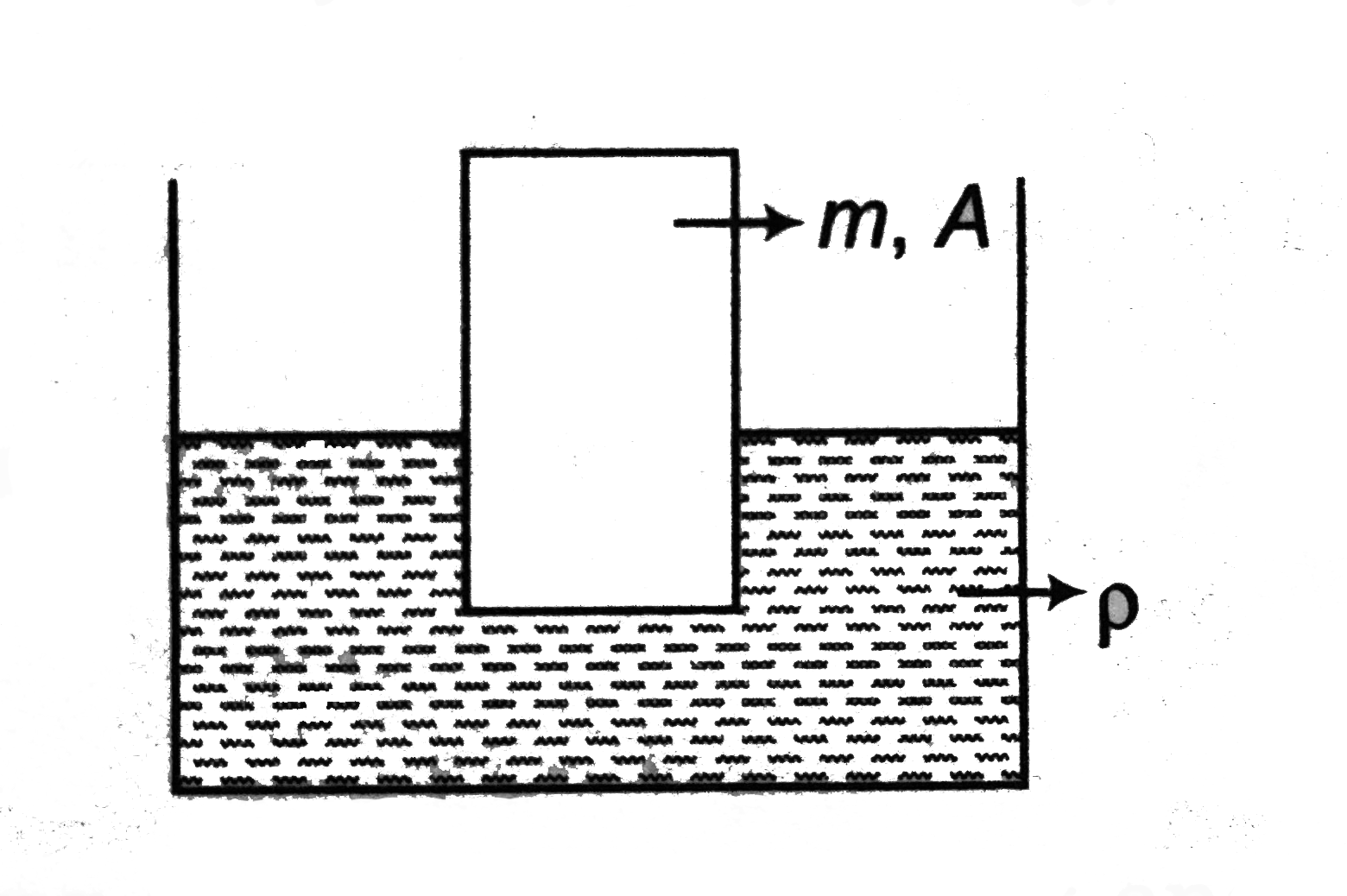
A rectangular block of mass m and area of cross-section A floats in a liquid of density $\rho$. If it is given a small vertical displacement from equilibrium, it undergoes oscillation with a time period T. Then

Options

A. `T prop sqrt(rho)`
B. `T prop (1)/(sqrt(A))`
C. `T prop (1)/(rho)`
D. `T prop (1)/(sqrt(m))`

Answer

B

Explanation

To solve the problem, we will derive the expression for the time period $$T$$ of oscillation of a rectangular block floating in a liquid when displaced vertically from its equilibrium position.

1. Understanding the Forces:
When the block is floating, it displaces a volume of liquid equal to the weight of the block. The buoyant force acting on the block is given by:
\( F_b = \rho g V \)
where \( V \) is the volume of the liquid displaced. For a block of area \( A \) and depth \( x \) submerged, the volume is \( V = A x \). Thus, the buoyant force becomes:
\( F_b = \rho g A x \)

2. Weight of the Block:
The weight of the block is:
\( F_g = mg \)

3. Equilibrium Condition:
At equilibrium, the buoyant force equals the weight of the block:
\( \rho g A h = mg \)
where \( h \) is the depth of the block submerged at equilibrium.

4. Displacement from Equilibrium:
When the block is displaced by a small distance \( x \) downwards, the new submerged depth is \( h + x \). The new buoyant force becomes:
\( F_b' = \rho g A (h + x) = \rho g A h + \rho g A x \)

5. Net Force:
The net force acting on the block when it is displaced is given by:
\( F_{net} = F_b' - F_g = \rho g A (h + x) - mg \)
Since \( \rho g A h = mg \), we can simplify this to:
\( F_{net} = \rho g A x \)

6. Using Newton's Second Law:
According to Newton's second law, the net force is also equal to mass times acceleration:
\( F_{net} = -m \frac{d^2x}{dt^2} \)
Setting these two expressions for net force equal gives:
\( -m \frac{d^2x}{dt^2} = -\rho g A x \)
Rearranging gives:
\( \frac{d^2x}{dt^2} + \frac{\rho g A}{m} x = 0 \)

7. Identifying the Angular Frequency:
This is a standard form of simple harmonic motion, where:
\( \omega^2 = \frac{\rho g A}{m} \)
Thus, the angular frequency \( \omega \) is:
\( \omega = \sqrt{\frac{\rho g A}{m}} \)

8. Finding the Time Period:
The time period \( T \) of oscillation is related to the angular frequency by:
\( T = \frac{2\pi}{\omega} = 2\pi \sqrt{\frac{m}{\rho g A}} \)

Final Expression:
Thus, the time period of oscillation \( T \) is given by:
\( T = 2\pi \sqrt{\frac{m}{\rho g A}} \)