Two parallel plates which are sparated by a very small distance d, are dipped in water. To how much height will the water raise between the plates (Assume contact angle θ→0)
Lets draw free body diagram of the water raised up. Forces on it are :
(i) The plates pull the surface in upward direction with a force 2Tl
(ii) The weight of raiseed water =(ρ)(lhd)g For equilibrium, force shoul be balanced.
2Tl=(ρ)(lhd)g⇒h=2Tρgd
Also Td/2=ρgh, so we can say that pressure excess due to cylindrical surface =Td/2=Trc
pressure excess due to spherical surface =2Tfc
pressure excess due to cylindrical surface =Trc
Alternative method :
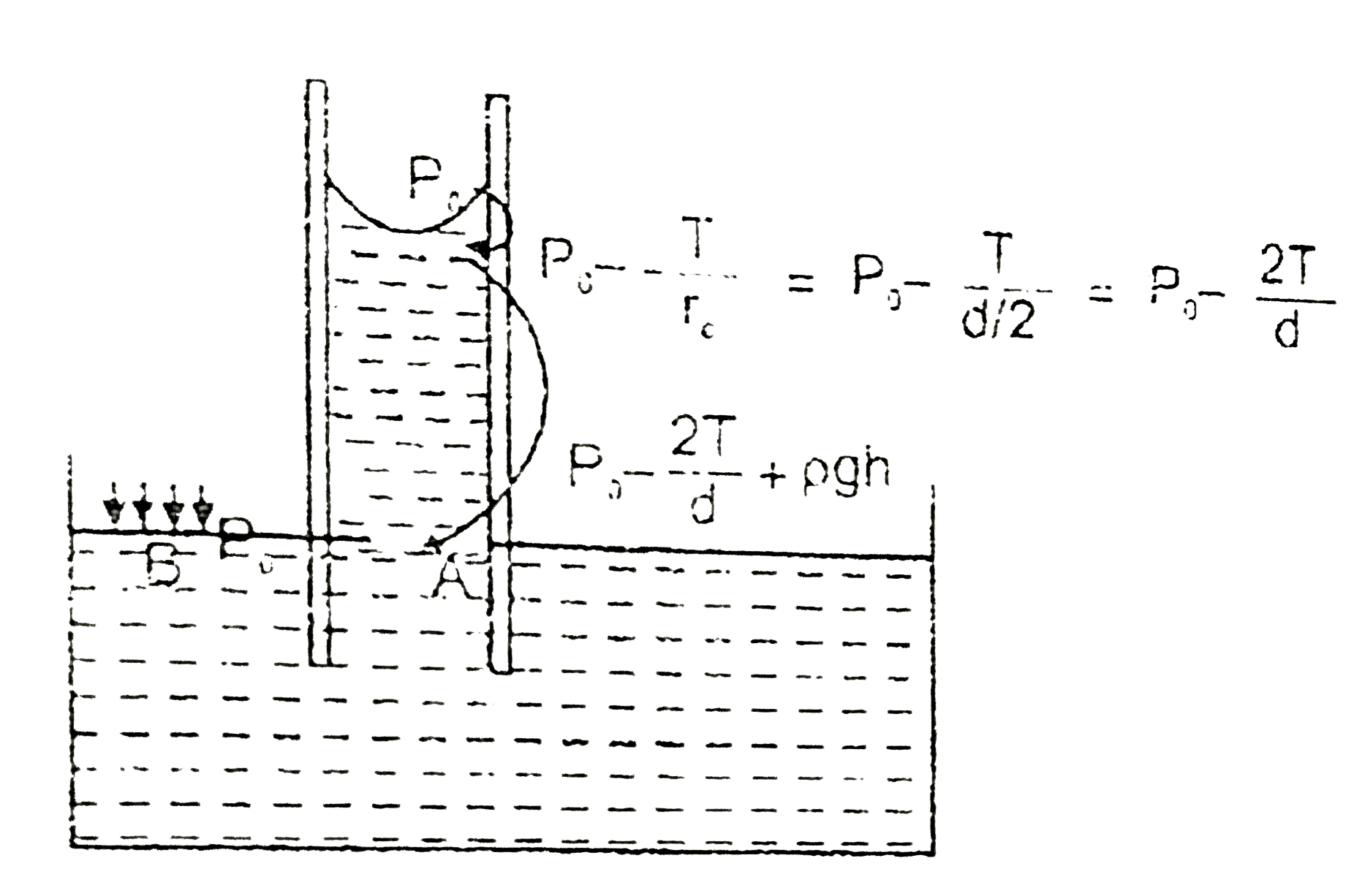
⇒P0−2Td+ρgh=P0⇒h=2Tρgd
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - SURFACE TENSIONEXERCISE - Exercise- 1 PART - I10videos
SURFACE TENSION
- BOOK - RESONANCECHAPTER - SURFACE TENSIONEXERCISE - Exercise- 1 PART - II17videos
SURFACE TENSION
- BOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise32videos
STRING WAVES
- BOOK - RESONANCECHAPTER - UNITS, DIMENSION & MEASUREMENTEXERCISE - Exercise27videos
UNITS, DIMENSION & MEASUREMENT
Similar Questions
A thin capillary of inner radius r1 and outer radius r2 (The inner tube is solid) is dipped in water. To how much height will the water raise in the tube ? (Assume contact angle θ→0)
View SolutionFind the attractive force is newton between two parallel glass plates, separated by a distance h=0.1mm after a water drop of mass m=70mg was introduced between them. Assume wetting to be complete and surface tensin of water, T=70 dyne/cm
View Solution
Knowledge Check
- Question 1 - Select One
Two vertical glass plates 1 mm apart are dipped into water. How high will the water rise between the plates. If the surface tension of water is 70 dyne cm−1
A1.43 cmB1.63 cmC2.86 cmD3.86 cm - Question 2 - Select One
Two vertical parallel glass plates , separated by 0.5 mm, are kept in water . The surface tension of water is 7×10−2 N/m . How high will the water rise between the plates ? (use g = 10m/s2)
A1.4 cmB2 cmC2.8 cmD3.5 cm - Question 3 - Select One
_E01_107_Q01.png)
Two very wide parallel glass plates are held vertically at a small separation d, and dipped in water. Some water climbs up in the gap between the plate. Let S be the surface tension of water P0= atmospheric pressure, P= pressure of water just below the water surface in the region between the plates-AP=P0−2SdBP=P0+2SdCP=P0−4SdDP=P0+4Sd
A capillary of internal radius 4mm, is dipped in water. To how much height, will the water rise in the capillary. (Twater=70×10−3N/m,g=10m/sec2,ρwater103kg/m3, contact angle θ→0)
View SolutionTwo parallel glass plates separated by a small distance x ar dipped partly in a liquid of density ' d keeping them vertical .The surface tension of the liquid is T and angle of contact is θ . What is the rise of the liquid between the plates due to capillarity ?
View SolutionTwo vertical parallel glass plates are partially submerged in water. The distance between the plates is d and the length is l. Assume that the water between the plates does not reach the upper edges of the plates and the wetting is complete. The water will rise to height (ρ= density of water and α= surface tension of water)
View SolutionTwo metal plates are separated by a distance d in a parallel plate condenser. A metal plate of thickness t and of the same area is inserted between the condenser plates. The value of capacitance increases by ….times.
View SolutionTwo parallel glass plates are dipped partly in the liquid of denstiy 'd' keeping them vertical. If the distance between the plates is 'x', Surface tension is T and angle of contact is θ then ries of liquid between the plates due to capillary will be
View Solution