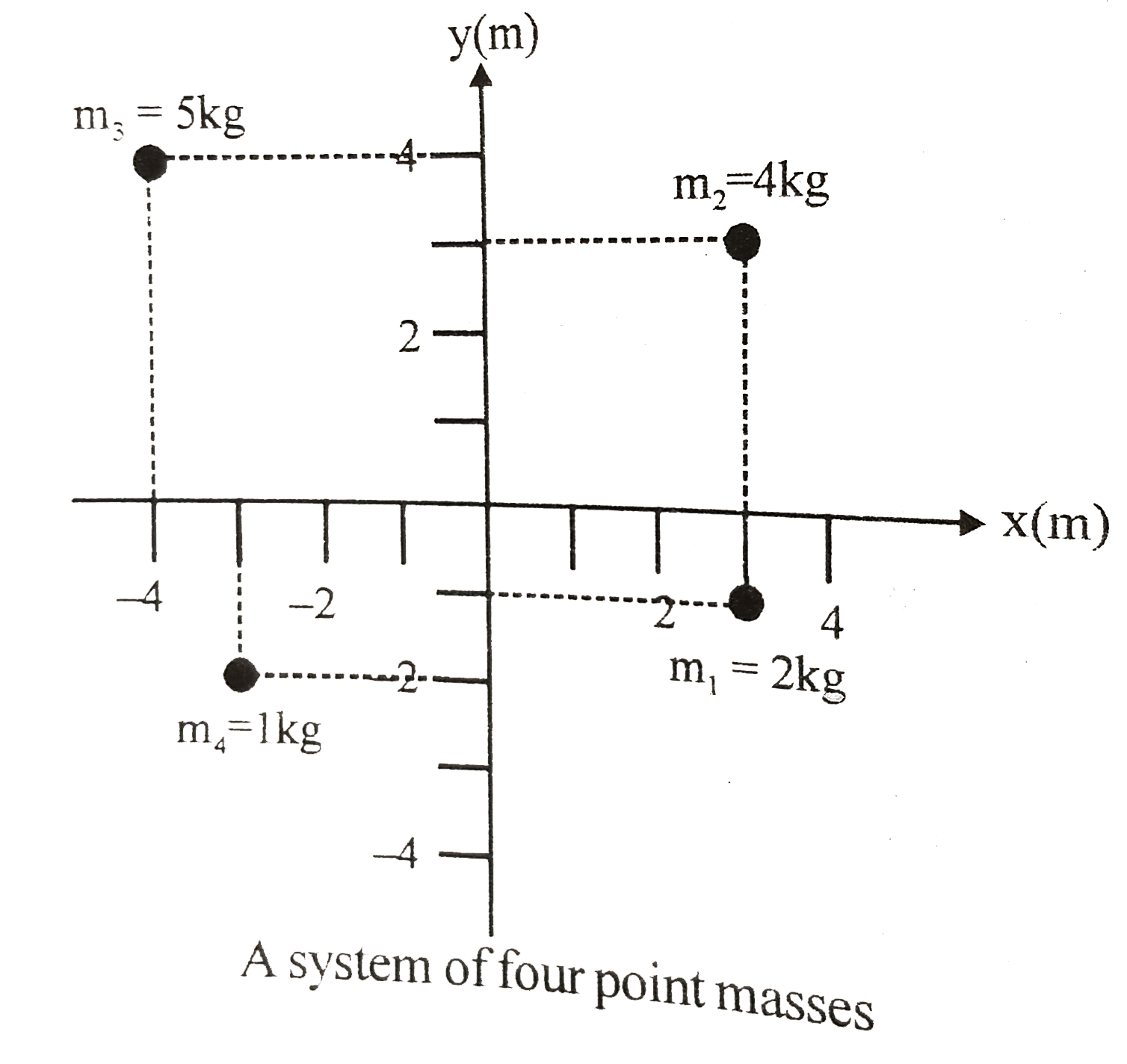
Find the center of mass of the four point masses as shown in figure.
Video Solution
Text Solution
The total mass M=12kg,
From equation we have, xcm=ΣmixiΣmi,ycmΣmiyiΣmi
xcm=(2kg)(3m)+(4kg)(3m)+(5kg)(−4m)+(1kg)(−3m)12kg=−512m
ycm=(2kg)(−1m)+(4kg)(3m)+(5kg)(4m)+(1kg)(−2m)12kg=2812m
The position of the cm is →rcm=−0.42ˆi+2.3ˆjm
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - Solved Examples15videos
CENTRE OF MASS & MOMENTUM CONSERVATION
- BOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - Practice Exercise20videos
CENTRE OF MASS & MOMENTUM CONSERVATION
- BOOK - BANSALCHAPTER - GRAVITATIONEXERCISE - EXERCISE -4 Section - B6videos
GRAVITATION
Similar Questions
Knowledge Check
- Question 1 - Select One
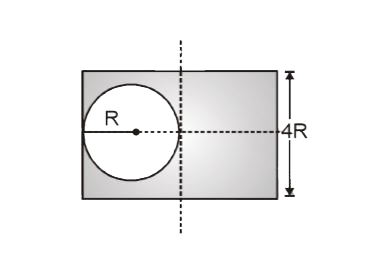
Find the position of center of mass of the uniform lamina shown in figure, if small disc of radius a2 is cut from disc of radius a.
 A(−a6,a)B(−a2,0)C(−a6,0)D(−a3,0)
A(−a6,a)B(−a2,0)C(−a6,0)D(−a3,0)
Find the position of centre of mass of the uniform lamina shown in figure.
 View Solution
View SolutionFind the velocity of center of mass of the system shown in the figure.
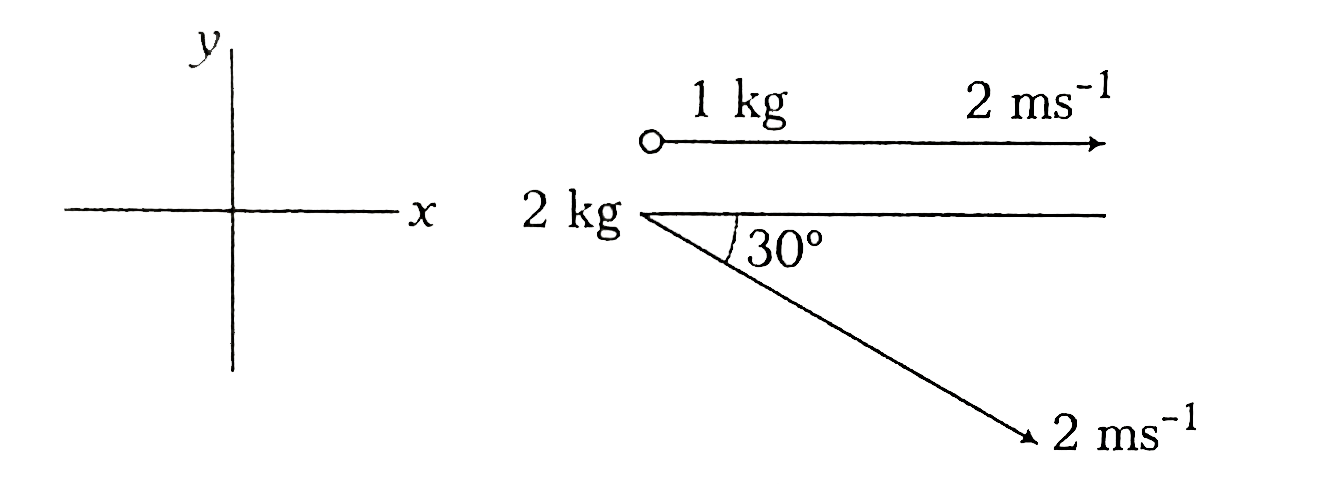 View Solution
View SolutionFind the center of mass of a uniform plate shown in Fig. 7.10a.
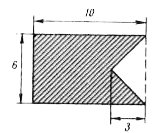 View Solution
View SolutionFind the position of centre of mass of the uniform lamina shown in figure. If the mass density of the lamina is σ.
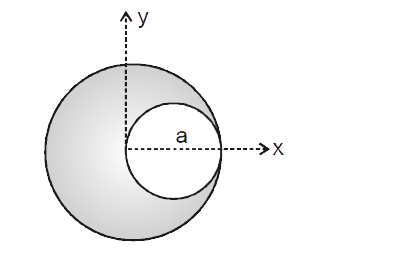 View Solution
View SolutionFind the centre of mass of the disc shown in figure. . A square is removed from the disc as shown
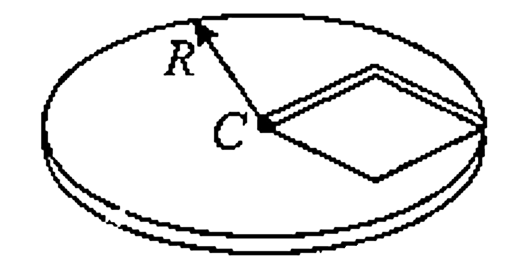 View Solution
View SolutionFind the position of centre of mass of the section shown in figure
 View Solution
View SolutionFind the co-ordinates of centre of mass of the lamina shown in figure
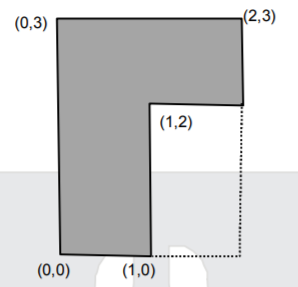 View Solution
View Solution