A satellite is launched tangentially from a height h above earth's surface as shown.
I. Find $v_{min}$ so that it just touches the earth's surface
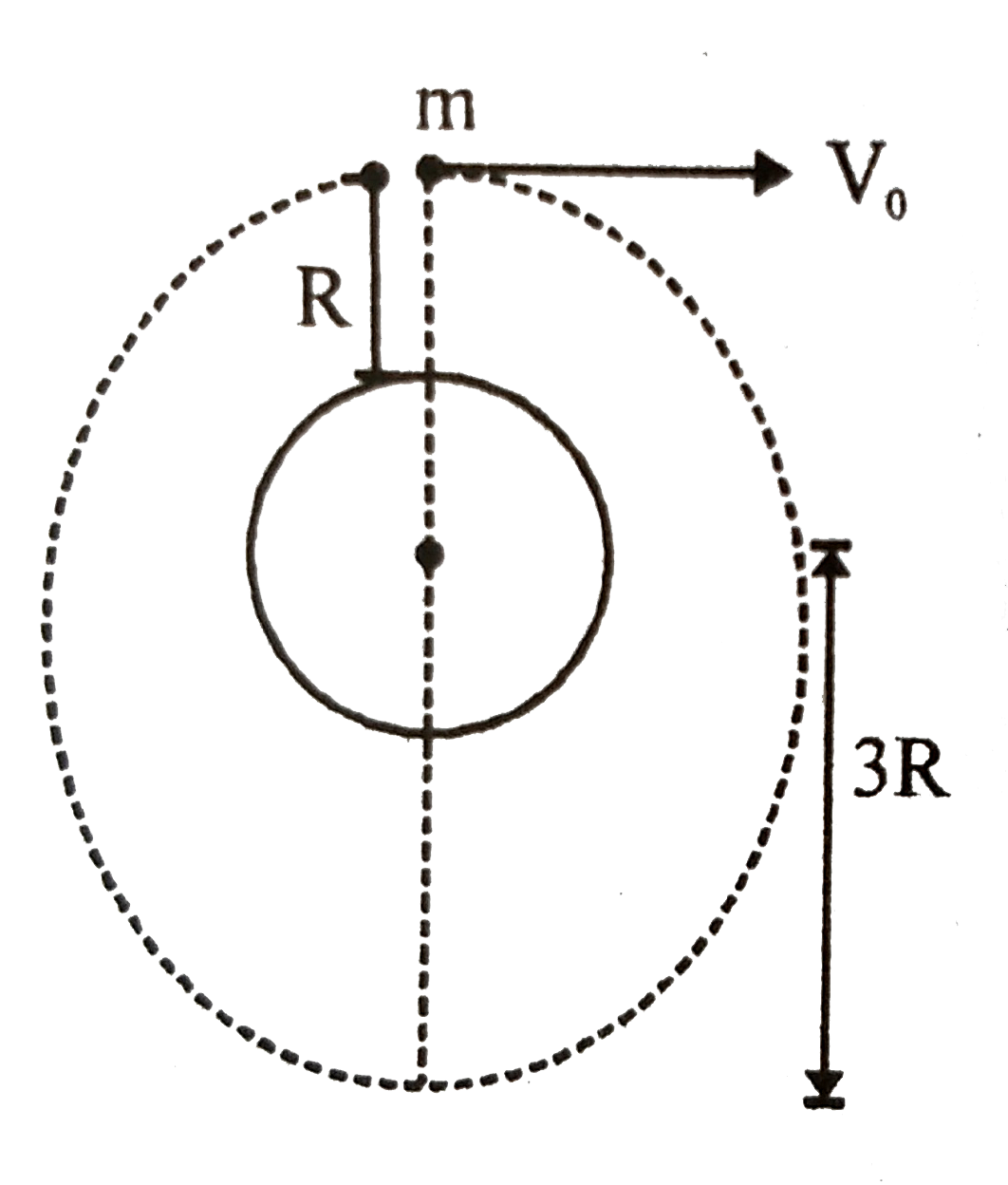
II. If h = R and satellite is launched tangentially with speed $= \sqrt{\frac{3 G M}{5 R}}$
find the maximum distance of satellite from earth's centre .

Text Solution

Verified by Experts

(I) Satellite just Grazes surface of earth
when $2 a = 2 R + h$
$a = (R + \frac{h}{2})$
Total energy $(E) = - \frac{G M m}{2 a} = - \frac{G M m}{(2 R + h)}$
$\frac{1}{2} m V_{0 min}^{2} - \frac{G M m}{R + h} = - \frac{G M m}{2 R + h}$
$V_{0 min}^{2} = 2 G M m [\frac{1}{R + h} - \frac{1}{2 R + h}]$
$= \frac{2 G M R}{(R + h) (2 R + h)} = \frac{2 G M R}{r (R + r)}$
$V_{0 min} = \sqrt{\frac{2 G M R}{r (R + r)}}$ where $r = h + R$

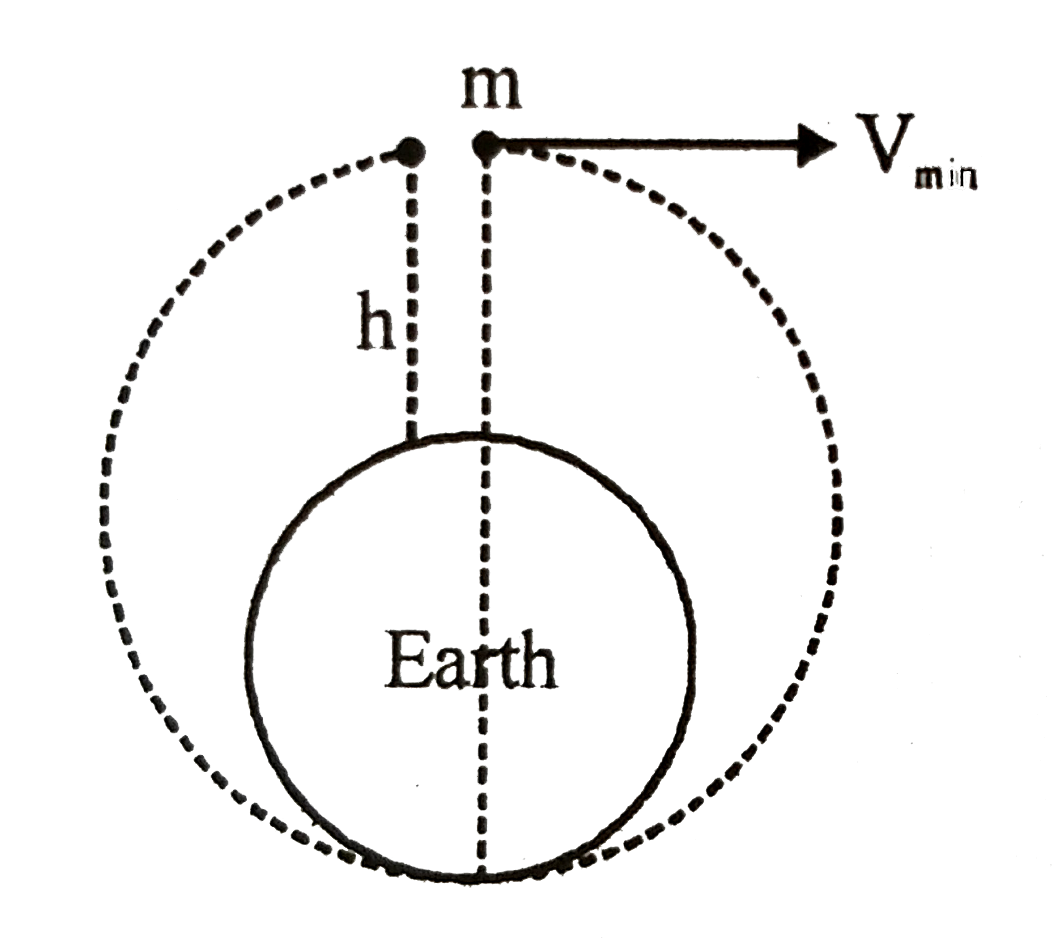
(II) To find maximum distance from centre.
$E = - \frac{G M m}{2 a} = \frac{1}{2} m v_{0}^{2} - \frac{G M m}{2 R}$
$= \frac{1}{2} m . \frac{3 G M}{5 R} - \frac{G M m}{2 R}$
$- \frac{1}{2 a} = \frac{3}{10 R} - \frac{1}{2 R} = - \frac{2}{10 R}$
$2 a = 5 R =$ Major axis
Required distance = 3R

Updated on:21/07/2023

A particle is launched from a height $8 R$ above the surface of earth and it is given a speed $(\frac{G M}{8 R})$ parallel to the surfaces then
AIt will escape fro the gravitational field of earth.
BIt will follow a circular orbit.
CIt will follow an elliptical orbit.
Dthe point of launching will be the perigee of its orbit.
A geostationary satellite is at a height $h$ above the surface of earth. If earth radius is $R$ -

AThe minimum colatitude on earth upto which the satellite can be used for communication is ${sin}^{- 1} (R / R + h)$
BThe maximum colatitudes on earth upto which the satellite can be used for communication is ${sin}^{- 1} (R / R + h)$
CThe area on earth escaped from this satellite is given as $(2 π R^{2} (1 + sin θ))$
DThe area on earth escaped from this satellite is given as $(2 π R^{2} (1 + cos θ)$
A satellite is revolving near by earth of radius $= R_{e}$ . If its velocity is increased $\sqrt{\frac{3}{2}} v$ where v is the orbital speed of satellite then find maximum distance of satellite from the center of the earth.
A $R_{e}$
B $2 R_{e}$
C $3 R_{e}$
D $4 R_{e}$