(Temperature of Sun)
Esimate the temperature $T_{c}$ of the earth, assuming it is in radiative equilibrium with the sun. (Assume radius of sun, $r_{s} = 7 \times 10^{8} m$ , temperature of solar surface $= 6000 K$ , distance of earth from sun, $R = 1.5 \times 10^{11} m$ )

Video Solution

Text Solution

Verified by Experts

Power radiated from sun $= σ \times$ surface area $\times T^{4}$
$= σ \times 4 π r_{s}^{2} \times T_{s}^{4}$
Power received by earth $= \frac{π r_{e}^{2}}{4 π R^{2}} \times$ power radiated by sun
since $π r_{e}^{2}$ is the effective area of the earth on which the sun's radiation is incident normally. figure and $4 π R^{2}$ is the total area on which the sun's radiation falls at a distance $R$ from the sun where the earth is situated.
Now power radiated by earth $= σ \cdot 4 π r_{e}^{2} \cdot T_{e}^{4}$

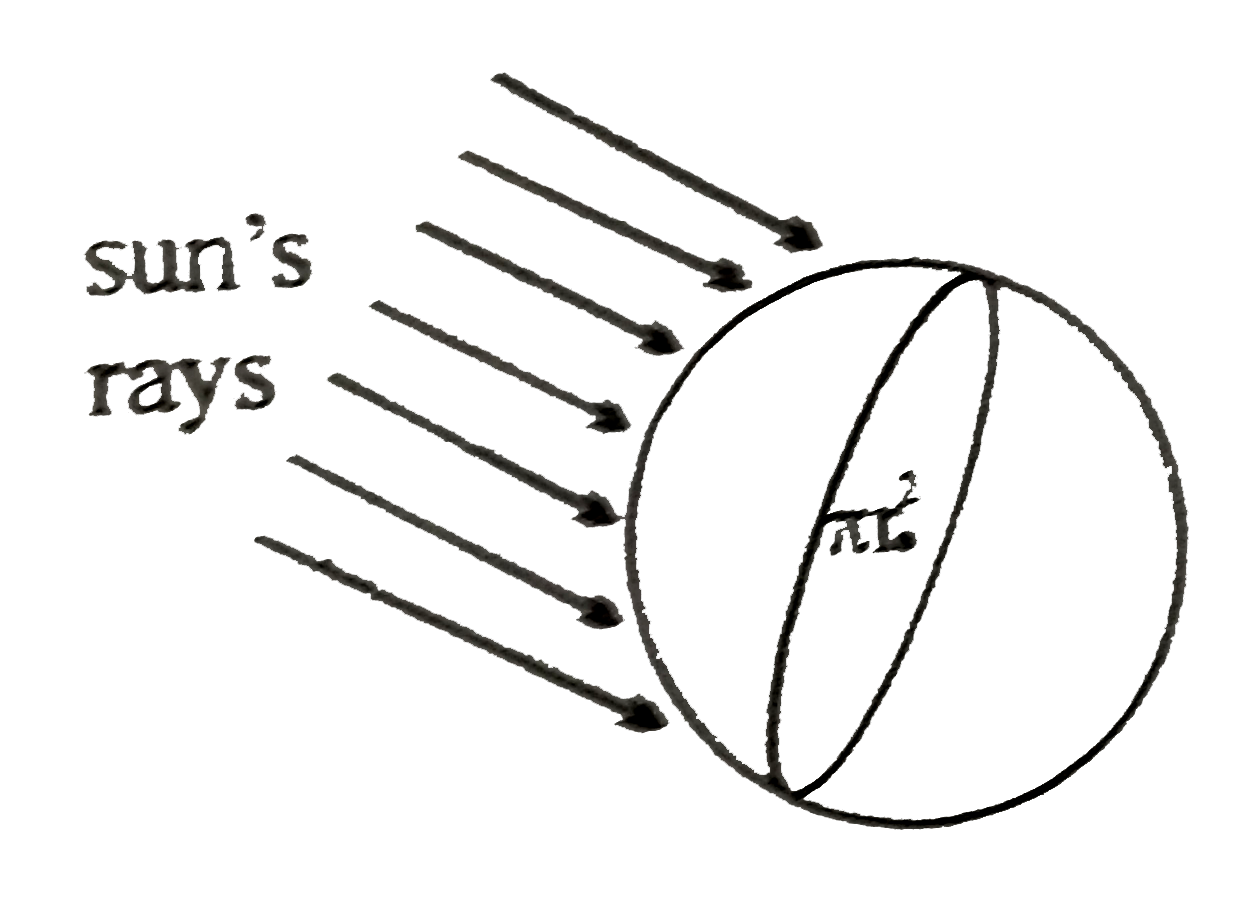
Assuming radiative equilibrium
power radiated by earth $=$ power received by earth
$∴ σ \cdot 4 π r_{e}^{2} \cdot T_{e}^{2} = σ \cdot 4 π r_{s}^{2} \cdot T_{s}^{4} \times \frac{π r_{c}^{2}}{4 π R^{2}}$
Cancelling $r_{e}^{2}$ and simplifying, then
$T_{e}^{4} = T_{s}^{4} \times (\frac{r_{s}^{2}}{4 R^{2}})$
$∴ T_{e} = T_{s} \times {(\frac{7 \times 10^{8}}{2 \times 1.5 \times 10^{11}})}^{1 / 2} = 209 K$
Note that the calculation is approximate, for example, the earth and the sun are not perfect black body radiators and the earth receives heat from its interior.

Updated on:21/07/2023

The time period of jupiter is 11.6 years, how far is jupiter from the sun. Distance of earth from the sun is $1.5 \times 10^{11} m$ .
A $4 \times 10^{11} m$
B $7.68 \times 10^{11} m$
C $9.11 \times 10^{11} m$
D $2 \times 10^{11} m$
If temperature of Sun=6000K, radius of Sun is $7.2 \times 10^{5} K m$ , radius of earth=6000 Km & distance
between earth and Sun $= 15 \times 10^{7}$ Km. find intensity of light on earth.
A $38.4 \times 10^{16}$
B $12.2 \times 10^{16}$
C $18.3 \times 10^{16}$
D $8.2 \times 10^{16}$
The surface temperature of the sun is $T_{0}$ and it is at average distance $d$ from a planet. The radius of the sun is $R$ . The temperature at which planet radiates the energy is
A $T_{0} \sqrt{\frac{R}{2 d}}$
B $T_{0} \sqrt{\frac{2 R}{d}}$
C $T_{0} \sqrt{\frac{R}{d}}$
D $T_{0} {(\frac{R}{d})}^{1 / 4}$