What is the mass of the goods that must be put on the piston so that the volume of the gas becomes halved?
56 kg
80 kg
110 kg
92 kg
The correct Answer is:B
P'V' =PV
⇒P=2P=8×105=P0+mg+MGS0
7×105×20×10−4−600=Mg
⇒M=80kg
Topper's Solved these Questions
- KINETIC THEORY OF GASESBOOK - BANSALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Reasoning Type
1 Videos
- KINETIC THEORY OF GASESBOOK - BANSALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Multiple Correct Choice Type
15 Videos
- KINETIC THEORY OF GASESBOOK - BANSALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Exercise 1
41 Videos
- HEAT TRANSFERBOOK - BANSALCHAPTER - HEAT TRANSFEREXERCISE - Exercise
89 Videos
- UNIT DIMENSION, VECTOR & BASIC MATHSBOOK - BANSALCHAPTER - UNIT DIMENSION, VECTOR & BASIC MATHSEXERCISE - Exercise
10 Videos
Similar Questions
A vertical cylinder of cross-sectional area 0.1m2 closed at both ends is fitted with a frictionless piston of mass M dividing the cylinder into two parts. Each part contains one mole of an ideal gas in equilibrium at 300K. The volume of the upper part is 0.1m3 and that of lower part is 0.05m3 .What force must be applied to the piston so that the volumes of the two parts remain unchanged when the temperature is increased to 500K?
View SolutionA vertical cylinder of cross-sectional area 0.1m2 closed at both ends is fitted with a frictionless piston of mass M dividing the cylinder into two parts. Each part contains one mole of an ideal gas in equilibrium at 300K. The volume of the upper part is 0.1m3 and that of lower part is 0.05m3 .What force must be applied to the piston so that the volumes of the two parts remain unchanged when the temperature is increased to 500K?
View Solution
Knowledge Check
In the cylinder shown in the figure, air is enclosed under the piston. Piston mass M=60 kg, crosssectional area of the cylinder S0=20cm2 atmospheric pressure P0=105paα=37∘. The air temperature is constant, the friction is negligible.

What is the mass of the goods that must be put on the piston so that the volume of the gas becomes half?A56kgB80kgC110 kgD92 kgThe volume of an ideal gas becomes zero at
A0∘CB273∘CC−273.15∘CD−273KAn insulating cylinder contains equal volumes of He and O2 separated by a massless freely moving adiabatic piston as shown. The gas is compressed by moving the insulating piston so that volume of He becomes half. Select the correct alternative(s).
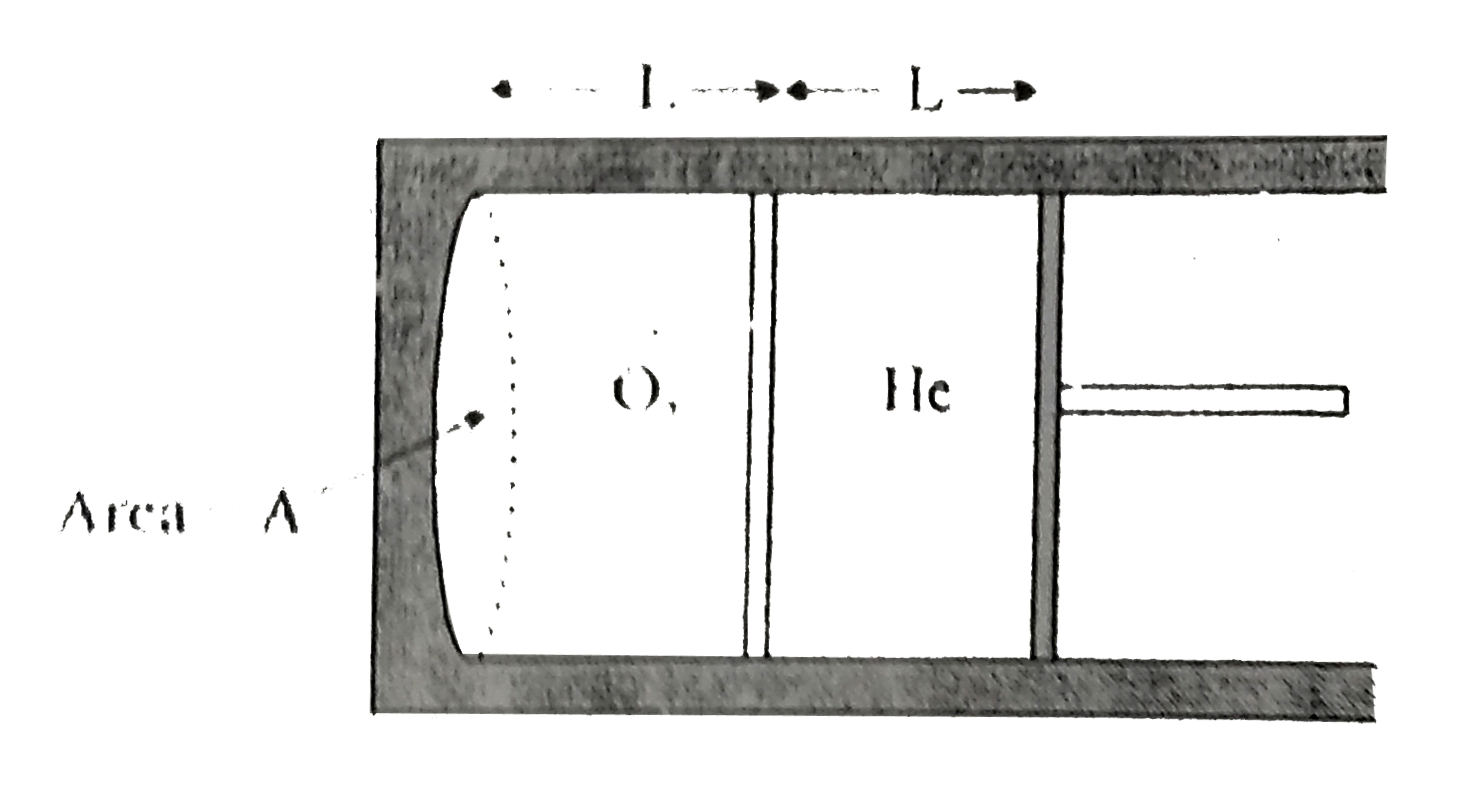 APressure in He chamber will be equal to pressure in O2 chamberBPressure in He chamber will be less then pressure in O2 chamberCVolume of He chamber will be equal to volume of O2 chamberDVolume of O2 chamber will be (LA)(2)25/21
APressure in He chamber will be equal to pressure in O2 chamberBPressure in He chamber will be less then pressure in O2 chamberCVolume of He chamber will be equal to volume of O2 chamberDVolume of O2 chamber will be (LA)(2)25/21
At what temperature, the volume of a gas would become zero ?
View SolutionThe pressure of a given mass of a gas is halved at constant temperature. What will be the volume of the gas in comparison to its initial volume? Explain on the basis of kinetic theory.
View SolutionIf the height and radii of the cylinder halved then the volume of the cylinder becomes ____
View SolutionTwo cylinder A and B having piston conneted by massless rod (as shown in figure). The cross-sectional area two cylinders are same & equal to 'S'.The cylinder A contains m gm of an ideal gas at Pressure P & temperature T0 The cylinder B contain identical gas at same temperature T0 but has different mass. The piston is held at the state in the position so that volume of gas in cylinder A & cylinder B are same and is equal to V0. The walls & piston of cylinder A are thermally insulated, whereas cylinder B is maintained at temperature T0 reservoir. The whole system is in vacuum. Now the piston is slowly released and it moves towards left & machanical equilibrium is reached at the state when the volume of gas in cylinder A becomes V_(0)/2 Then (here gamma for gas =1.5)
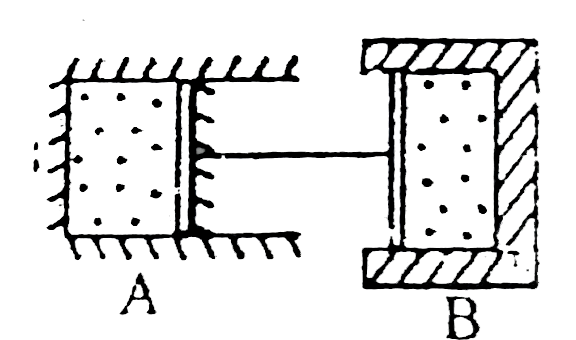
The mass of gas in cylinder B
View SolutionSuppose there are N molecules each of mass m, of an ideal gas in a container. The x component of velocity of a molecule is denoted by ux. The gas is enclosed using a horizontal piston of area A as shown.

If the piston is moved so as to reduce the volume of gas by half, keeping the temperature of gas constant , we know from the gas law that the pressure will be doubled. On microscopic level the increase in pressure on the piston is because
View Solution
