A block of mass m rests on a horizontal floor for which coefficient of friction is μ . Find the magnitude and the direction of force that should be applied to just move the block so that the applied force is minimum.
μmg
√1+μ2μmg
μ√1+μ2mg
μmg√1+μ2
The correct Answer is:D
Suppose the force F is applied at an angle θ with the horizontal as shown in adjoining figure For vertical equilibrium,

R+Fsinθ=mg
or R=mg−Fsinθ ….(i)
While for horizontal motion
Fcosθ≥f1 or Fcosθ≥μR ......(ii)
From eqns. (i) and (ii), we get,
Fcosθ≥μ(mg−Fsinθ)
or F≥μmg(cosθ+μsinθ)
For the force F to be the minimum (cosθ+μsinθ)
must be maximum.
i.e, ddθ(cosθ+μsinθ)=0
or −sinθ+μcosθ=0
i.e, tanθ=μorθ=tan−1(μ)
∴ sinθ=μ√1+μ2andcosθ=1√1+μ2
∴ F≥μmg1√1+μ2+μ√1+μ2
∴ Fmin=μmg√1+μ2
Topper's Solved these Questions
Similar Questions
A block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View SolutionA blocky of mass m rests on a horizotal floor with which it has a coefficient of static friction μ. It is desired to make the body move by applying the minimum possible force F. Find the magnitude of F and the direction in which it has be applies.
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass 2 kg is kept on a rough horizontal floor an pulled with a force F. If the coefficient of friction is 0.5. then the minimum force required to move the block is :-
 A10 NB10011NC1008ND20 N
A10 NB10011NC1008ND20 N - Question 2 - Select One
A block of mass m=5kg is resting on a rough horizontal surface for which the coefficient of friction is 0.2 . When a force F=40N is applied, the acceleration of the block will be (g=10m/s2) .
 A5.73m/sec2B8.0m/sec2C3.17m/sec2D10.0m/sec2
A5.73m/sec2B8.0m/sec2C3.17m/sec2D10.0m/sec2 - Question 3 - Select One
A blocky of mass m rests on a horizotal floor with which it has a coefficient of static friction μ. It is desired to make the body move by applying the minimum possible force F. Find the magnitude of F and the direction in which it has be applies.
AμmgB√1+μ2μmgCμ√1+μ2mgDμmg√1+μ2
Consider the situation shown in the figure . The coefficient of friction between the blocks is μ. Find the minimum and the maximum force F that can be applied so that two blocks move together.
 View Solution
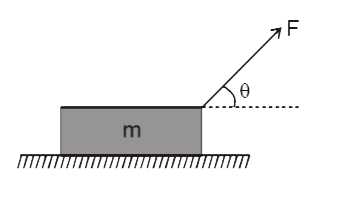
View SolutionA wooden block of mass m resting on a rough horizontal table (coefficient of friction =μ ) is pulled by a force F as shown in figure.The acceleration of the block moving horizontally is:
 View Solution
View SolutionA block A of mass 2 kg rests on another block B of mass 8 kg which rests on a horizontal floor. The coefficient of friction between A and B is 0.2 while that between B and floor is 0.5. when a horizontal force of 25 N is applied on the block B. the force of friction between A and B is
View SolutionAn inclined plane is inclined at an angle θ with the horizontal. A body of mass m rests on it, if the coefficient of friction is μ, then the minimum force that has to be applied to the inclined plane to make the body just move up the inclined plane is
View SolutionA block of mass m is placed on a rough horizontal surface. The coefficent of friction between them is μ. An external horizontal force is applied to the block and its magnitude is gradually increased. The force exerted by the block on the surface is R.
View Solution

