The area (in square unit) bounded by the parabola y=x2–1, the tangents drawn from the point (0,–5) to it isλμ then find the value of 3λ–μ is :
Text Solution
The correct Answer is:45.00
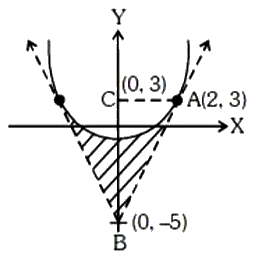
Equations of tangents from (0,−5) on y=x2−1 is
(y−52−1)2=4(x−y−1)2
i.e. y=4x−5 and y=−4x−5
Required area = 2[ar(ΔABC)−∫3−1√y+1dy]
=2(8−163)=163.
|
Similar Questions
Knowledge Check
The area (in sq. units) bounded by the parabola y=x2−1, the tangent at the point (2,3) to it and the y-axis is
A143B563C83D323Area bounded by the parabola y=x2−2x+3 and tangents drawn to it from the point P(1, 0) is equal to
A4√2 sq. unitsB4√23 sq. unitsC8√23 sq. unitsD16√23 sq. unitsThe area of the region bounded by the parabola (y−2)2=x−1, the tangent to the parabola at the point (2,3) and the x-axis is
A7 sq. unitsB6 sq. unitsC9 sq. unitsDNone of these
The area of the region bounded by the parabola (y−2)2=x−1, the tangent to the parabola at the point (2,3) and the x-axis is
View Solutionif .1μ2 is 1.5, then find the value of λ1λ2.
View SolutionIf the area (in sq. units) bounded by the parabola y2=4λx and the line y=λx,λ>0, is 19, then λ is equal to:
View SolutionThe area of the region bounded by the parabola (y−2)2=x−1, the tangent to the parabola at the point (2,3) and the X-axis is
View SolutionThe area of the region bounded by the parabola (y−2)2=x−1, the tangent to the parabola at the point (2,3) and the x-axis is
View Solution
