Each of the two square plates of a capacitor has sides of length l. The angle $θ$ between the two plates is very small. If the medium between the plates is air and the minimum distance between them is t, determine the capacitance of the capacitor.

Text Solution

Verified by Experts

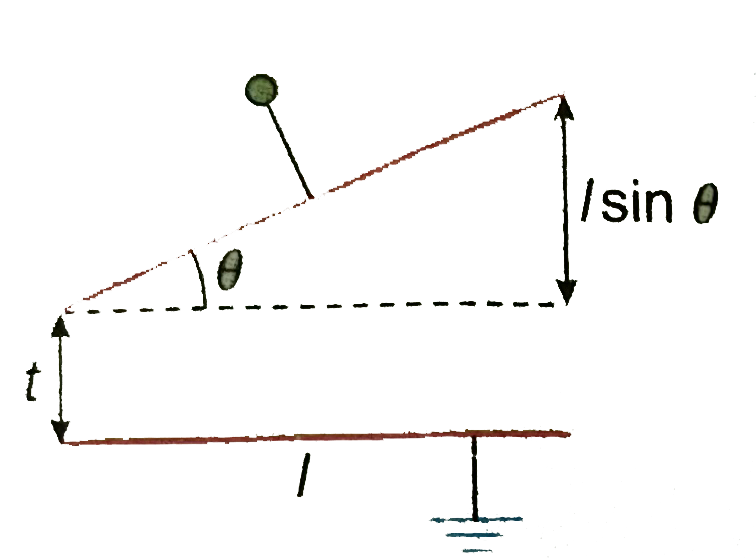
The average distance between the plates, $d = \frac{t + (t + l sin θ)}{2} = t \frac{l}{2} θ [∴ θ is very small, sin θ \approx θ]$
Capacitance of the capacitor,
$C = \frac{\in_{0} α}{d} = \frac{\in_{0} l^{2}}{t + \frac{l}{2} θ} = \frac{\in_{0} l^{2}}{t (1 + \frac{l θ}{2 t})} = \frac{\in_{0} l^{2}}{t} {(1 + \frac{l θ}{2 t})}^{- 1}$
$\approx \frac{\in_{0} l^{2}}{t} (1 - \frac{l θ}{2 t})$ .

Updated on:21/07/2023

If we increases the distance between two plates of the capacitor, the capacitance will.
Adecrease
Bremain same
Cincrease
Dfirst decrease then increase
A parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
A3C
B $\frac{C}{3}$
C12 C
D $\frac{C}{6}$
A parallel plate capacitor with air between the plates has a capacitance of 8pF. The separation between the plates is now reduced by half and the space between them is filled with medium of dielectric constant 5. The value of capacitance of a capacitor in the second case is
A0.8 pF
B3.2 pF
C80 pF
D40 pF