Show that the equivalent capacitance of an infinite circuit formed by the repetation of a similar loop made of two similar capacitors, each of capacitance C=(√5+1)μF, is 2μF.
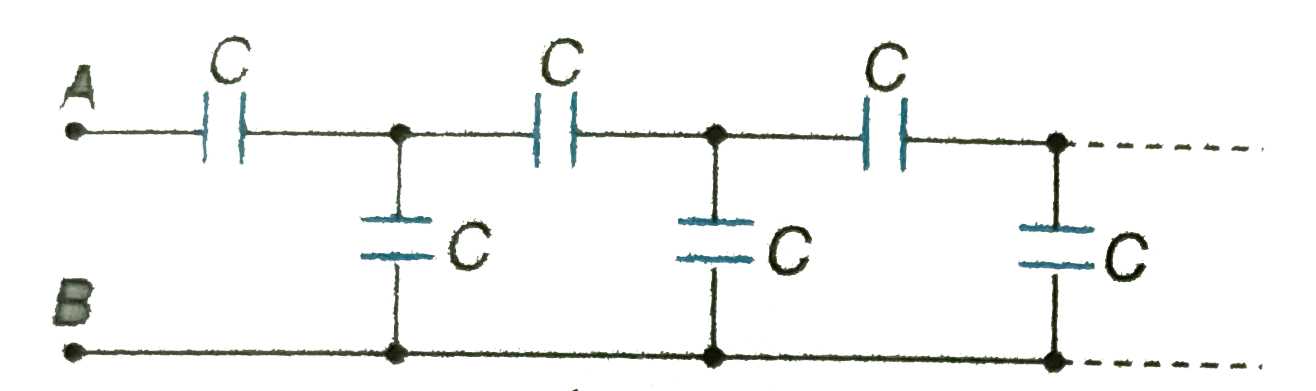
Let the capacitance of the infinite circuit be C'.
So the circuit on the right hand side starting from the second loop is also infinite. Therefore, its capacitance will also be C'.
In this case, the equivalent circuit will be that of Fig.4.27
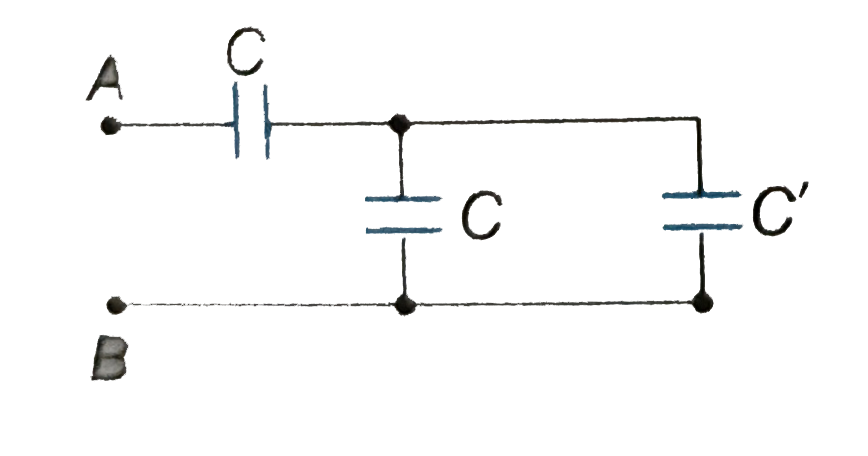
1C'=1C+1C'+C or, 1C'−1C'+C=1C
or, C'+C−C'C'(C'+C)=1C or, C'2+C'C−C2=0
C'=−C±√C2+4C22=−1±√5C2
Neglecting the negative value of C' we have.
C'=(√5−1)C2=(√5−1)2⋅(√5+1)=2μF.
Topper's Solved these Questions
- CAPACITANCE AND CAPACITORBOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - SECTION RELATED QUESTIONS
30 Videos
- CAPACITANCE AND CAPACITORBOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - HIGHER ORDER THINKING SKILL
39 Videos
- ATOMIC NUCLEUSBOOK - CHHAYA PUBLICATIONCHAPTER - ATOMIC NUCLEUSEXERCISE - CBSE SCANNER
14 Videos
- COMMUNICATION STSTEMBOOK - CHHAYA PUBLICATIONCHAPTER - COMMUNICATION STSTEMEXERCISE - CBSE SCANNER
28 Videos
Similar Questions
The equivalent capacitance of an infinite ladder of a circuit formed by the repetition of the same link consisting of identical capacitors, each with capacitance C , is
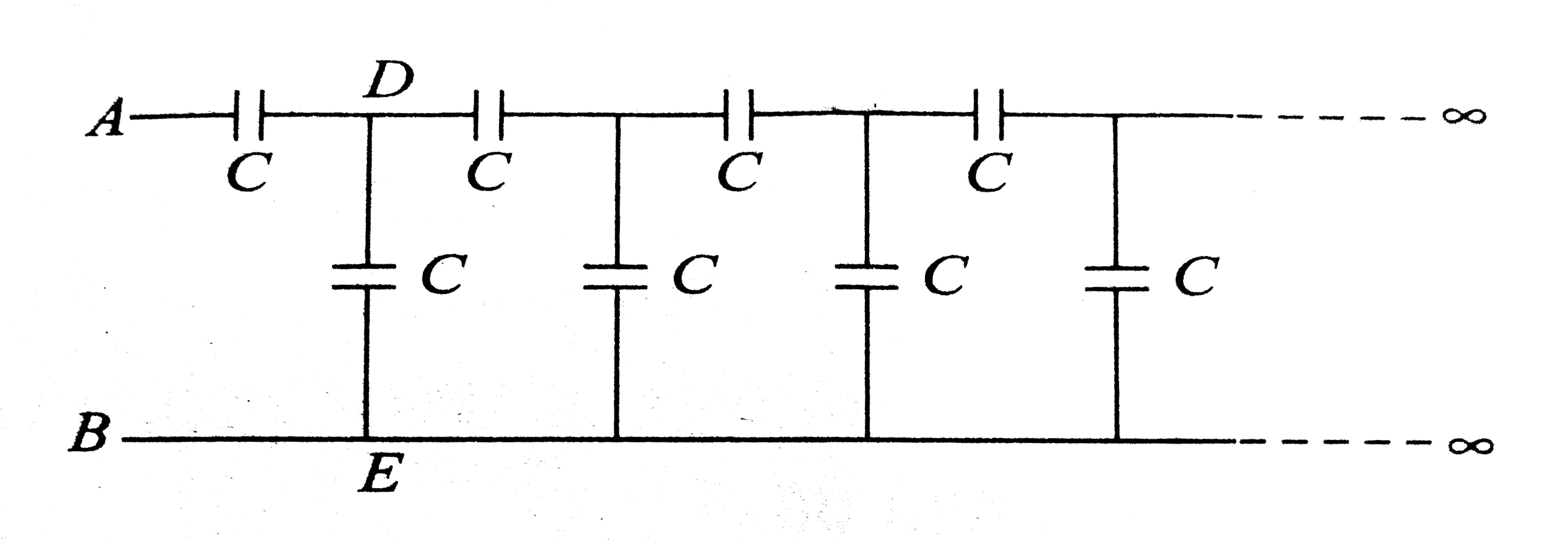 .
.
View Solution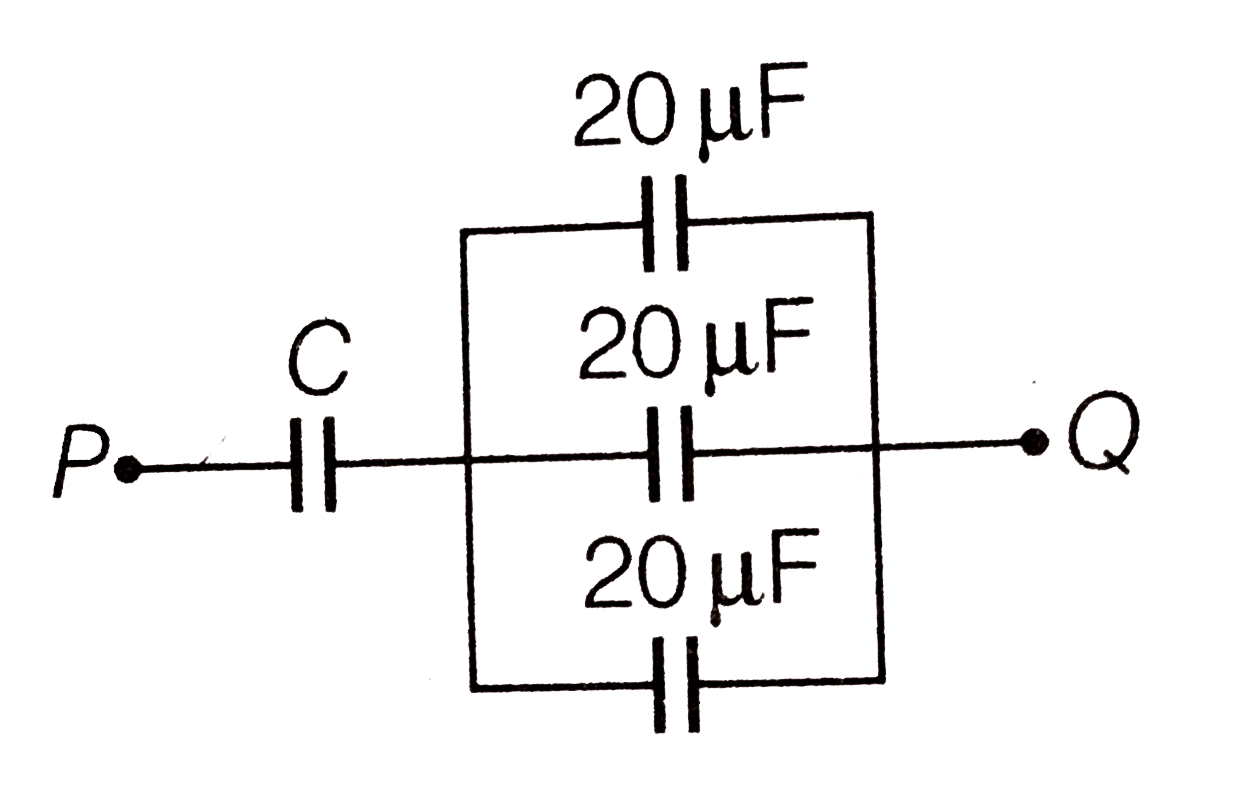
The equivalent capacitance of the combination shown in the figure between P and Q is 30μF, the capacitance of capacitor C is
View Solution
Knowledge Check
If the equivalent capacitance between points P and Q of the combination of the capacitors show in figure below us 30μF, the capacitor C is
 A60μFB30μFC10μFD5μF
A60μFB30μFC10μFD5μFShows a part of a circuit. If all the capacitors have a capacitance of 2μF, then the
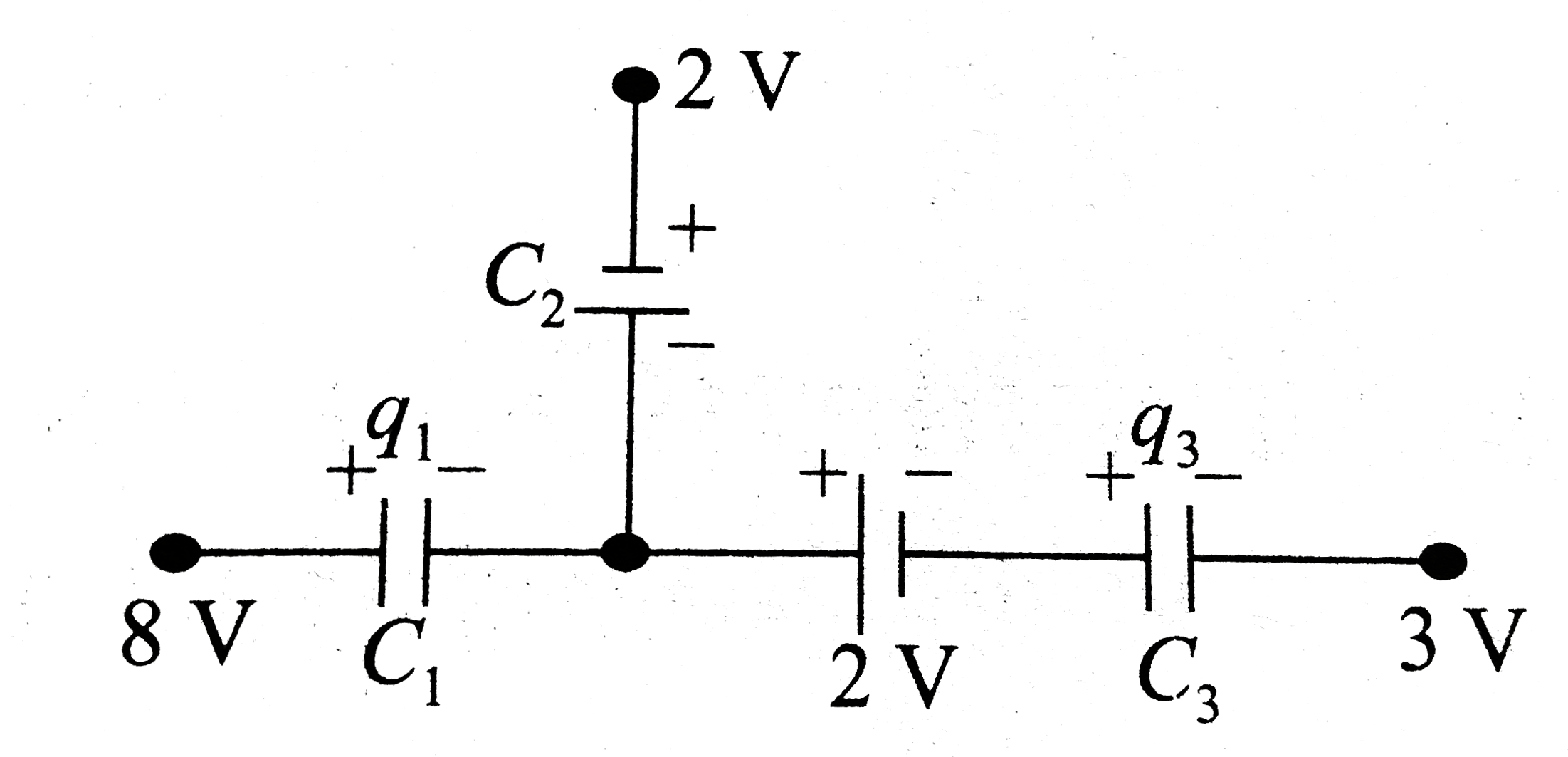 . Acharge on C3 is zeroBcharge on C3 is 12μCCcharge on C1 is 6μCDcharge on C1 in 6μC
. Acharge on C3 is zeroBcharge on C3 is 12μCCcharge on C1 is 6μCDcharge on C1 in 6μC
If the equivalent capacitance between points P and Q of the combination of the capacitors show in figure below us 30μF, the capacitor C is

View SolutionShows a part of a circuit. If all the capacitors have a capacitance of 2μF, then the
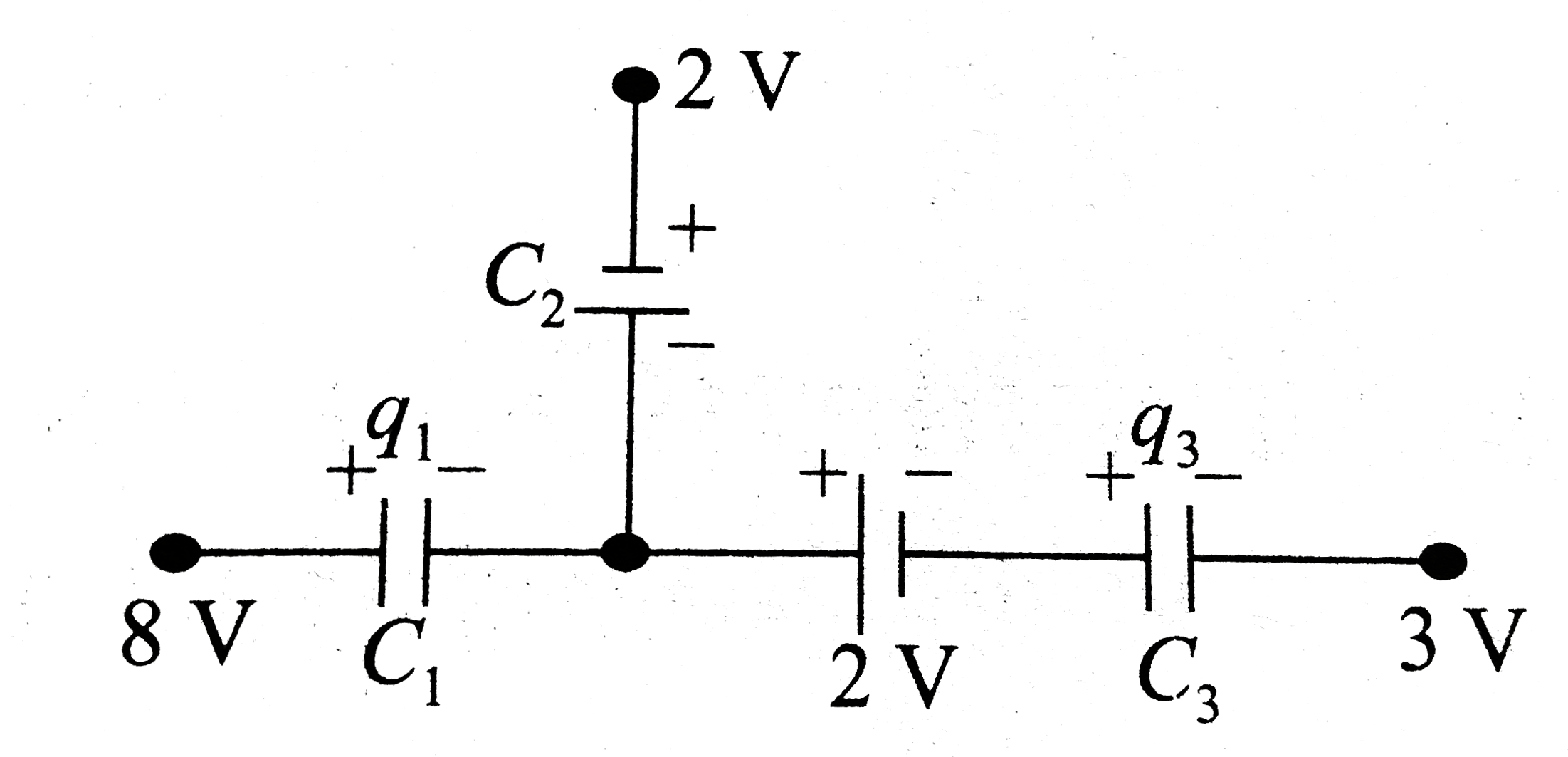 .
.
View Solution(i) Find equivalent capacitance between A and B in the combination give below . Each capacitor is a of 2μF capacitance .

View SolutionFind the equivalent capacitance between points A and B. Capacitance of each capacitor is 2μF.
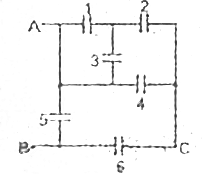
View SolutionFind the equivalent capacitance between points A and B. Capacitance of each capacitor is 2μF.

View SolutionFind the equivalent capacitance between terminals A & C, if each capacitor is of capacitance 4μF
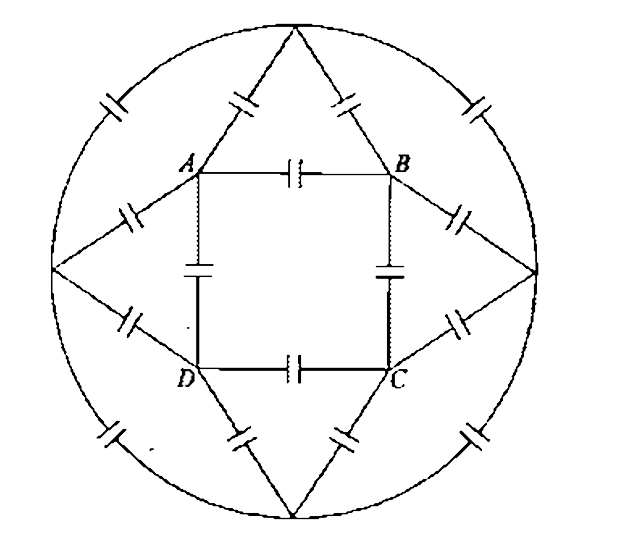
View Solution
