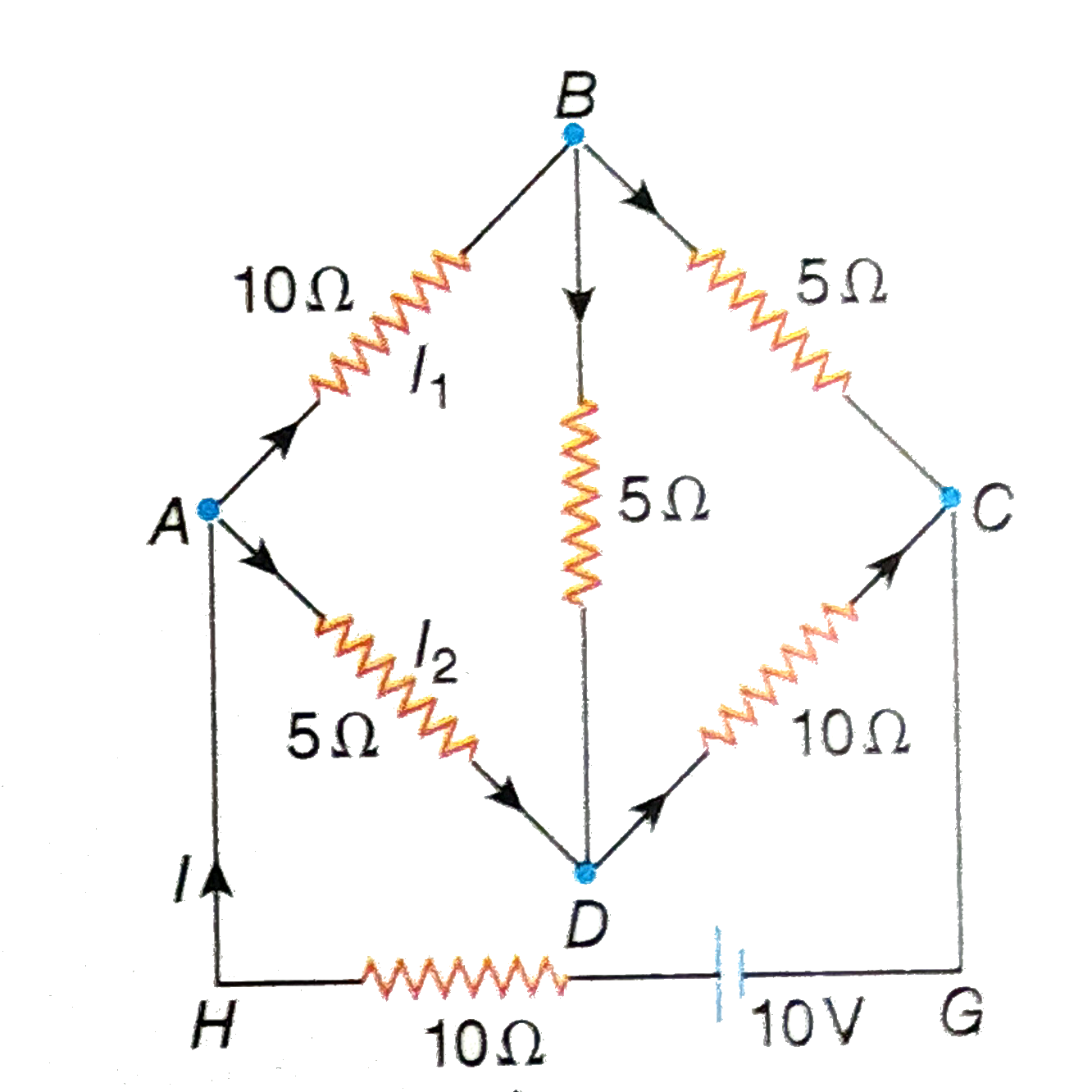
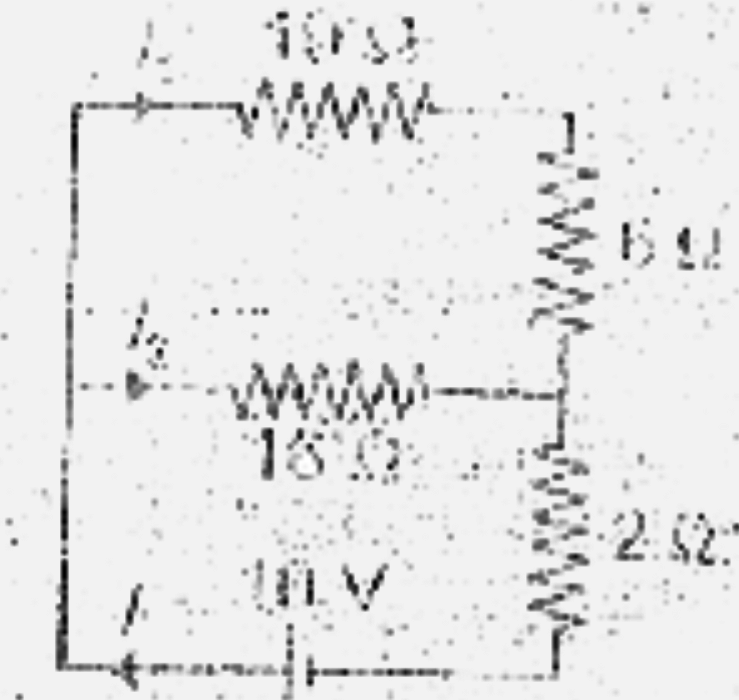
Determine the current in each branch of the network shown in Fig
Text Solution
Applying Kirchhoff's second law to the network ABDA , we get ,
10I1+5I2−5(I−I1)=0<br> or , 3I1+I2−I=0 ...(1)
For the loop BCDB ,
5(I1−I2)=0−10(I−I1+I2)−5I2=0 or , 3I1+4I2−2I=0 ...(2)
For the loop ABCGHA ,
10I+10I1+5(I1−I2)0 or , 2I1+3I2−I2=2 ...(3)
Solving the equations we get
I=1017A,I1=417A,I2=−217A
∴ Current through AB=417A
Current theough DC=(I−I1+I2)
=1017−417−217=417A
Current trough BD=217A
Current through AD=(I−I1)=10−417=617A
|
Topper's Solved these Questions
- KIRCHHOFF'S LAWS AND ELECTRICAL MEASUREMENT BOOK - CHHAYA PUBLICATIONCHAPTER - KIRCHHOFF'S LAWS AND ELECTRICAL MEASUREMENT EXERCISE - NCERT EXEMPLAR QUESTIONS WITH ANSWER HINT (MCQ -1)
2 Videos
- KIRCHHOFF'S LAWS AND ELECTRICAL MEASUREMENT BOOK - CHHAYA PUBLICATIONCHAPTER - KIRCHHOFF'S LAWS AND ELECTRICAL MEASUREMENT EXERCISE - NCERT EXEMPLAR QUESTIONS WITH ANSWER HINT (MCQ -2)
1 Videos
- KIRCHHOFF'S LAWS AND ELECTRICAL MEASUREMENT BOOK - CHHAYA PUBLICATIONCHAPTER - KIRCHHOFF'S LAWS AND ELECTRICAL MEASUREMENT EXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
20 Videos
- ELEMENTARY PHENOMENA OF ELECTROSTATICS BOOK - CHHAYA PUBLICATIONCHAPTER - ELEMENTARY PHENOMENA OF ELECTROSTATICS EXERCISE - EXAMINATION ARCHIVE
2 Videos
- LIGHT WAVE AND INTERFERENCE OF LIGHT BOOK - CHHAYA PUBLICATIONCHAPTER - LIGHT WAVE AND INTERFERENCE OF LIGHT EXERCISE - CBSE SCANNER
15 Videos
Similar Questions
Determine current in each branch of the network shown in figure

View SolutionFind the current in each branch of the cirucit shown in figure
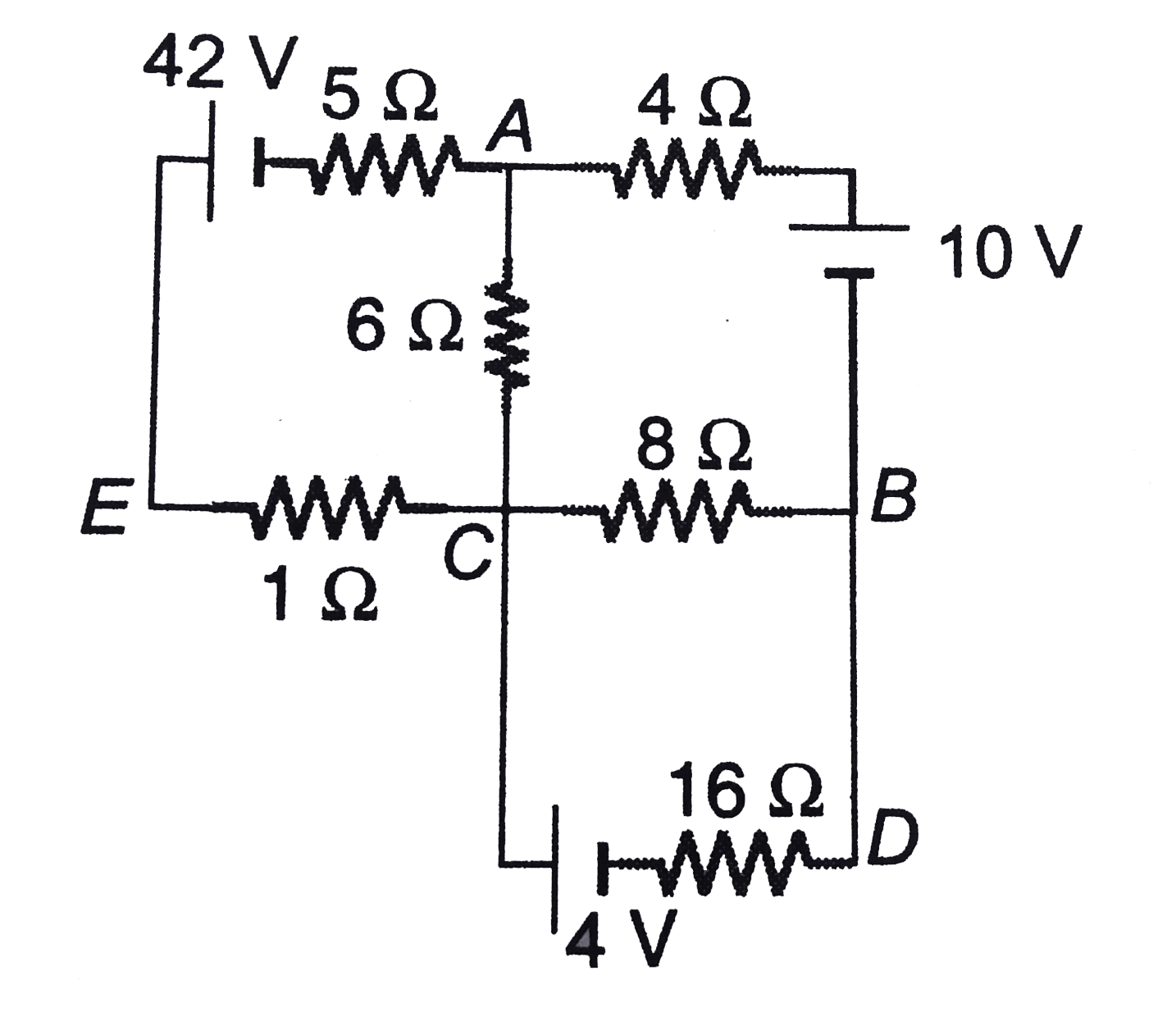
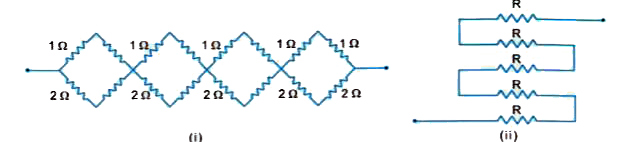
View SolutionDetermine the equivalent resistance of networks shown in Fig. (i) and (ii).
View SolutionThe current in the branch of a circuit shown below is
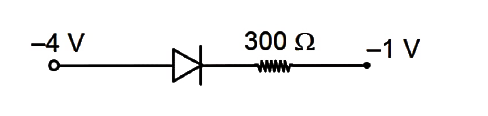
View SolutionThe current in the branch of a circuit shown below is

View SolutionFind the current in each resistor in the circuit as shown in fig.

View SolutionFind the current in each resistor in the circuit as shown in fig.

View SolutionCurrent I in the network shown in figure
View Solution
Find potential difference between points A and B of the network shown in fig. and distribution of given main current through different resistors.
View SolutionCurrent l in the network shown in figure is

View Solution
