Two circular coils of radii a and 2a having a common centre, carry identical current I but in opposite directions. Number of turns of the second conductor is 8. show that magnetic field intensity at the centre is 3 times that due to the smaller one. Also find out the change in the previous when current flow in the same direction through both the coils.

Text Solution

Verified by Experts

Mangnetic field intensity at the centre O due to the smaller loop is,
$B_{1} = \frac{μ_{0} i}{2 a}$ (upwards)
Similarly magnetic field intensity at the centre due to the bigger loop,
$B_{2} = 8 \times \frac{μ_{0} i}{2 (2 a)} = \frac{2 μ_{0} i}{a}$ (upwards)

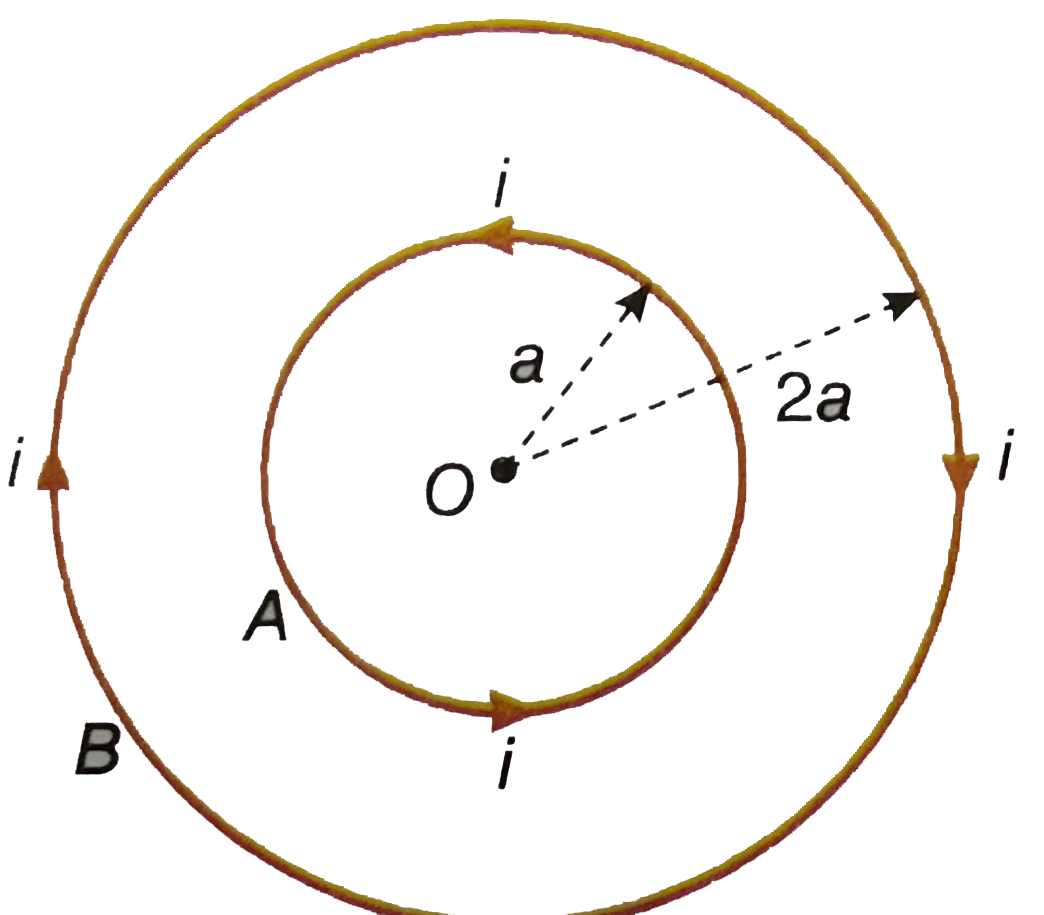
$∴$ Net magnetic field at O
$B = B_{2} - B_{1}$
$= \frac{μ_{o} i}{a} (2 - \frac{1}{2})$
$= \frac{3 μ_{0} i}{2 a} = 3 B_{1} [∴ B_{1} = \frac{μ_{0} i}{2 a}]$
Hence resultant field is 3 times that due to the smaller lopp.
Now if the direction of the current is same for both the loops, the resultant field will be,
$B = B_{1} + B_{2} = \frac{μ_{0} i}{2 a} + \frac{2 μ_{0} i}{a} = 5 \frac{μ_{0} i}{2 a} = 5 B_{1}$
Hence the resultant field will be 5 times that due to the smaller loop is current flows in the same direction.

Updated on:21/07/2023

When the current flowing in a circular coil is doubled and the number of turns of the coil in it is halved, the magnetic field at its centre will become
AFour times
BSame as at $Q$
CHalf
DDouble
Two concentric coils carry the same current in opposite directions. The diameter of the inner coil is equal currents. The outer coil. If the magnetic field produced by the outer coil at the common centre are $1 T$ , the net field at the centre is
A $1 T$
B $2 T$
C $3 T$
D $4 T$
A circular coil carrying a certain current produces a megnetic field $B_{o}$ at its centre. The coil is now rewound so as to have 3 turns and the same current is passed through it. The new magnetic field at the centre is
A $B_{o} / 2$
B $9 B_{o}$
C $B_{o} / 3$
D $3 B_{o}$