A wire loop is formed by joining two semicircular wires or radii r1andr2 as shown in the figure. If the loop caries a current, I, find the magnetic field at the centre O.
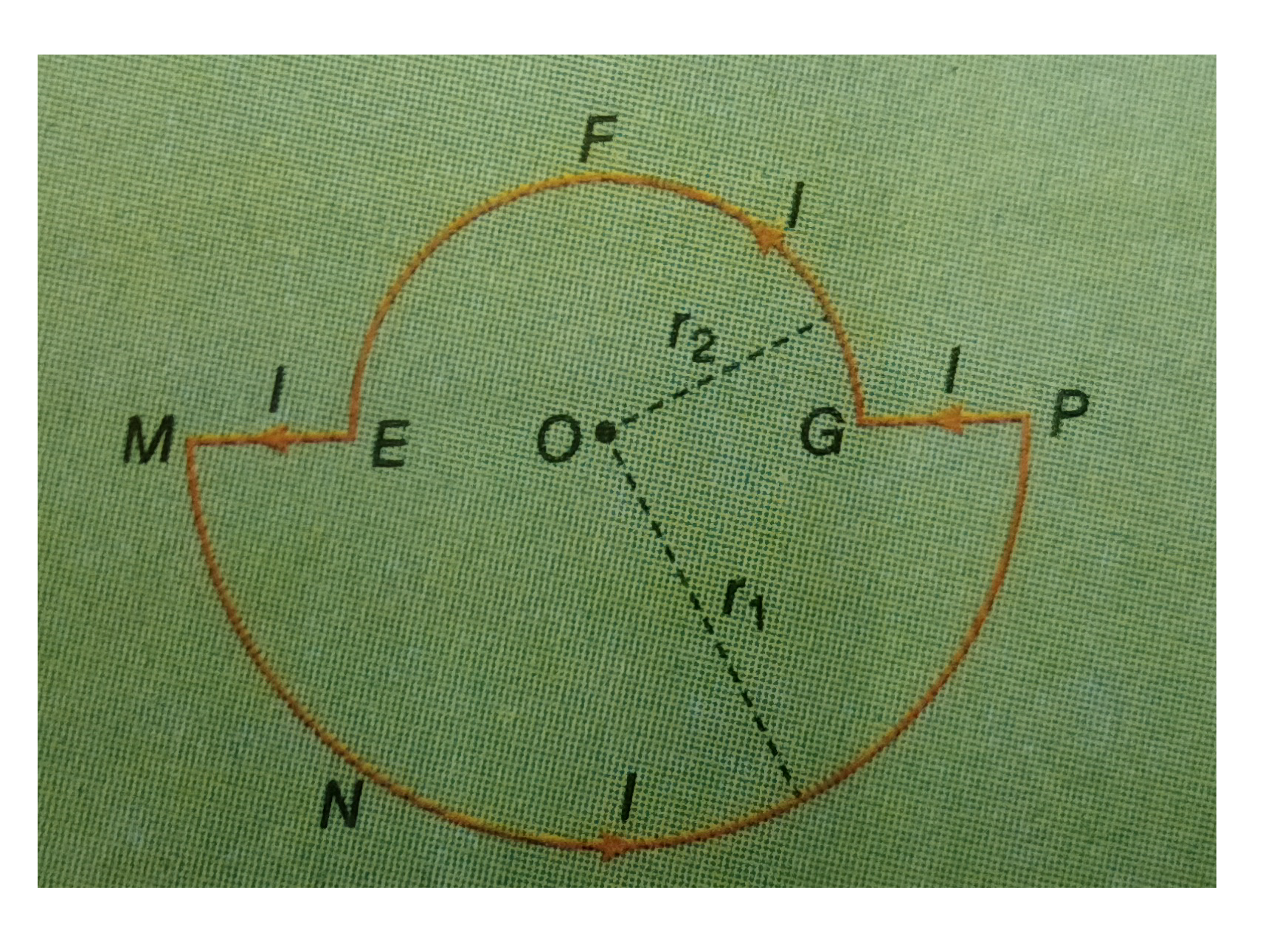
Magnetic field at the point O due to the semicircular part MNP is,
BMNP=μ04⋅Ir1 (upward)
Similarly magnetic field at O due to the semicircular part GEF is,
BGFE=μ04⋅Ir2 (upwards)
As the point O lies along the straight part ME and Pg of the loop, the magnetic field due to them at O is zero.
So, net magnetic field at O due to the whole loop,
B=B1+B2
=μ04⋅Ir1+μ04⋅Ir2
∴B=μ04I[1r1+1r2] (upwards)
Topper's Solved these Questions
- ELECTROMAGNETISM BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETISM EXERCISE - SECTION RELATED QUESTIONS
64 Videos
- ELECTROMAGNETISM BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETISM EXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
48 Videos
- ELECTROMAGNETIC WAVES BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVES EXERCISE - CBSE SCANNER
17 Videos
- ELEMENTARY PHENOMENA OF ELECTROSTATICS BOOK - CHHAYA PUBLICATIONCHAPTER - ELEMENTARY PHENOMENA OF ELECTROSTATICS EXERCISE - EXAMINATION ARCHIVE
2 Videos
Similar Questions
The wire loop shown in figure carries a current as shown. The magnetic field at the centre O is ,

View SolutionTwo wire loop PQRSP formed by joining two semicircular wires of radii R1andR2 carries a current i as shown in the figure given below. What is the magnetic field induction at the centre O in cases (A) and (B) ?
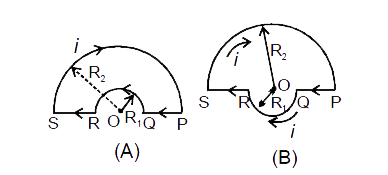
View Solution
Knowledge Check
The wire loop PQRS formed by joining two semi circular wires of radii R1, and _(2)Ry carries a current I as shown the figure. The inagnitude of magnetic induction at the centre O is
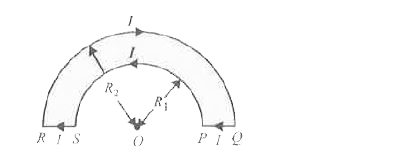 Aμ0I4(1R2−1R1)Bμ0I4(1R1−1R2)Cμ0I(1R2−1R1)Dμ0I(1R1−1R2)
Aμ0I4(1R2−1R1)Bμ0I4(1R1−1R2)Cμ0I(1R2−1R1)Dμ0I(1R1−1R2)The wire loop shown in figure carries a current as shown. The magnetic field at the centre O is ,
 AzeroBμ0I4(1R1−1R2)Cμ0I4(1R2+1R1)Dμ0I2(1R1−1R2)
AzeroBμ0I4(1R1−1R2)Cμ0I4(1R2+1R1)Dμ0I2(1R1−1R2)The wire loop ABCDEA formed by joining two semicircular loops of radiiR1andR2carries a current I. What is the magnetic induction at the center O in the following figure?
Aμ0i4(1R1−1R2)Bμ0i4(R2−R2)Cμ0i4(R2+R2)Dμ0i4(1R2−1R2)
A wire loop formed by joining two semicircular wires of radii R1 and R2 carries a current I as shown in figure. Find the magnetic field induction at the centre O.

View SolutionThe wire loop PQRSP formed by joining two semicircular wires of radii R1 and R2 carries a current I as shown . The magnitude of the magnetic induction at the center C is ………..
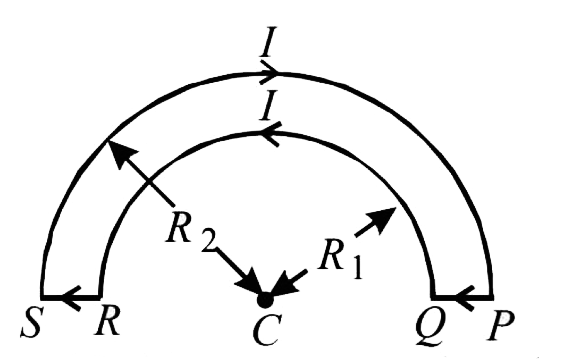
View SolutionThe wire loop PQRSP formed by joining two semicircular wires of radii R1 and R2 carries a current I as shown . The magnitude of the magnetic induction at the center C is ………..

View SolutionThe wire loop PQRSP formed by joining two semicircular wires of radii R1 and R2 carries a current I as shown. The magnitude of the magnetic induction at the centre C is ____________.

View SolutionThe current-loop PQRSTP formed by two circular segments of radii R1 and R2 carries a current of I ampers. Find the magnetic field at the common centre O. what will be the field if angle α=90∘ ?
View Solution
