Find the remainder when 12013+22013+32013+...+20122013 is divisible by 2013
Video Solution
Text Solution
12013+22013+32013+...+20122013
=(12013+20122013)+(22013+20112013)+...(10062013+10072013)
So, these terms are of the form an+bn and they will be divided by a+b as n=2013 and it is odd.
∴ Remainder of the given expression when divided by 2013 is 0.
|
Similar Questions
Knowledge Check
2013×?…..1=62403
A1B2C3D4The population of a village in Madurai is increasing year after year. Find out the population (in lakhs) in 2013 from the following information:
Year 2008 2009 2010 2011 2012 2013
Population 4 7 13 22 34 ?A49B46C45D43If sin−1x+sin−1y+sin−1z=3π2, Then x2013+y2013+z2013−9x2013+y2013+z2013 is equal to
A1B0C-1D-3
Real root of the equation
(x−1)2013+(x−2)2013+(x−3)2013+……….+(x−2013)2013=0 is a four digit number. Then the sum of the digits is :
View SolutionReal root of the equation
(x−1)2013+(x−2)2013+(x−3)2013+……….+(x−2013)2013=0 is a four digit number. Then the sum of the digits is :
View SolutionFind the percentage increase in sales from 2012 to 2013
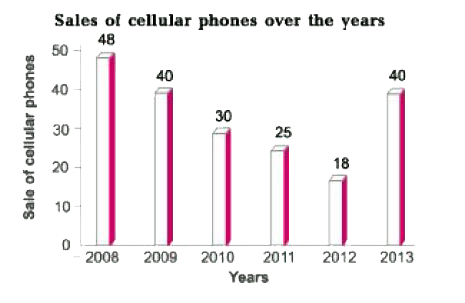
View SolutionIf sin−1x+sin−1y+sin−1z=3π2, Then x2013+y2013+z2013−9x2013+y2013+z2013 is equal to
View SolutionThe digit in the unit place of 172013+112013−72013
View Solution
