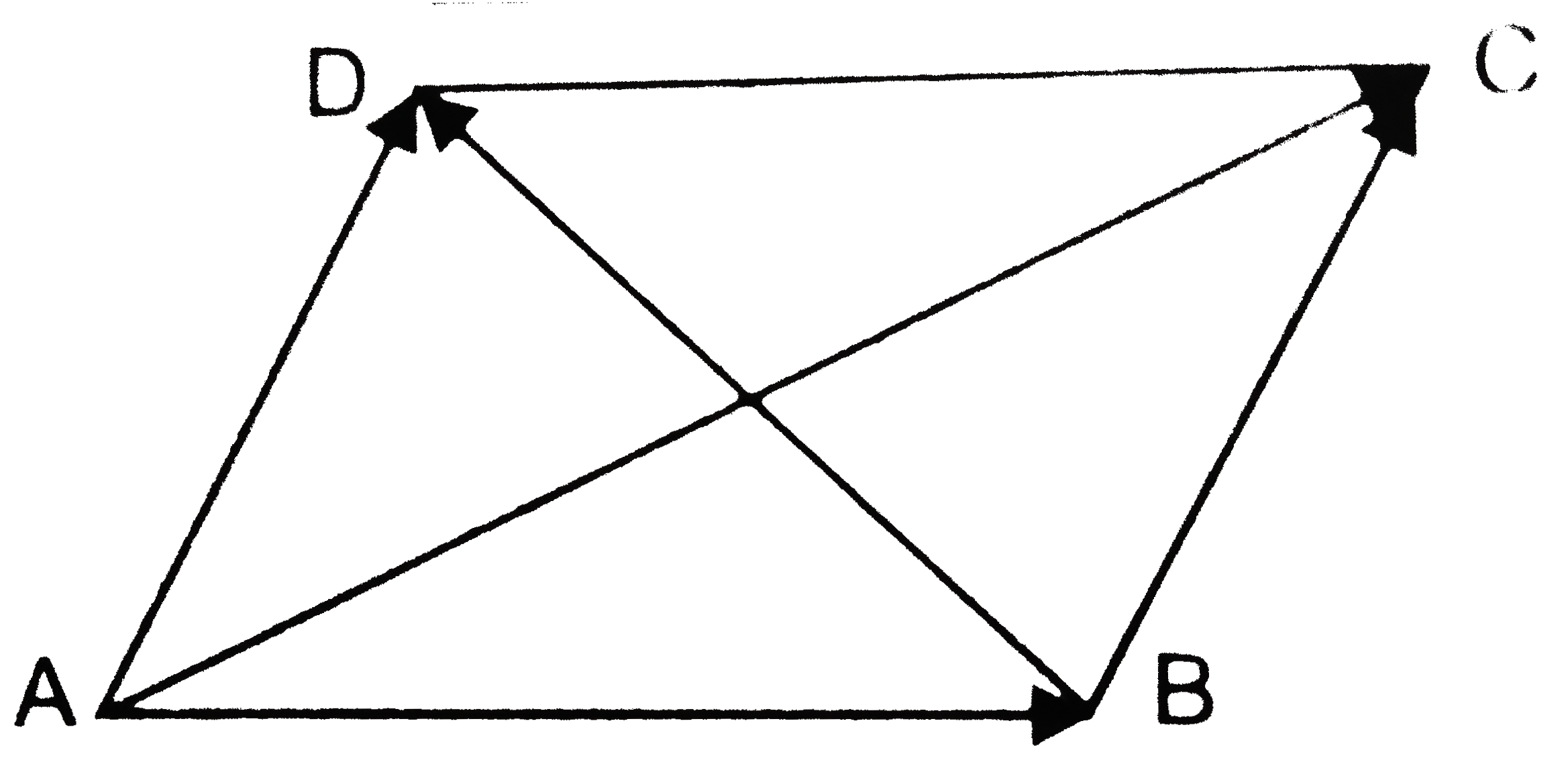
If A,B,C and D are four points and ¯¯¯¯¯¯AB=¯¯¯¯¯¯DC then ¯¯¯¯¯¯AC+¯¯¯¯¯¯BD= (A) 2−−→AD (B) 2−−→CB (C) 2−−→AC (D) none
Step by step video & image solution for If A, B, C and D are four points and bar (AB) = bar (DC) then bar (AC) +bar (BD) = (A) 2vec(AD) (B) 2vec(CB) (C) 2vec(AC) (D) none by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If A, B, C, D be any four points and E and F be the middle points of AC and BD respectively, then A→B+C→B+C→D+−−→AD is equal to
A3−−→EFB4−−→EFC4−−→FED3−−→FEIn a quadrilateral ABCD, ¯¯¯¯¯¯AC⊥¯¯¯¯¯¯BD and AB=AD. ABCD is a ________
ATrapeziumBRhombusCRectangleDKite
ABCD is a parallelogram Fig. 2 (c ) .64. ACand(BD)areitsdiagonals.Showtˆ<br> (a) vec (AC) +vec (BD) =2 vec (BC)<br>(b)vec (AC) - vec (BD) =2 vec (AB)
 .
.
View SolutionA, B, C, D are four points in the space and satisfy ∣∣∣−−→AB∣∣∣=3,∣∣∣−−→BC∣∣∣=7,∣∣∣−−→CD∣∣∣=11and∣∣∣−−→DA∣∣∣=9. Then find the value of −−→AC⋅−−→BD.
View SolutionA, B, C, D are four points in the space and satisfy ∣∣∣−−→AB∣∣∣=3,∣∣∣−−→BC∣∣∣=7,∣∣∣−−→CD∣∣∣=11and∣∣∣−−→DA∣∣∣=9. Then find the value of −−→AC⋅−−→BD.
View SolutionIf A,B,C,D are any four points in space prove that −−→AB×−−→CD+−−→BCx−−→AD+−−→CA×−−→BD=2−−→AB×−−→CA
View SolutionIf A,B,C,D are any four points in space prove that −−→AB×−−→CD+−−→BCx−−→AD+−−→CA×−−→BD=2−−→AB×−−→CA
View SolutionABCDE is a regular hexagon. i) If ¯¯¯¯¯¯AB+¯¯¯¯¯¯AE+¯¯¯¯¯¯BC+¯¯¯¯¯¯DC+¯¯¯¯¯¯ED+¯¯¯¯¯¯AC=λ¯¯¯¯¯¯AC then λ=
View Solution
