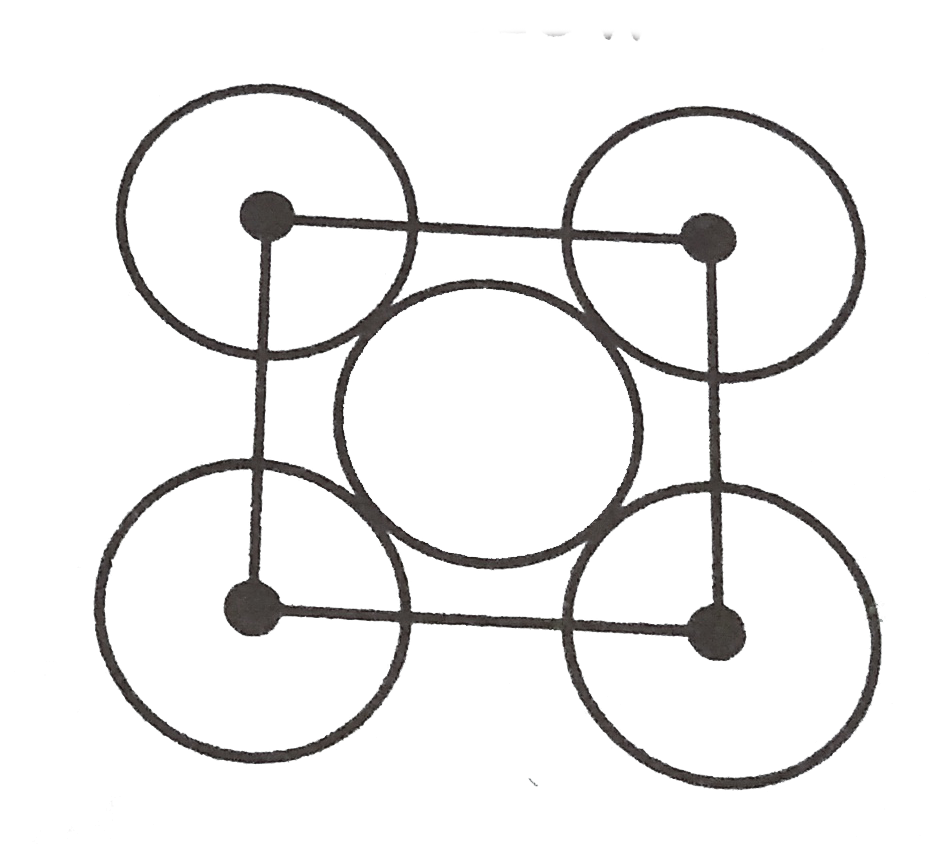
Calculate the number of particles per unit cell of the following two-dimensional lattices.

Text Solution
(a) Here, the unit cell is square. Each corner atom is shared with 4 adjoining unit cells. Therefore, number of corner-atoms present per unit cell = 4×14=1
Here, one atom is present in body-centre of each unit cell. Therefore, total number of atoms present per unit cell = 1 + 1 = 2.
(b) Here, the unit cell is two-dimensional hexagonal. In this type of unit cell, each corner-atoms is shared by 3 adjacent unit cells. Again, one atom is also present in the centre of the unit cell. Therefore, total number of atoms present per unit cell = 6×13+1=3.
|
Topper's Solved these Questions
- SOLID STATEBOOK - CHHAYA PUBLICATIONCHAPTER - SOLID STATEEXERCISE - VERY SHORT ANSWER TYPE
60 Videos
- SOLID STATEBOOK - CHHAYA PUBLICATIONCHAPTER - SOLID STATEEXERCISE - SOLVED WBCHSE SCANNER
8 Videos
- SOLID STATEBOOK - CHHAYA PUBLICATIONCHAPTER - SOLID STATEEXERCISE - PRACTICE SET 1
13 Videos
- PREVIOUS YEARS QUESTION PAPER 2019BOOK - CHHAYA PUBLICATIONCHAPTER - PREVIOUS YEARS QUESTION PAPER 2019EXERCISE - WBCHSE 2019 PART-B
19 Videos
- SOME p-BLOCK ELEMENTSBOOK - CHHAYA PUBLICATIONCHAPTER - SOME p-BLOCK ELEMENTSEXERCISE - PRACTIVE SET 11
15 Videos
Similar Questions
Knowledge Check
The number of atoms per unit cell in bcc lattice is
A1B2C4D9The effective number of lattice points per unit cell
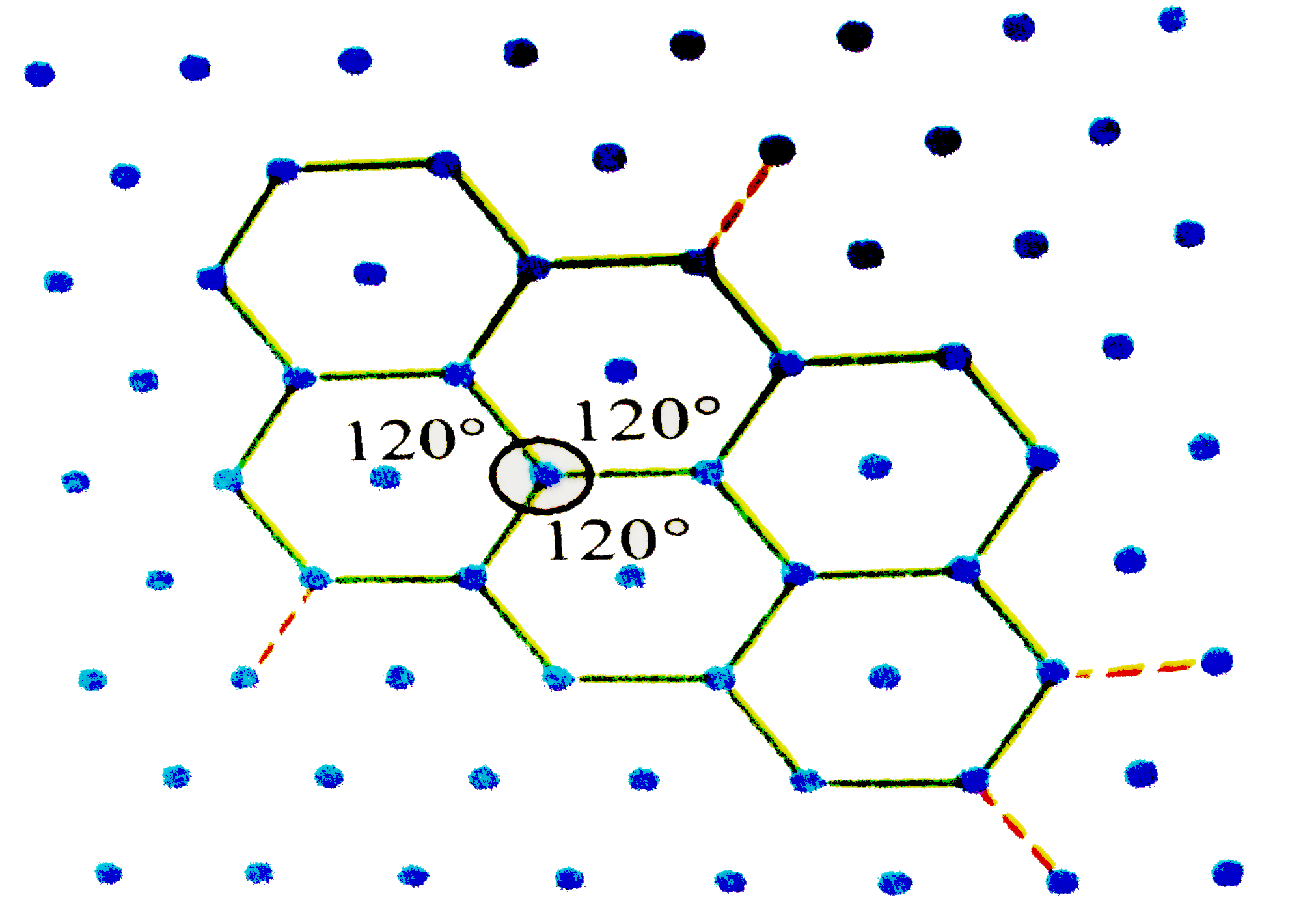 A1B2C3D4
A1B2C3D4In CsCl the number of formula units per unit cell is
A2B4C6D12
Unit cells can be divided into two categories, primitive and centred unit cells.
(a) Differentiate between unit cell and crystal lattice.
(b) Calcualte the number of atoms per unit cell in the following:
(i) body centred cubic unit cell (bcc)
(ii) face centred cubic unit cell (fcc) .
View SolutionCalculate the number of tetrahedral voids in the unit cell of a face-centred cubic lattice of similar atoms.
View SolutionCalculate the number of atoms per unit cell present in simple, fcc and bcc unit cells.
View SolutionThe number of carbon atoms per unit cell of diamond unit cell is
View SolutionThe number of carbon atoms per unit cell of diamond unit cell is
View Solution