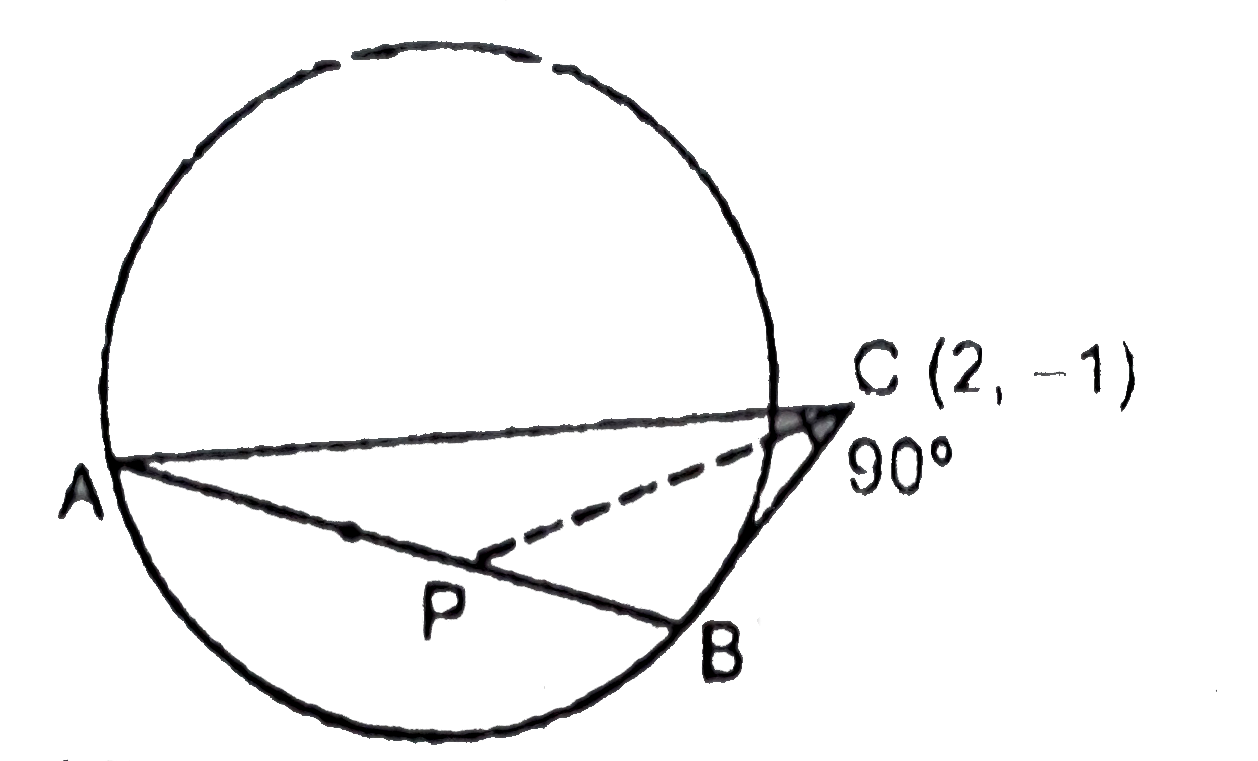
The locus of the midpoint of a chord of the circle $x^{2} + y^{2} = 4$ which subtends a right angle at the origins is $x + y = 2$ (b) $x^{2} + y^{2} = 1$ $x^{2} + y^{2} = 2$ (d) $x + y = 1$

$x^{2} + y^{2} - 2 x + y - 2 = 0$

$x^{2} + y^{2} - 2 x - y - 2 = 0$

$x^{2} + y^{2} - 2 x + y + 1 = 0$

$x^{2} + y^{2} - 2 x + y + 2 = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Updated on:21/07/2023

The locus of the midpoint of chord of the circle $x^{2} + y^{2} = 1$ which subtends a right angle at the origin is
A $x^{2} + y^{2} = \frac{1}{4}$
B $x^{2} + y^{2} = \frac{1}{2}$
C $x y = 0$
D $x^{2} - y^{2} = 0$
The locus of the mid -point of the chords of a circle $x^{2} + y^{2} = 4$ ,which subtends a right angle at the centre , is
A $x + y = 2$
B $x^{2} + y^{2} = 1$
C $x^{2} + y^{2} = 2$
D $x - y = 0$