Inside a solid glass sphere of radius R, a point source of light lies at a distance x(x<R) from centre of the sphere. The solid is surrounded by air of refractive index 1. The maximum angle of incidence for rays incident on the spherical glass air interface directly from the point soure is:
cos−1xR
sin−1xR
cos−1√xR
sin−1√xR
The correct Answer is:B
Let O be the centre of A be the point source for ray incident
at any point P t angle have maxmum angle of incidence i, 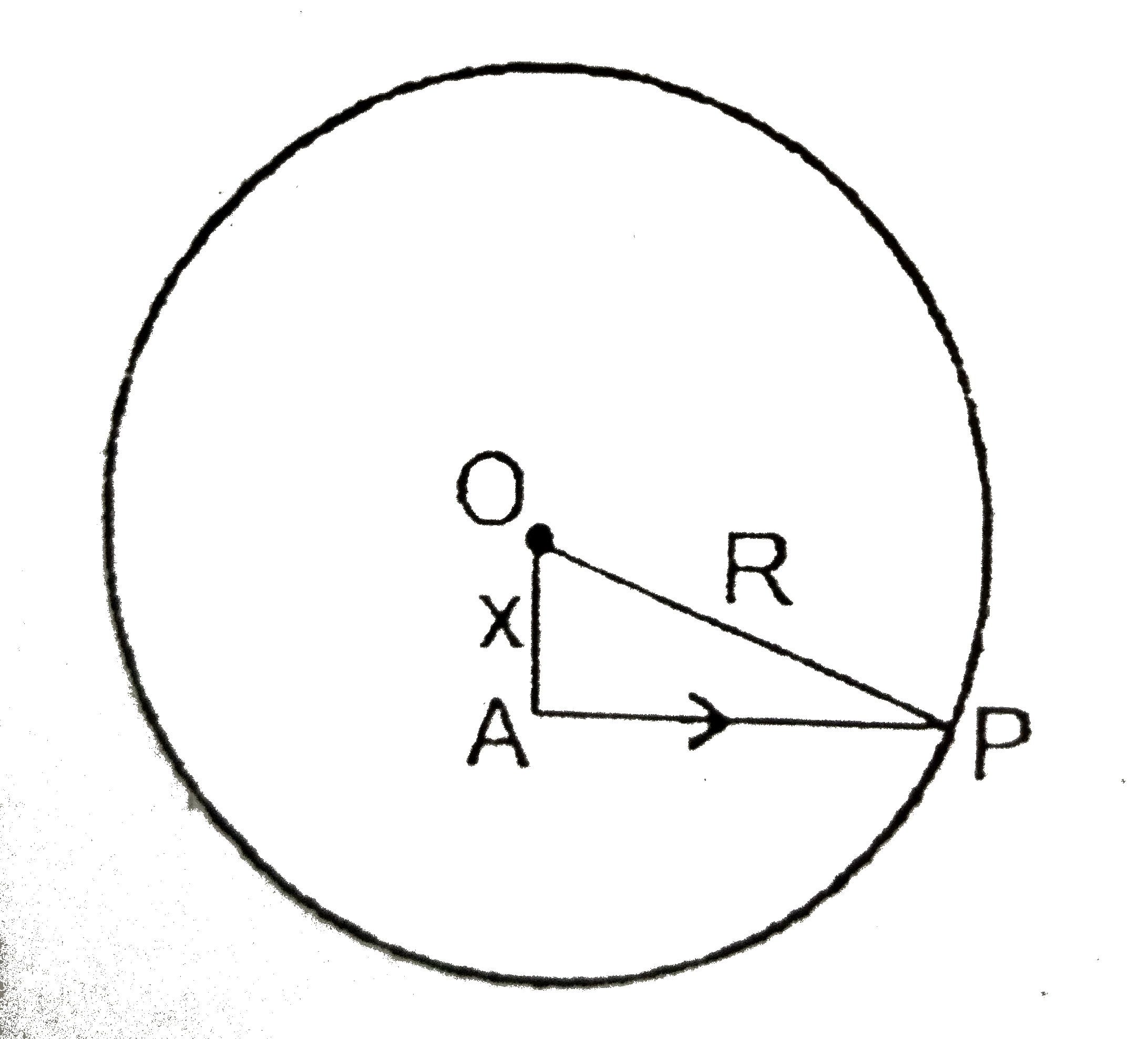
Similar Questions
Knowledge Check
- Question 1 - Select One
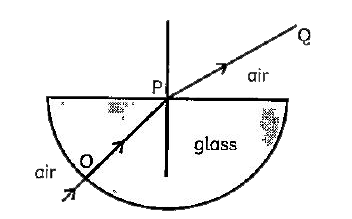
The angle of incidence from air to glass at the point O on the hemispherical glass slab is.A45∘B0∘C90∘D180∘ - Question 2 - Select One
A ray of light is incident on a glass sphere of refractive index 3/2. What should be the angle of incidence so that the ray which enters the sphere does not come out of the sphere ?
Atan−1(2/3)Bsin−1(2/3)C90∘Dcos−1(1/3) - Question 3 - Select One
A ray of light is incident on a glass sphere of refractive index 3/2 . What should be the angle of incidence so that the ray which enters the sphere does not comme out of the sphere?
Atan−1(2/3)B60∘C90∘D30∘
A light source S is placed at the centre of a glass sphere of radius R and refractive index μ. The maximum angle θ with the x-axis (as shown in the figure) an incident light ray can make without suffering total internal reflection is
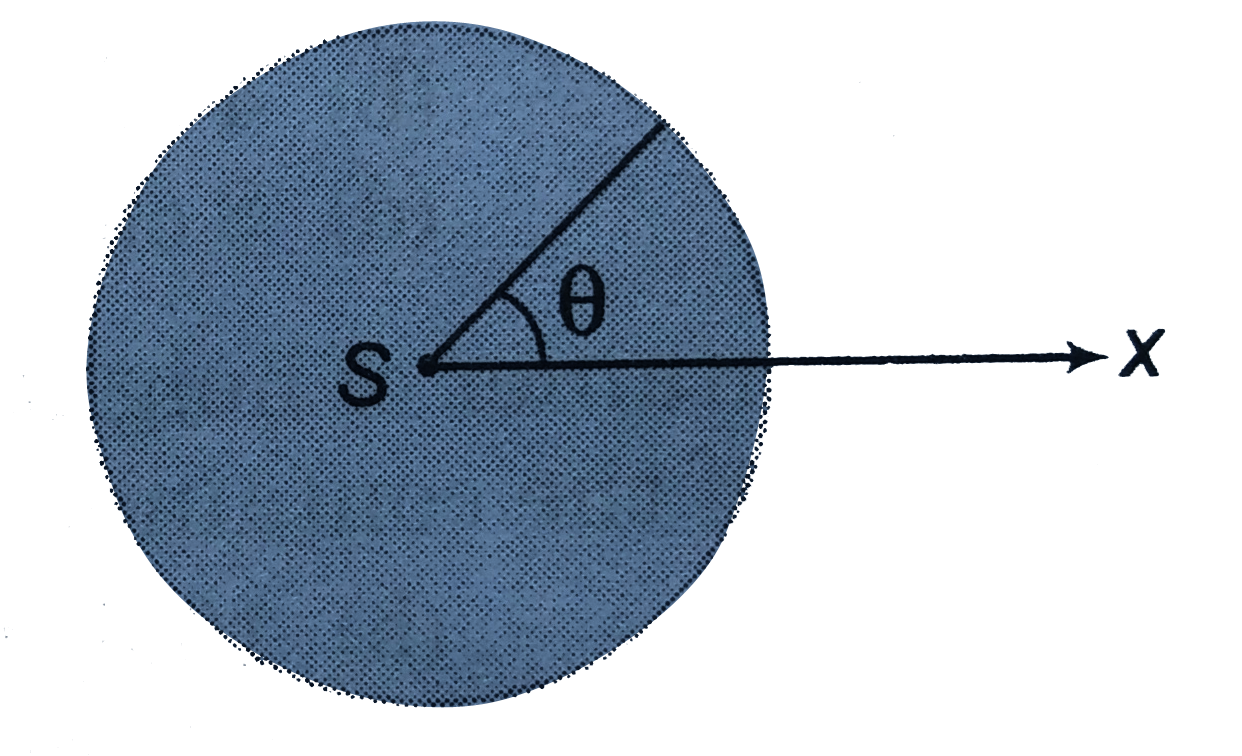 View Solution
View SolutionA ray of light passes from glass having a refractive index of 1.6, to air. The angle of incidence for which the angle of refraction is twice the angle of incidence is
View SolutionA ray of light passes from glass, having a refractive indx of 1.6, to air. The angle of incidence for which the angle of refraction is twice the angle of incidence is
View SolutionA point object is placed at the centre of a glass sphere of radius 6 cm and refractive index 1.5. The distance of the virtual image from the surface of the glass is
View SolutionA ray of light is incident on the surface of plate of glass of refractive index 1.5 at the polarising angle. The angle of refraction of the ray will be
View Solution


