The angles of elevation of the top of a lighthouse from 3 boats A,B and C in a straight line of same side of the lighthouse are a,2a,3a respectively. If the distance between the boats A and B is x meters. Find the height of lighthouse.Solution in Telugu
The correct Answer is:xtanatan2atan2a−tana
Step by step video, text & image solution for The angles of elevation of the top of a lighthouse from 3 boats A,B and C in a straight line of same side of the lighthouse are a,2a,3a respectively. If the distance between the boats A and B is x meters. Find the height of lighthouse. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - APPLICATIONS OF TRIGONOMETRYEXERCISE - OBSERVATION MATERIAL21videos
APPLICATIONS OF TRIGONOMETRY
- BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - APPLICATIONS OF TRIGONOMETRYEXERCISE - CREATIVE QUEATIONS6videos
APPLICATIONS OF TRIGONOMETRY
- BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - APPLICATIONS OF TRIGONOMETRYEXERCISE - EXERCISE 12.29videos
APPLICATIONS OF TRIGONOMETRY
- BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - APPENDIX MATHEMATICAL MODELLINGEXERCISE - THINK & DISCUSS1videos
APPENDIX MATHEMATICAL MODELLING
- BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - COORDINATE GEOMETRY EXERCISE - CCE model examination151videos
COORDINATE GEOMETRY
Similar Questions
A boy observed the top of an electric pole at an angle of elevation of 30∘, when the observation point is 10 meters away from the foot of the pole. Draw suitable diagram for the above sitution.
03:15View SolutionA pole is arranged from a height of 30m from the ground, making 60∘ angle with earth. Then what is its length?
04:41View SolutionA TV tower stands vertically on the side of a road. From a point on the other side directly opposite to the tower, the angle of elevation of the top of tower is 60∘. From another point 10 m away from this point, on the line joining this point to the foot of the tower, the angle of elevation of the top of the tower is 30∘. Find the height of the tower and the width of the road.
02:02View SolutionDiscuss with your friends some more examples in real life where we cannot directly measure and must use mathematical modelling.
02:36View SolutionFind the distance between the points (5 , 7) and (7 , 5) by plotting them in co-ordinate plane with the help of a right angled triangle .
03:45View SolutionFrom the top of a lighthouse 120m above the sea, the angle of depression of a boat is 15∘. What is the distance of the boat from the lighthouse?
05:20View SolutionFrom the top of a lighthouse 70 m high with its base at sea level, the angle of depression of a boat is 15∘. The distance of the boat from the foot of the lighthouse is :
04:09View SolutionFrom the top of a lighthouse, 100 m high, the angle of depression of a boat is tan−1(512). What is the distance between the boat and the lighthouse?
13:21View SolutionFrom the top of a lighthouse, an observer looking at a boat makes an angle of depression of 60∘. If the height of the lighthouse is 90m, then find how far is the boat from the lighthouse.
(√3=1.73)03:06View Solution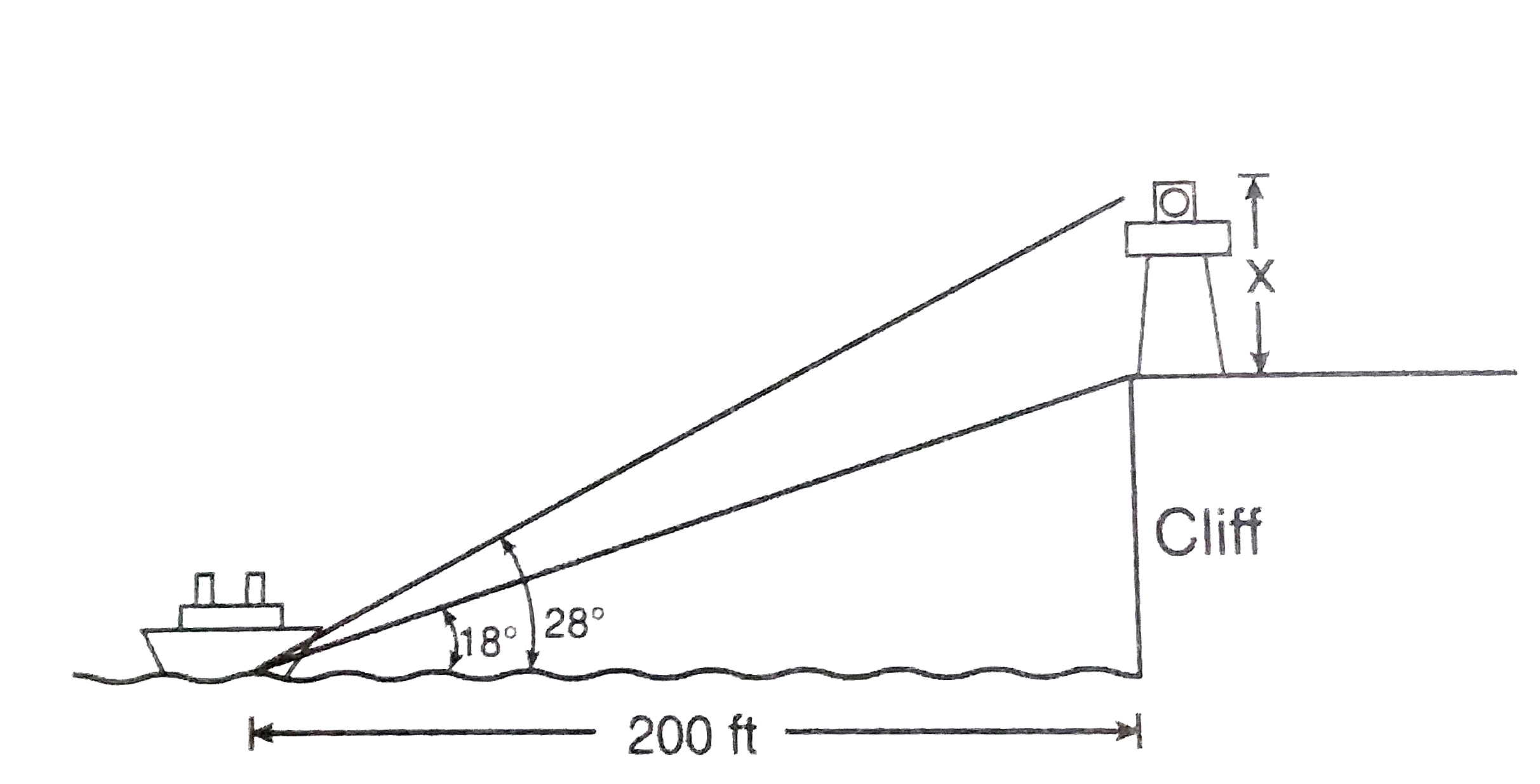
A lighthouse is built on the edge of a cliff near the ocean, as shown in the diagram above. From a boat located 200 feet from the base of the cliff, the angle of elevation to the top of the cliff is 18∘ and the angle of elevation to the top of the lighthouse is 28∘. Which of the following equations could be used to find the height of the lighthouse, x, in feet?04:40View SolutionTwo ships are sailling in the sea on the two sides of a lighthouse. If the distance between the ships is 10(√3+1) meters and their angle of elevations of the top of the lighthouse are 60∘and45∘, then the height of the lighthouse is (The two ships and the foot of lighthouse are in a straight line)
02:23View SolutionFrom the top of a lighthouse 60 meters high with its base at the sea level, the angle of depression of a boat is 15^(@) . The distance of the boat from the foot of the lighthouse is
03:57View SolutionThe three boats A, B and C were traveling in a straight line coming towards the lighthouse. The upper bouts featured two cutaways, for easier access to the higher frets. What is the height of the lighthouse x the distance between boats A and B?
06:39View SolutionThe angles of elevation oand depression of the top and bottom of a lighthouse from the top of a building, 60 m high, are 30∘ and 60∘ respectively. Find
(i) the difference between the heights of the lighthouse and the building.
(ii) distance between the lighthouse and the building.View SolutionThe angles of elevation of the top of a lighthouse from 3 boats A, B and C in a straight line of same side ofthe light house are a, 2a, 3a respectively. If the distance between the boats A and B and the boats B and Care x and y respectively find the height of the light house?
02:05View Solution

