Question

A small ball of mass $2\times {10}^{-3}$ kg having a charge of $1\mu C$ is suspended by a string of length 0.8 m. Another identical ball having the same charge is kept at the point of suspension. Determine the miniumum horizontal velocity which should be imparted to the lower ball, so that it can make complete revolution.

Explanation

Let charge of each ball =q, the radius of the vertices circular path =l and the velocity of the rotating ball at the highest and lowest point of that circular path be v and u respectively.
At the highest point A, three forces act simultaneously which are (1) upward electrostatic force of repulsion, `F_(e) =q^(2)/l^(2)`. (2) weight of the rotating ball, W =mg (down-wards), (3) tension in the string =T (downwards).

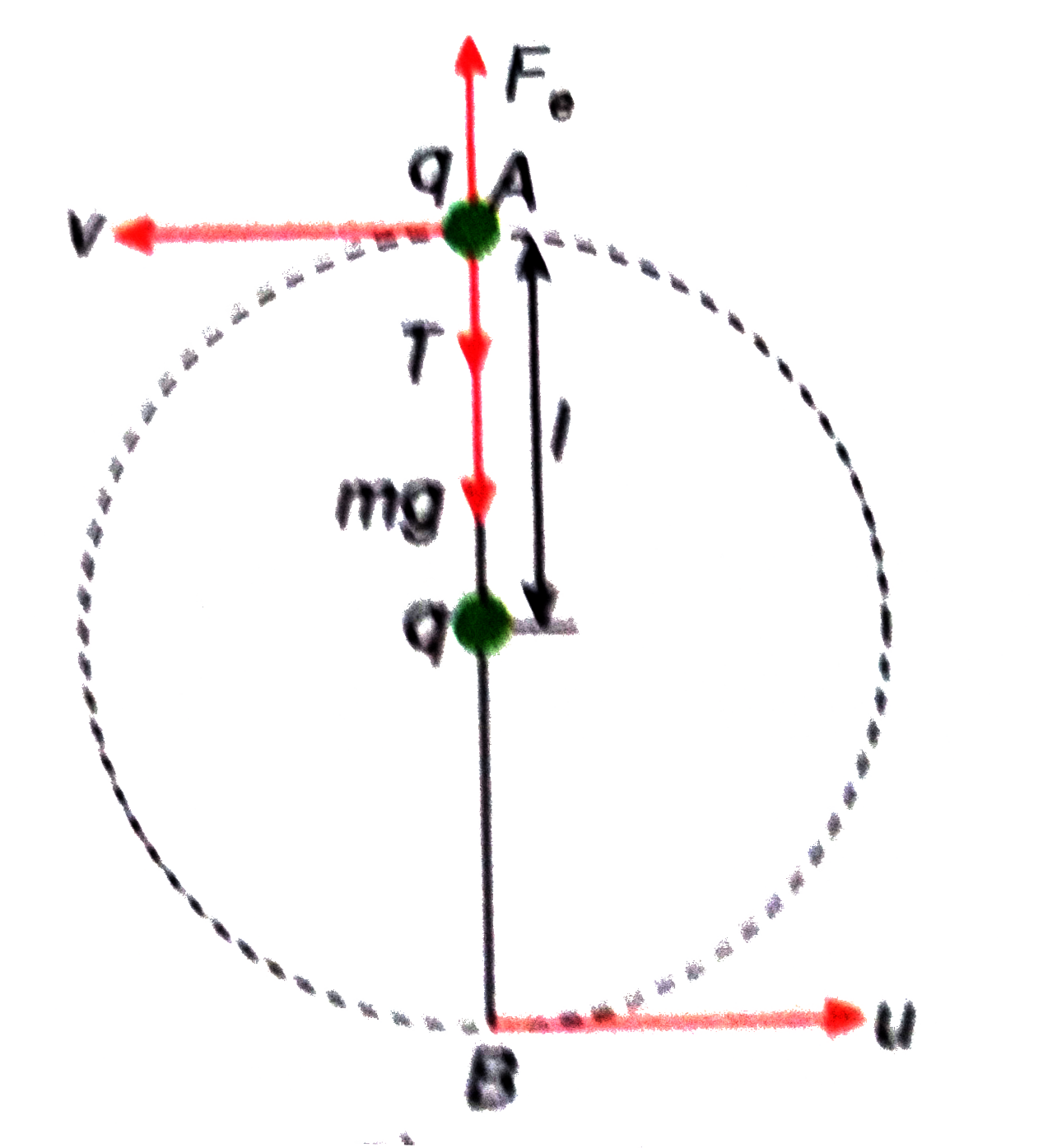
To move in a vertical circular path, the ball should have a minimum velocity at A. In that case, T=0 and the necessary centripetal force is provided by `(W-F_(e))`.
`therefore W-F_(e) =(mv^(2))/l`
`therefore v^(2) = l/m[mg - q^(2)/l^(2)]`
Applying the law of conservation of total mechanical energy at A and B we can write,
`1/2 mv^(2) + mg xx 2l = 1/2 mu^(2)`
or `u^(2) =v^(2) =4gl =l/m[mg-q^(2)/l^(2)] + 4gl =5gl -q^(2)/(ml)`
`therefore u=sqrt(5gl-q^(2)/(ml)]`
Hence, minimum horizontal velocity at the lowest point is `sqrt(5gl -q^(2)/(ml))`.