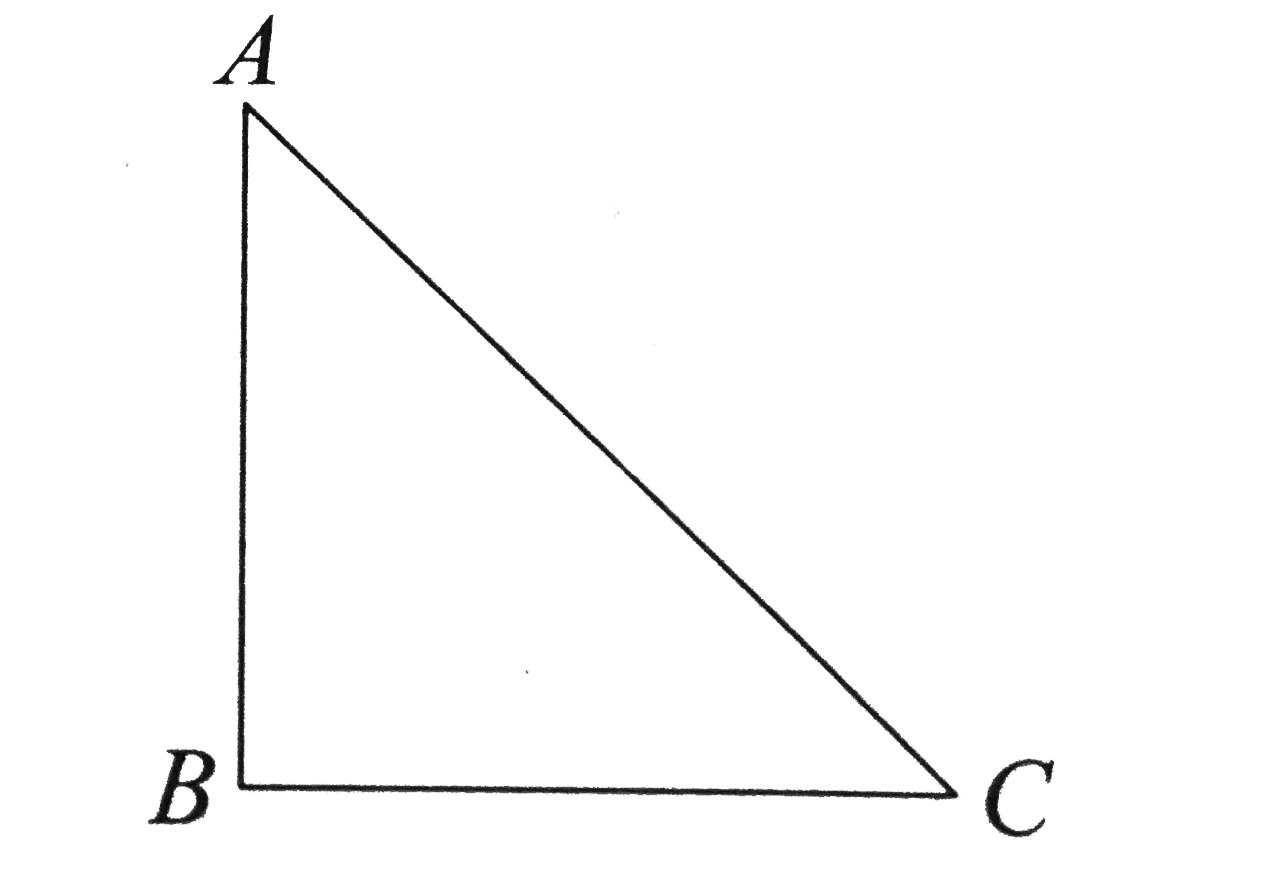
A rigid insulated wire frame in the form of a right angled triangle ABC is set in a vertical plane as shown in the figure. Two beads, each of mass m and carrying charges q1 and q2 are connected by a cord of length l and kept between the arms AB and AC of the triangle. In such a way that they can slide without friction along the arms. When the beads are stationary, determine- (i) the angle α, (ii) the tension in the cord and (iii) the normal reaction on the beads.
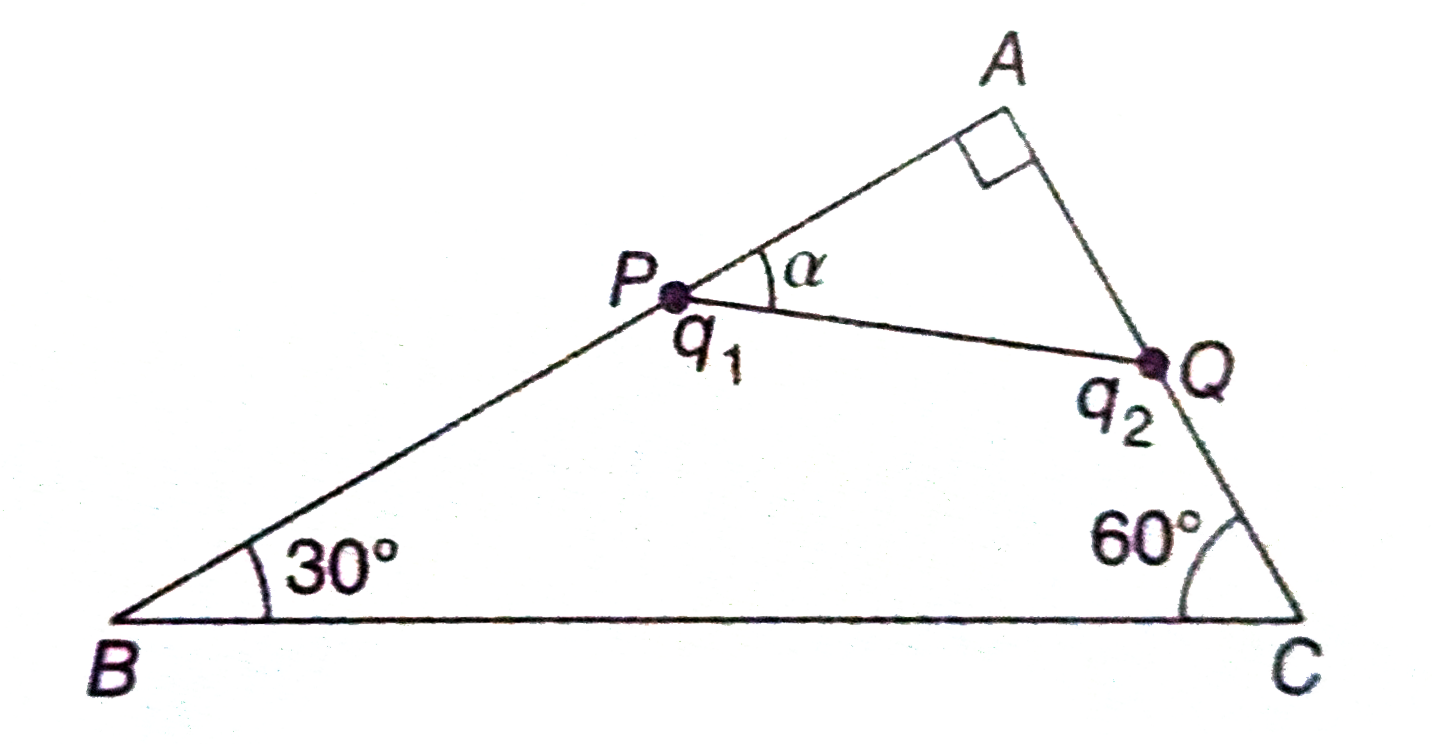
If the cord is now cuts what are the values of charge for which the beads continue to remain stationary?
Let us consider the force acting on bead P as shown in the figure. The forces are (1) weight mg acting vertically downward. (2) tension T in the cord, (3) electric force between P and Q given by, F=14πε0.q1q2l2, (4) normal reaction NR1
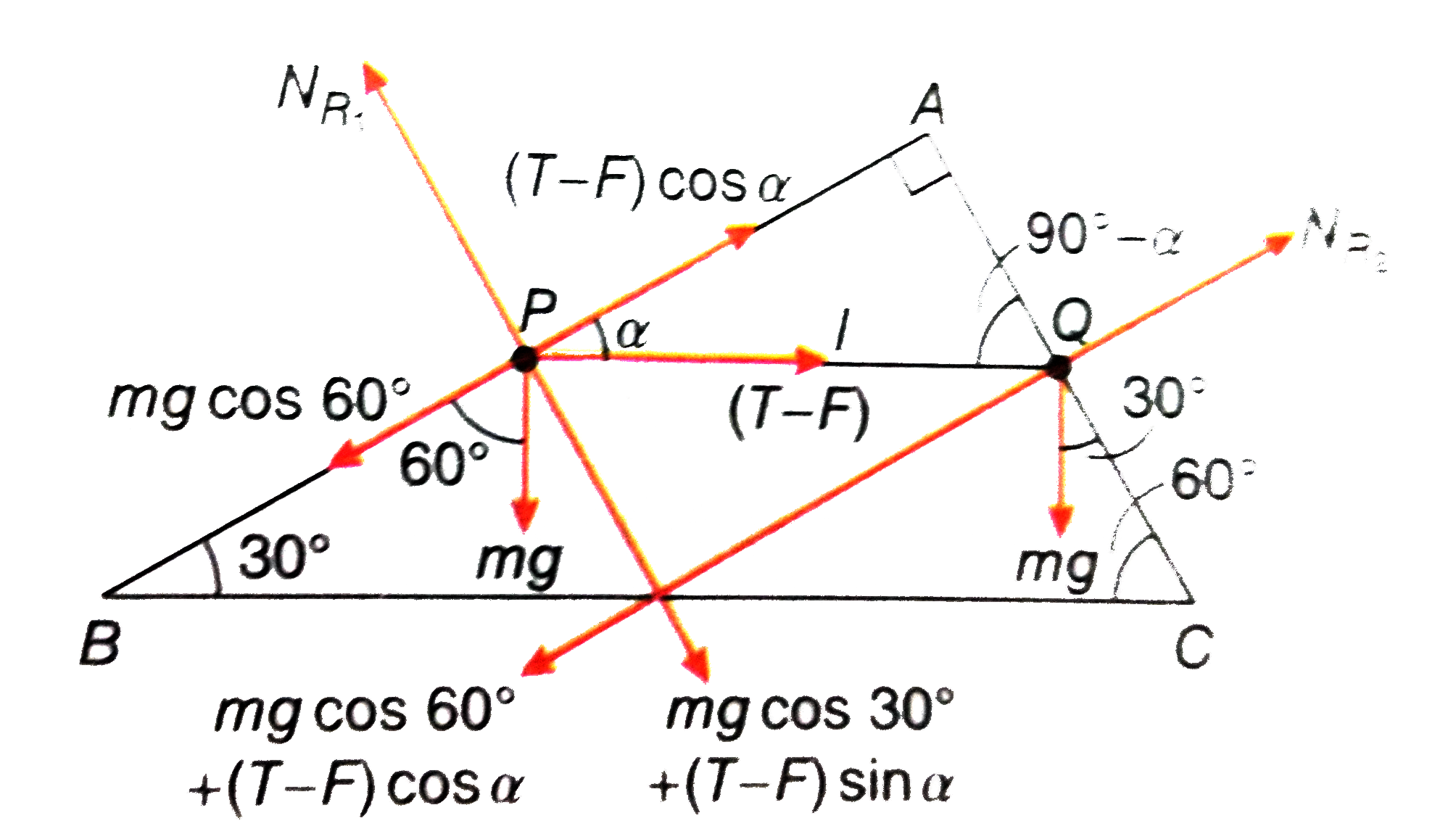
When the bead P is in at equilibrium,
mgcos60∘=(T−F)cosα
and NR1=mgcos30∘+(T−F)sinα....(2)
For the bead Q, we have
mgsin60∘=(T−F)sinα.......(3)
and N2=mgcos60∘+(T−F)cosα........(4)
(i) Divide (3) by (1),
tanα=tan60∘∴α=60∘
(ii) Again putting α=60∘, in equation (3),
mgsin60∘=(T−F)sin60∘
or T=F+mg=14πε0.q1q2l2+mg.......(5)
(iii) From equation (4), T-F= mg
∴NR2=mgcos60∘+mgcos60∘=mg
From equation (2),
NR1=mgcos30∘+mgsin60∘=√3mg
When the cord is cut, T=0
From equation (5), we get
mg=14πε0.q1q2l2
The right hand side of the equation should be positive which is possible only is q1 and q2 have opposite signs. Thus for equilibrium, the beads must have unlike charges. The magnitude of the product of the charges is [q1q2]=4πε0mgl2.
Topper's Solved these Questions
- ELECTRIC FIELDBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - SECTION RELATION QUESTIONS
50 Videos
- ELECTRIC FIELDBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - HIGHER ORDER THINKING SKILL QUESTIONS
33 Videos
- ELECTRIC ENERGY AND POWERBOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC ENERGY AND POWEREXERCISE - CBSE SCANNER
7 Videos
- ELECTRIC POTENTIAL BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC POTENTIAL EXERCISE - CBSE Scanner
13 Videos
Similar Questions
A rigid insulated wire frame in the form of a right-angled triangle ABC is set in a vertical plane as shown in fig. two beads of equal masses m each and carrying charges q1 and q2 are connected by a cord of length l and can slide without friction on the wires.
Cinsidering the case when the beads are stationary, determine
(i) the angel α
(ii) the tension in the cord, and
(iii) The normal recation on teh beads.
If the cord is now cut, what are the value of the charges for which the beads continue to remain stationary?
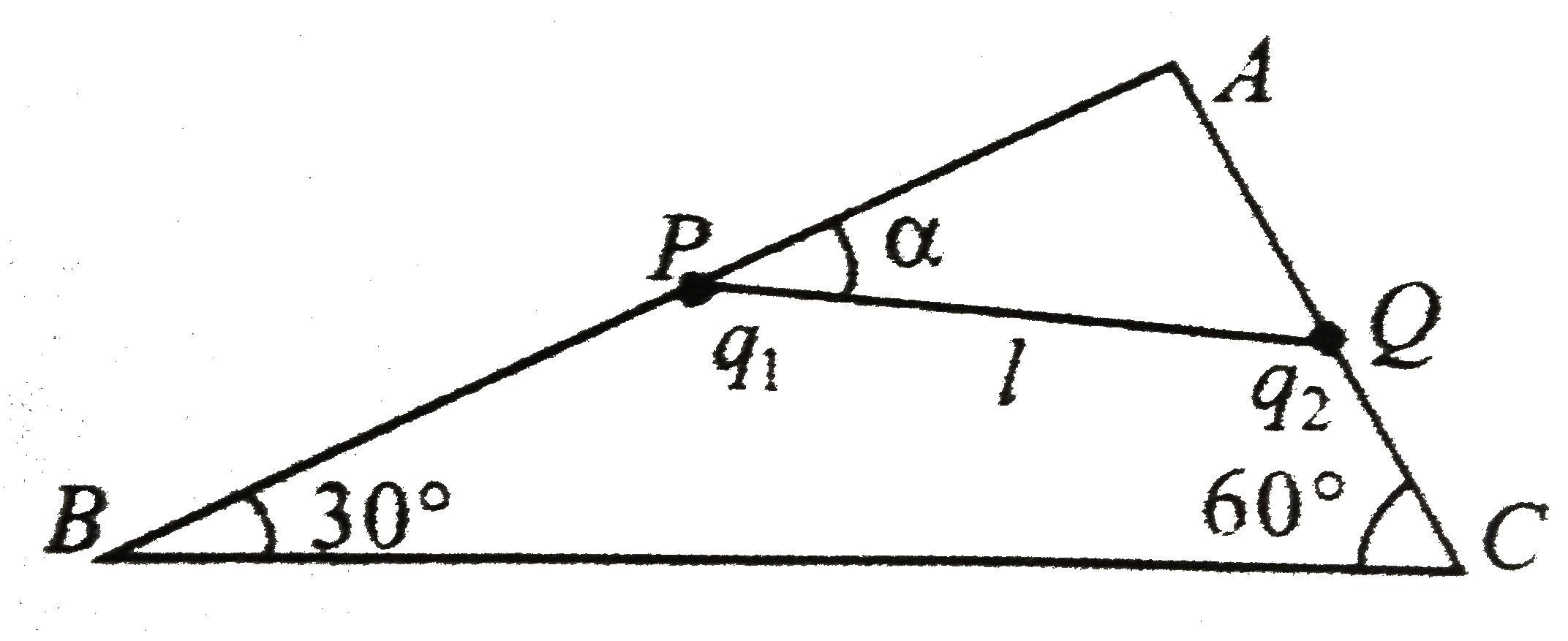
View SolutionA rigeid insulated wire frame in the form of a right-angled traingle ABC is set in a vertical plane as shown in fig. two beads of equal masses m each and carrying charges q1 and q2 are connected by a cord of length l and can slide without friction on the wires.
Cinsidering the case when the beads are stationary, determine
(i) the angel α
(ii) the tension in the cord, and
(iii) The normal recation on teh beads.
If the cord is now cut, what are the value of the charges for which the beads continue to remain stationary?

View Solution
Knowledge Check
A current carrying closed loop in the form of a right angle isosceles triangle ABC is placed in a uniform magnetic field acting along AB. If the magnetic force on the arm BC is →F, the force on the arm AC is
 A−√2→FB−→FC→FD√2→F
A−√2→FB−→FC→FD√2→FA current carrying closed loop in the from of a right angle isoseles triangle ABC is placed in a unifrom magnetic fild acting along AB. If the magnetic force on the arm BC is F, the force on the arm AC is
 A−FBFC√2FD−√2F
A−FBFC√2FD−√2F
1. In a right angled triangle ABC, AB = AC. Thena:b:c is
View Solution1. In a right angled triangle ABC, AB = AC. Thena:b:c is
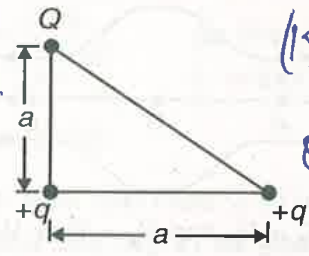
View SolutionThree charges +q , +q, and Q are placed at the comers of a right angled isosceles triangle as shown in the figure. If the net potential energy of the system is zero then value of Q is
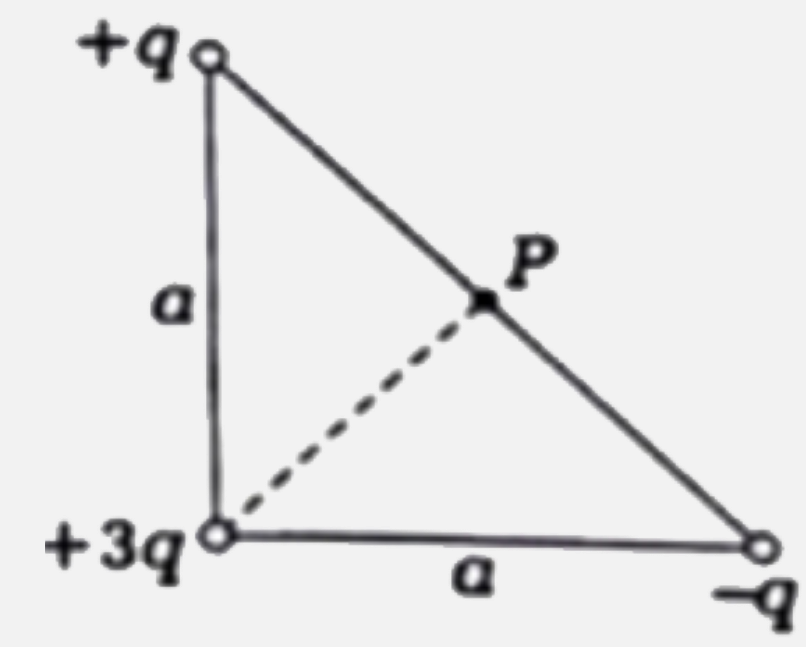
View SolutionA right isosceles triangle of side a has changes q, 3q and - q arranged on its verticals as shown in the figure. What is the electric potential at P midway between the line connecting the + q and – q charges ?
View SolutionA current carrying closed loop in the from of a right angle isoseles triangle ABC is placed in a unifrom magnetic fild acting along AB. If the magnetic force on the arm BC is F, the force on the arm AC is

View SolutionA right angled triangular shaped frame ABC rests in xy plane as shown in figure. It starts sliding overx-axis keeping AandB on axes. If AB=AC=10, then locus of circumcentre of ΔABC is
View Solution
