A ball is launched from the top of Mt. Everest which is at elevation of 9000 m. The ball moves in circular orbit around earth. Acceleration due to gravity near the earth's surface is g. The magnitude of the ball's acceleration while in orbit is -
close to g/2
Zero
much greater than g
nearly equal to g
The correct Answer is:D
Step by step video, text & image solution for A ball is launched from the top of Mt. Everest which is at elevation of 9000 m. The ball moves in circular orbit around earth. Acceleration due to gravity near the earth's surface is g. The magnitude of the ball's acceleration while in orbit is - by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
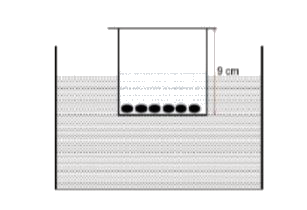
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
 01:47View Solution
01:47View SolutionAn astronaut orbiting the earth in a circular orbit 120km above the surface of earth, gently drops a ball from the space-ship. The ball will
03:07View SolutionA spaceship is launched in to a circular orbit of radius R close to surface of earth. The additional velocity to be imparted to the spaceship in the orbit to overcome the earth's gravitational pull is (g=acceleration due to gravity)
01:57View SolutionAn earth satellite of mass m revolves in a circular orbit at a height h from the surface of the earth. R is the radius of the earth and g is acceleration due to gravity at the surface of the earth. The velocity of the satellite in the orbit is given by
02:36View SolutionA satellite is in a circular orbit round the earth at an altitude R above the earth's surface, where R is the radius of the earth. If g is the acceleration due to gravity on the surface of the earth, the speed of the satellite is
05:03View SolutionAn artificial satellite is moving in a circular orbit around the earth with a speed equal to half the magnitude of escape velocity from the surface of earth. R is the radius of earth and g is acceleration due to gravity at the surface of earth. (R=6400 km). The time period of revolution of satellite in the given orbit is
02:01View SolutionAn artificial satellite is moving in circular orbit around the earth with speed equal to half the magnitude of escape velocity from the surface of earth. R is the radius of earth and g is acceleration due to gravity at the surface of earth (R=6400km)
Then the distance of satelite from the surface of earth is .02:58View SolutionA satellite is revolving around the earth in a circular orbit at an altitude h where the acceleration due to gravity is g'. If the earth is a sphere of radius R then the period of the satellite is give by
03:04View SolutionAs we move far from the earth's surface the magnitude of acceleration due to gravity ......
02:18View SolutionA satellite is revolving in a circular orbit of round the earth at a height of h. If the acceleration due to gravity at that height is g. The orbital speed of the satellite .
View SolutionA spaceship is launched into a circular orbit of radius R close to the surface of the earth. The additional velocity to be imparted to the spaceship in the orbit to overcome the earth's gravitational pull is (g→ acceleration due to gravity)
View SolutionA spaceship is launched into a circular orbit of radius R close to the surface of the earth. The additional velocity to be imparted to the spaceship in the orbit to overcome the earth's gravitational pull is (g→ acceleration due to gravity)
09:19View SolutionAn artificial satellite is moving in a circular orbit around the Earth at a speed equal to half the magnitude of the migration velocity from the surface of the Earth. The acceleration due to gravity on the Earth's radius R and the surface of the Earth (R = 6400km) is g Periodicity of satellite revolving in a given orbit
02:49View SolutionIf the earth were to spin faster, acceleration due to gravity at the poles :
03:24View SolutionAn artificial satellite is moving in circular orbit around the earth with speed equal to half the magnitude of escape velocity from the surface of earth. R is the radius of earth and g is acceleration due to gravity at the surface of earth (R=6400km)
Then the distance of satelite from the surface of earth is .04:06View Solution

