A ball of radius r hits a cushion with a pure rolling motion and rebounds, with a pure rolling motion. Find the ratio of the height h of the cushion to the radius r of the ball. Assume that the foce exerted on the ball by the cushion is horizontal during the impact and that the ball hits the cushion normally.
Step by step video & image solution for A ball of radius r hits a cushion with a pure rolling motion and rebounds, with a pure rolling motion. Find the ratio of the height h of the cushion to the radius r of the ball. Assume that the foce exerted on the ball by the cushion is horizontal during the impact and that the ball hits the cushion normally. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ANURAG MISHRACHAPTER - RIGID BODY MOTIONEXERCISE - SCQ_TYPE83videos
RIGID BODY MOTION
- BOOK - ANURAG MISHRACHAPTER - RIGID BODY MOTIONEXERCISE - MCQ_TYPE41videos
RIGID BODY MOTION
- BOOK - ANURAG MISHRACHAPTER - IMPULSE AND MOMENTUMEXERCISE - matching3videos
IMPULSE AND MOMENTUM
- BOOK - ANURAG MISHRACHAPTER - UNIT AND DIMENSIONSEXERCISE - Matching Type Problem4videos
UNIT AND DIMENSIONS
Similar Questions
A ball of radius r is made to oscillate in a bowl of radius R, find its time period of oscillation.
View SolutionA ball weighing 50g hits a hard surface normally with a speed of 25m/s and rebounds with the same speed. The ball remains in contact with the surface for 0.05s .The average force exerted by the surface on the ball is?
View Solution
Knowledge Check
- Question 1 - Select One
A : The work done by friction on an object during pure rolling motion is zero.
R : In pure rolling motion, there is relative motion at the point of contact.AIf both Assertion & Reason are true and the reason is the correct explanation of the assertion,BIf both Assertion & Reason are true but the reason is not the correct explanation of the assertion,CIf Assertion is true statement but Reason is false,DIf both Assertion and Reason are false statements, - Question 2 - Select One
Where must the cue hit a billiard ball so that it rolls without sliding form the start if R is the radius of the ball?
AAt a height 25 R above centre.BAt a height equal to the radius from tableCAt a height equal to 2R from the tableDAt a height equal to R2 from table. - Question 3 - Select One
A ball is thrown from a point in level with velocity u and at a horizontal distance r from the top of a tower of height h.
At what horizontal distance x from the foot of the tower does the ball hit the ground ?
Aucosθg{(u2sin2θ+gh)1/2−usinθ}Bucosθg{(u2cos2θ+2gh)1/2−ucosθ}Cucosθg{(u2cos2θ+gh)1/2−ucosθ}Ducosθg{(u2cos2θ+gh)1/2−ucosθ}
A ball of radius R is uniformly charged with the volume density ρ. Find the flux if the electric field strength vector across the balls section formed by the plane located at a distance r0<R from the centre of the ball.
View SolutionA batsman hits a cricket ball which then rolls on a level ground. After covering a short distance, the ball comes to rest. The ball slows to a stop because (a) the batsman did not hit the ball hard enough, (b) velocity is proportional to the force exerted on the ball, (c ) there is a force on the ball opposing the motion ,(d) there is no unbalanced forcr on the ball, so the ball would want to come to rest.
View SolutionA spherical rigid ball is realeased from rest and starts rolling down an inclined plane from height h=7m, as shown in the figure. It hits a block at rest on the horizontal plane (assme elastic collision). If the mass of both the ball and the block is m and the ball is rolling without sliding, then the speed of the block after collision is close to
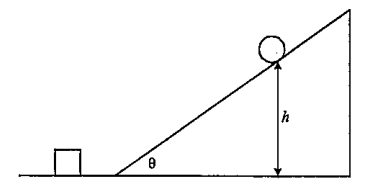View SolutionA ball falls freely form a height onto and smooth inclined plane forming an angle a with the horizontal. Find the ratio of the distance between the points at which the jumping ball strikes the inclined plane. Assume the impacts to be elastic.
View SolutionA small block of mass m slides along a smooth frictional track as shown in the figure. If it starts from rest at P, what is the resultant force acting on it at Q?
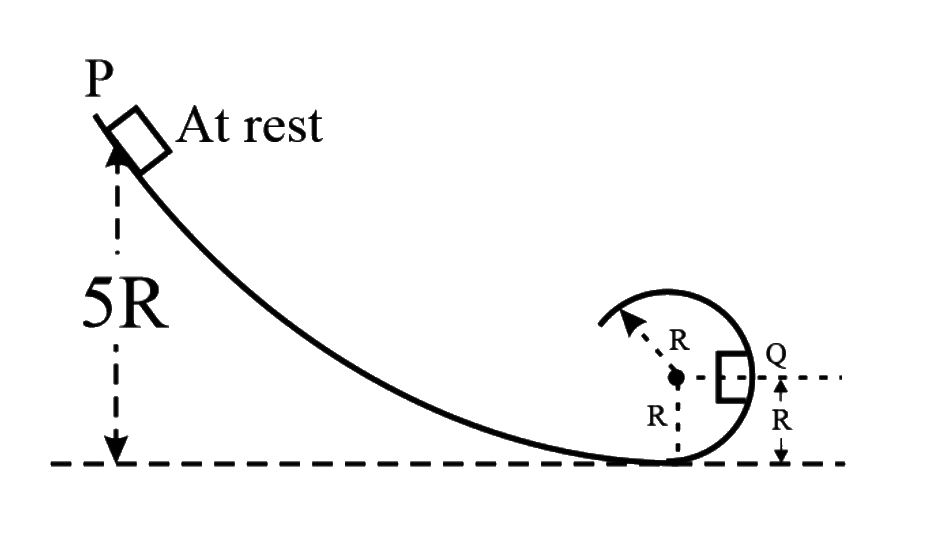View Solution