In a reactor, an element X decays to a radioactive element Y, at a constant rate r atoms per second. Each decay reaction releases energy E1. Half life of element Y is equal to T and decays to a stable element. During each decay of Y, energy E2 is released. If at t=0, there was no atom of element Y and all the energy released is used in the reactor for generation of electrical power with efficiency η, calculate electrical power generation in the reactor
(i) at time t and (ii) in steady state.
The correct Answer is:[(i) ηr[E1+E2(1−etlog2T)],(ii) ηr(E1+E2)]
Similar Questions
Knowledge Check
Rate of radiocative decay for a radioactive element depends on :
Aamount of radioactive elementBtemperatureCpressureDistopic from of radioactive elementN1 atoms of a radioactive element emit N2 beta partilces per second. The decay cosntant of the element is (in s−1)
AN1N2BN2N1CN1ln(2)DN2ln(2)The graph between number of decayed atoms N' of a radioactive element and time t is.
A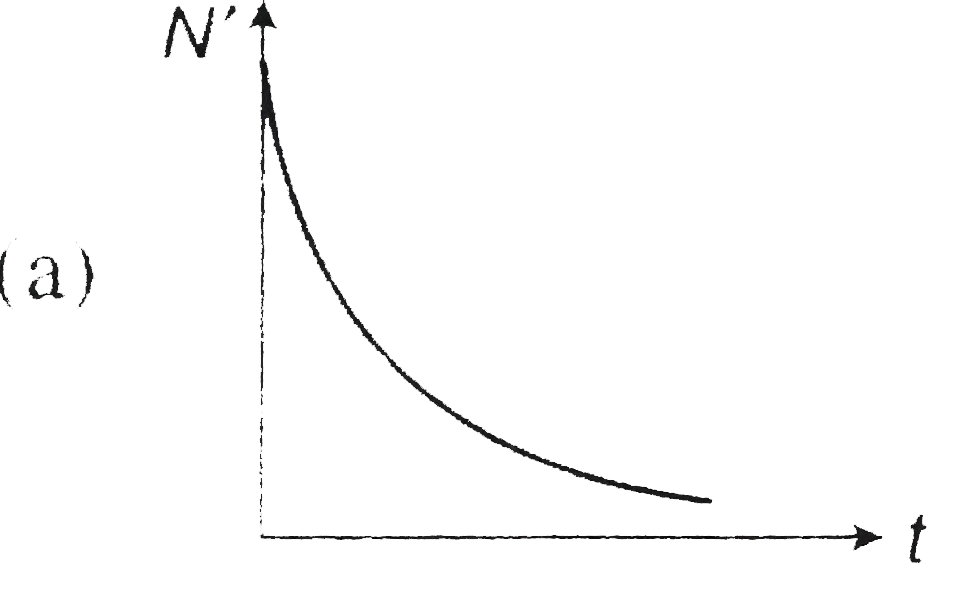 .B
.B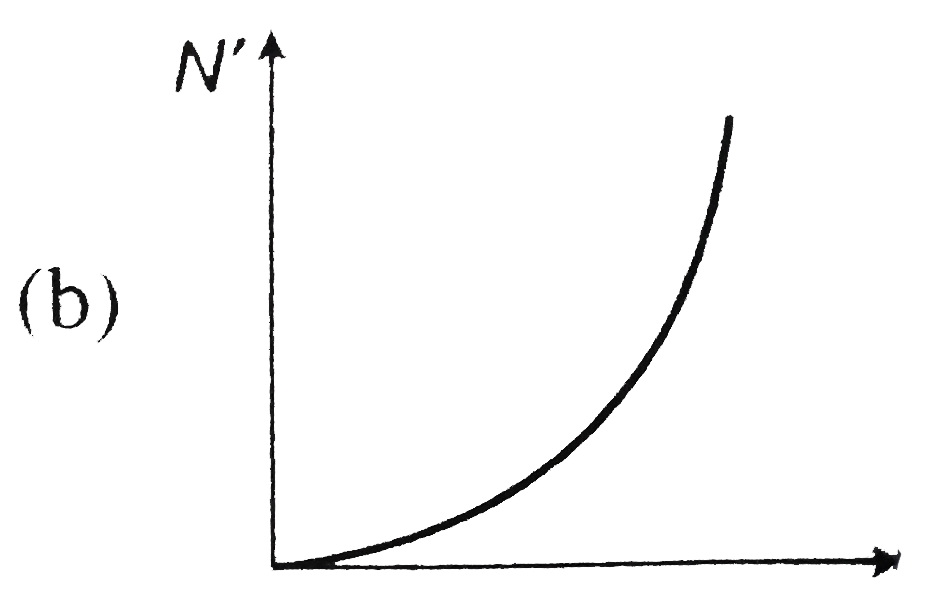 .C
.C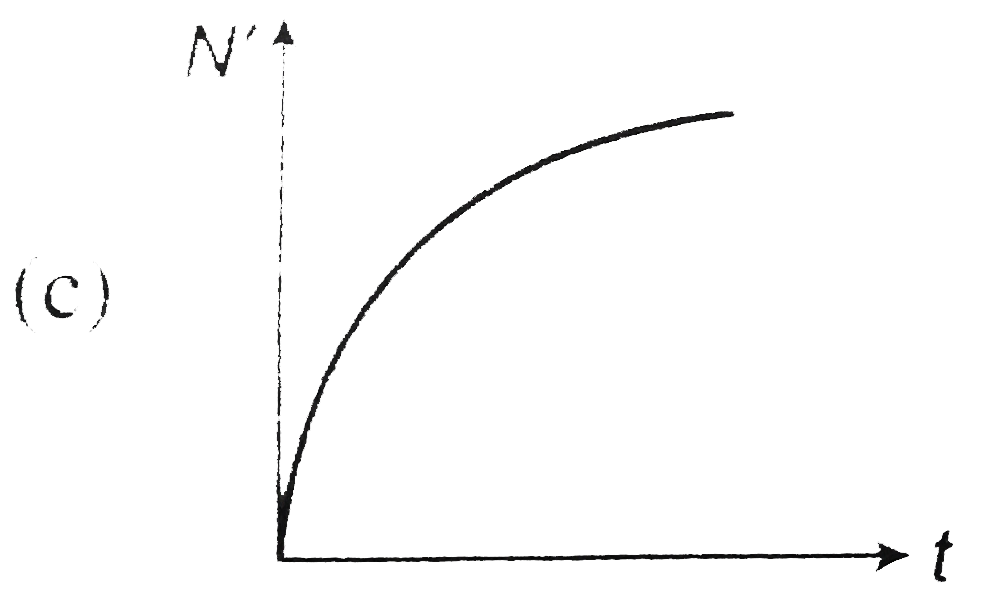 .D
.D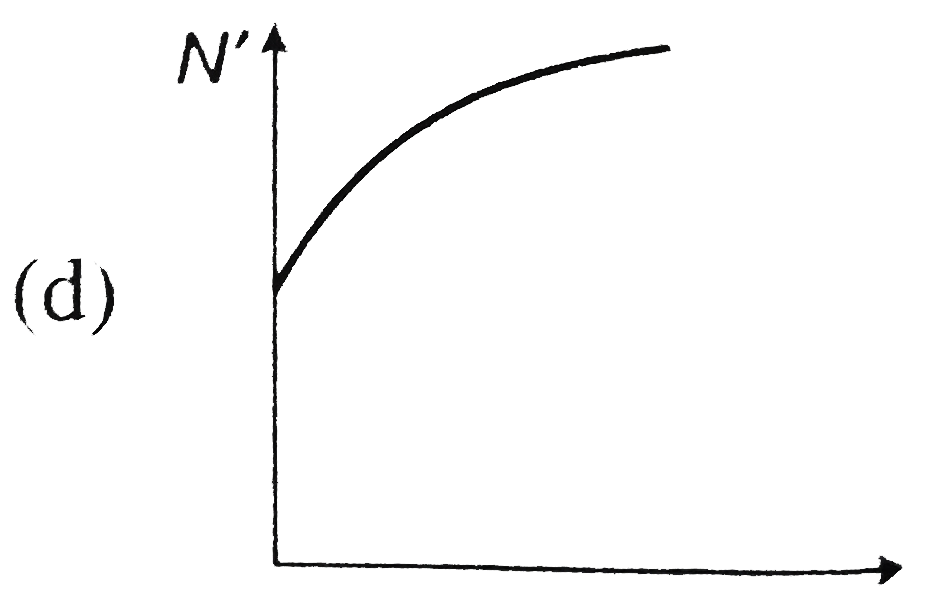 .
.
In a nuclear reactor an element X decays to a radio active element Y at a constant rate 1015 atoms per sec. Each decay release 100MeV energy. Half life of Y equals T and decays to a stable product Z. Each decay of Y releases 50MeV. All energy released inside the reactor is used to produce electricity at an afficiency of 25%. Calculate the electrical power in kw generated in the reactor in steady state.
View SolutionIn a nuclear reactor an element Xdecays to a radio active element Y at a constant rate 1015 atoms per sec. Each decay releases 100 MeV energy. Half life of Y equals T and decays to a stable product Z. Each decay of Y releases 66 MeV . All energy released inside the reactor is used to produce electricity at an efficiency of 25%. Calculate the electrical power in kW generated in the reactor in steady state, e=1.6×10−19
View SolutionN1 atoms of a radioactive element emit N2 beta partilces per second. The decay cosntant of the element is (in s−1)
View SolutionThe radioactive decay rate of a radioactive element is found to be 103 disintegration per second at a certain time . If the half life of the element is one second , the decay rate after one second is?
View SolutionHalf-life on an element is 't' secound. In t/2 second the fraction of the element decayed is:
View Solution
