Two positive numbers, a and b, are in the sequence 4. a,b,12. The first three numbers form a geometric sequence, and the last three numbers form an arithmetic sequence. The difference b-a equals
1
112
2
3
The correct Answer is:D
To solve the problem, we need to find the difference b−a given that the numbers 4,a,b,12 form a geometric sequence for the first three numbers and an arithmetic sequence for the last three numbers.
1. Identify the properties of the sequences:
- The first three numbers 4,a,b form a geometric sequence. This means that the ratio of consecutive terms is constant.
- The last three numbers a,b,12 form an arithmetic sequence. This means that the difference between consecutive terms is constant.
2. Set up the equations for the geometric sequence:
- For the geometric sequence 4,a,b, we have:
a4=ba
- Cross-multiplying gives:
a2=4b(Equation 1)
3. Set up the equations for the arithmetic sequence:
- For the arithmetic sequence a,b,12, we have:
b−a=12−b
- Rearranging gives:
2b=a+12(Equation 2)
4. Substitute Equation 1 into Equation 2:
- From Equation 1, we know b=a24.
- Substitute b into Equation 2:
2(a24)=a+12
- Simplifying gives:
a22=a+12
- Multiplying through by 2 to eliminate the fraction:
a2=2a+24
- Rearranging gives:
a2−2a−24=0(Equation 3)
5. Solve the quadratic equation (Equation 3):
- We can factor the quadratic:
(a−6)(a+4)=0
- This gives us two potential solutions:
a=6ora=−4
- Since a must be positive, we take a=6.
6. Find b using the value of a:
- Substitute a=6 back into Equation 1:
b=624=364=9
7. Calculate b−a:
- Now we can find the difference:
b−a=9−6=3
Final Answer:
The difference b−a is 3.
- The first three numbers 4,a,b form a geometric sequence. This means that the ratio of consecutive terms is constant.
- The last three numbers a,b,12 form an arithmetic sequence. This means that the difference between consecutive terms is constant.
- For the geometric sequence 4,a,b, we have:
a4=ba
- Cross-multiplying gives:
a2=4b(Equation 1)
- For the arithmetic sequence a,b,12, we have:
b−a=12−b
- Rearranging gives:
2b=a+12(Equation 2)
- From Equation 1, we know b=a24.
- Substitute b into Equation 2:
2(a24)=a+12
- Simplifying gives:
a22=a+12
- Multiplying through by 2 to eliminate the fraction:
a2=2a+24
- Rearranging gives:
a2−2a−24=0(Equation 3)
- We can factor the quadratic:
(a−6)(a+4)=0
- This gives us two potential solutions:
a=6ora=−4
- Since a must be positive, we take a=6.
- Substitute a=6 back into Equation 1:
b=624=364=9
- Now we can find the difference:
b−a=9−6=3
The difference b−a is 3.
Topper's Solved these Questions
Similar Questions
If t8=4andt12=−2, find the first three terms of the arithmetic sequence.
View SolutionLet <an> be an arithmetic sequence whose first term is 1 and <bn> be a geometric sequence whose first term is 2. If the common ratio of geometric sequence is half the common difference of arithmetic sequence, the minimum value of (a4b1+a3b2+2a1b3) is equal to
View Solution
Knowledge Check
- Question 1 - Select One
The first three terms of a geometric sequence are 4√3,8√3,1. The fourth term is
A32√3B16√3C116√3D18√3 - Question 2 - Select One
What number should be added to each of the three numbers, 3,11 and 27 so that the resulting numbers form a geomatric sequence ?
A2B3C4D5
If each number of a sequence is 4 more than the previous number, and the 3rd number in the sequence is 13, what is the 114th number in the sequence?
View SolutionThe first three terms of a geometric progression are 48,24,12 . What are the common ratio and fourth term of this sequence ?
View SolutionIn the sequence of reactions , . The product B is
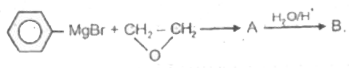 View Solution
View SolutionThe first two terms of an ordered sequence of positve integers are 1 and 3. if each number of the sequence after 3 is obtained by adding the two numbers immediately precedingit, how many the first 1,000 numbers in this sequence are even?
View SolutionIf the first four terms of an arithmetic sequence are a,2a,b and (a−6−b) for some numbers a and b, find the sum of the first 100 terms of the sequence.
View SolutionThe number of tripeptides formed by three different amino acids is:
View Solution

