A certain 4 inch spring stretches 1.5 inches for each ounce of weight attached to it.
The correct Answer is:L(x)=4+1.5x
To solve the problem, we need to determine the final length of the spring when a certain weight is attached to it. We know the following:
1. The initial length of the spring is 4 inches.
2. The spring stretches 1.5 inches for each ounce of weight attached to it.
Let's denote the weight attached to the spring in ounces as x.
Step 1: Identify the stretch of the spring based on the weight.
- The stretch of the spring for x ounces of weight is given by the formula:
Stretch=1.5×x
Step 2: Calculate the final length of the spring.
- The final length of the spring can be calculated by adding the initial length of the spring to the stretch caused by the weight:
Final Length=Initial Length+Stretch
- Substituting the values we have:
Final Length=4+(1.5×x)
Step 3: Write the final expression for the length of the spring.
- Therefore, the final length of the spring as a function of the weight x is:
Final Length=4+1.5x
Final Expression:
The final length of the spring when x ounces of weight is attached is given by:
Final Length=4+1.5x
2. The spring stretches 1.5 inches for each ounce of weight attached to it.
- The stretch of the spring for x ounces of weight is given by the formula:
Stretch=1.5×x
- The final length of the spring can be calculated by adding the initial length of the spring to the stretch caused by the weight:
Final Length=Initial Length+Stretch
- Substituting the values we have:
Final Length=4+(1.5×x)
- Therefore, the final length of the spring as a function of the weight x is:
Final Length=4+1.5x
The final length of the spring when x ounces of weight is attached is given by:
Final Length=4+1.5x
Topper's Solved these Questions
- PROBLEM SOLVING AND DATA ANALYSISBOOK - ENGLISH SATCHAPTER - PROBLEM SOLVING AND DATA ANALYSISEXERCISE - Multiple Choice
128 Videos
- PROBLEM SOLVING AND DATA ANALYSISBOOK - ENGLISH SATCHAPTER - PROBLEM SOLVING AND DATA ANALYSISEXERCISE - Grib-In
40 Videos
- PROBLEM SOLVING AND DATA ANALYSISBOOK - ENGLISH SATCHAPTER - PROBLEM SOLVING AND DATA ANALYSISEXERCISE - EXERICSE
80 Videos
- PROBLEMS IN CONTEXT BOOK - ENGLISH SATCHAPTER - PROBLEMS IN CONTEXT EXERCISE - PRACTICE TEST
10 Videos
Similar Questions
On a certain map, 1 inch represents 2 kilometers. A region is located on the map that is 1.5 inches by 4.0 inches. What is the actual area of the region in square miles if 1 kilometer is equal to 0.6 mile?
View SolutionThe length of a rectangle is 15 inches and the breadth 12 inches. Calculate its area in SI units.
View Solution
Knowledge Check
A rectangular box with length 22 inches , width 5 inches , and height 5 inches is to be packed with steel balls of radius 2 inches in such a way that the centers of the balls are collinear. What is the maximum number of balls that can fit into the box, provided that no balls should protrude from the box ?
A0B5C6D10The amount of light produced by a cylindrical fluorescent light bulb depends on its lateral area. A certain cylindrical fluorescent light bulb is 36 inches long, has a 1 inch diameter, and is manufactured to produce 0.283 wants of light per square inch. What is the best estimate for the total amount of light that it is able to produce?
A32 wattsB34 wattsC40 wattsD48 wattsl=24+3.5m
One end of a spring is attached to a ceiling. When an object of mass m kilograms is attached to the other end of the spring, the spring stretches to a length of l centimeters as shown in the equation above. What is m when l is 73 ?A14B27.7C73D279.5
A spherical balloon is expanding. If the radius in increasing at the rate of 2 inches per minute the rate at which the volume increases (in cubic inches per minute) when the radius is 5 inches is
View SolutionCarla cuts a 70 - inch piece of ribbon into 2 pieces. If the first piece is 5 inches more than one - fourth as long as the second piece, how long is the longer piece of ribbon?
View Solution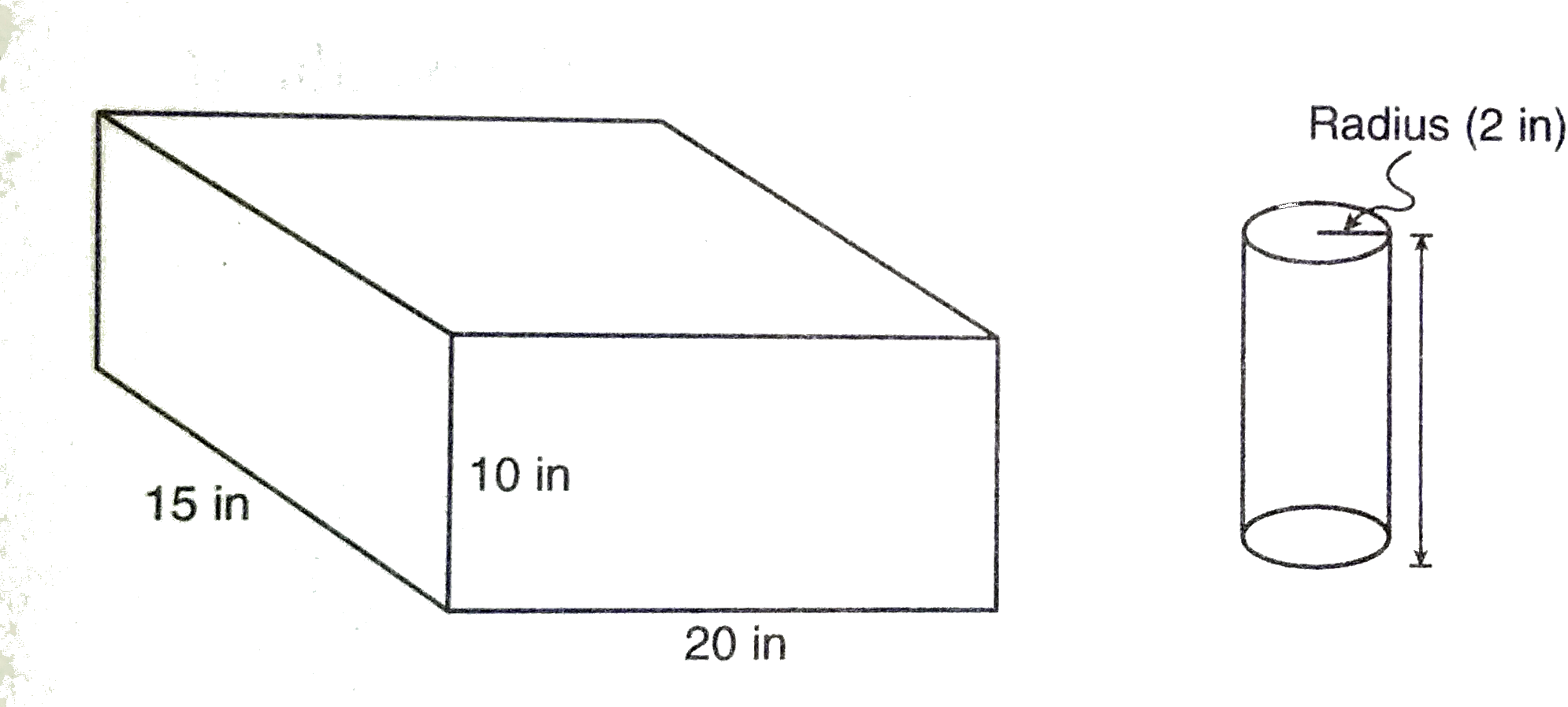
In the figure above, a rectangular container with the dimensions 10 inches by 15 inches by 20 inches is to be filled with water, using a cylindrical cup whose radius is 2 inches and whose height is 5 inches. What is the maximum number of full cups of water that can be placed into the container without the water overflowing the container?
View SolutionA ramp rises 6 inches for each 24 inches of horizontal run . Thus ramp rises many inches for 62 inches of horizontal run ?
View SolutionA company ships notepads in rectangular boxes that each have inside dimensions measuring 9 inches long, 9 inches wide, and 12 inches tall. Each notepad is in the shape of a cube with an edge length of 3 inches. What is the maximum number of natepads that will fit in 1 closed box?
View Solution
