A fort is at the top of a hill of height h above the sea-level.Prove that the greatest horizontal distance from which a gun in the ship can hit the fort is 2√k(k−h) where √2gk is the muzzle velocity.
Step by step video & image solution for A fort is at the top of a hill of height h above the sea-level.Prove that the greatest horizontal distance from which a gun in the ship can hit the fort is 2sqrt(k(k-h)) where sqrt(2gk) is the muzzle velocity. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A projectile is launched at an angle α from a cliff of height H above the sea level. If it falls into the sea at a distance D from the base of the cliff, show that its maximum height above the sea level is
[H+D2tan2α4(H+Dtanα)]
View SolutionA particle is fired horizontally with a velocity of 100ms−1 for the top of a hill 400m high. Find (i) the time taken to reach the ground (ii) thedistance of the target from the hill and (iii) the velocity with which the projectile hits the ground. Take g=10ms−2.
View Solution
Knowledge Check
A projectile is fired horizontally with velocity of 98 m/s from the top of a hill 490 m high. Find
(a) the time taken by the projectile to reach the ground,
(b) the distance of the point where the particle hits the ground from foot of the hill and
(c) the velocity with which the projectile hits the ground. (g=9.8m/s2)
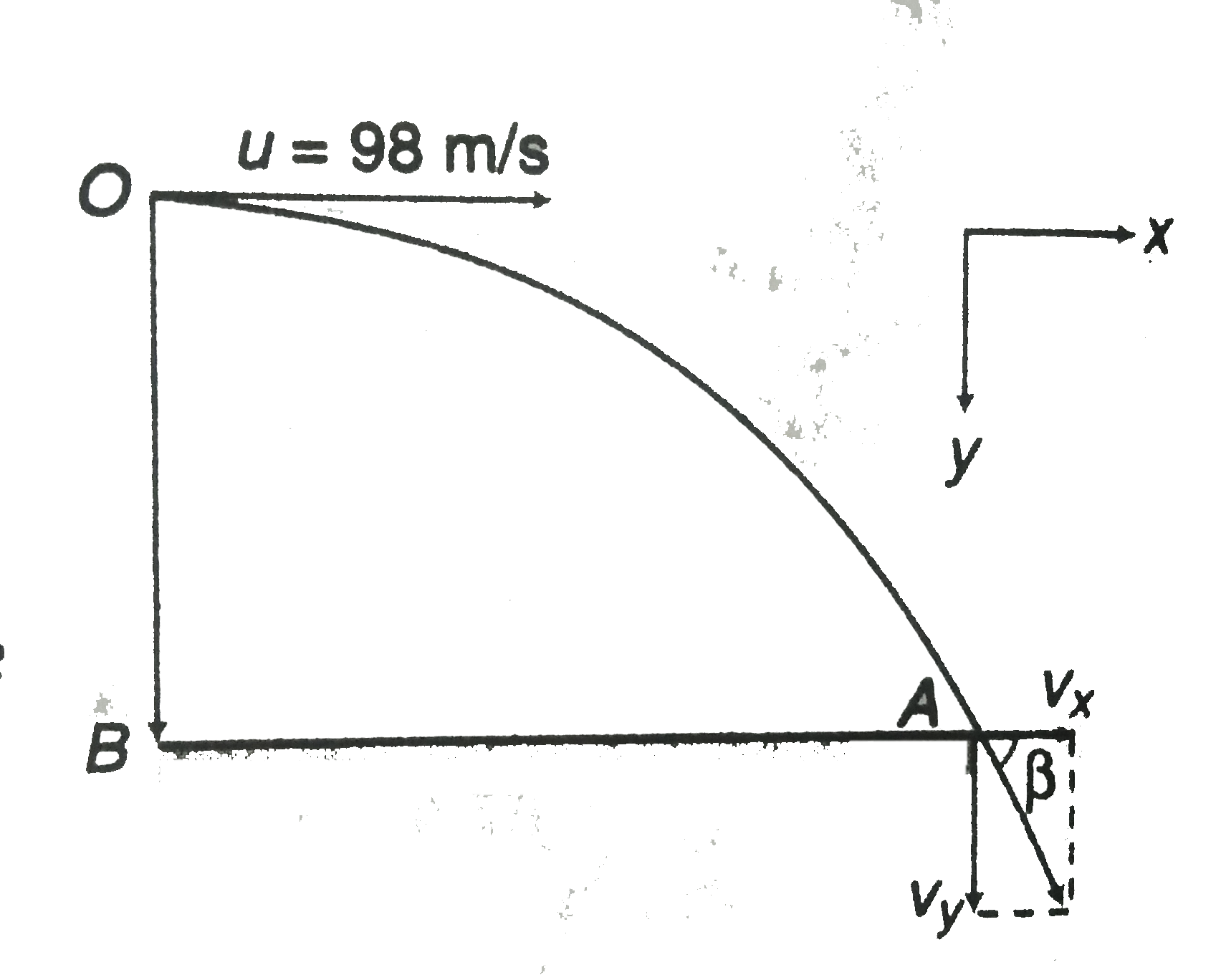 .A12s , 980m , 98(√2)m/s β=45B10s , 98m , 98(√2)m/s β=45C10s , 980m , 98m/s β=45D10s , 980m , 98(√2)m/s β=45
.A12s , 980m , 98(√2)m/s β=45B10s , 98m , 98(√2)m/s β=45C10s , 980m , 98m/s β=45D10s , 980m , 98(√2)m/s β=45A stone is projected at an angle α to the horizontal from the top of a tower of height 3h If the stone reaches a maximum height h above the tower, then calculate the distance from the foot of the tower where particle strikes the foot of the where particle strikes the ground.
A6htanαB6hcotαChtanαDhcotαA body, rpojected horizontally with a speed u from the top of a tower of height h, reaches the ground at a horizontally distance R from the tower. Another body, projected horizontally from the top of a tower of height 4h, reaches the ground at horizontal distance 2R from the tower. The initial speed of the second body is
AuB2uC3uD4u
A particle is projected from a point on the level ground and its height is h when at horizontal distances aand2a from its point of projection. Find the velocity of projection.
View SolutionA body projected horizontally with a velocity V from the top of a tower of height h touches the level ground at a distance X from the foot of the tower. Another body is projected horizontally with velocity 2V simultaneously from the same point exactly in the opposite direction. On reaching the ground, separation between the two bodies is?
View SolutionA stone is thrown up from the top of a tower 20,m with a velocity of 24m/s a tan elevation of 30∘ above the horizontal. Find the horizontal distance from the foot of the tower to the point at which the stone hits the ground. Take g=10m/s2
View SolutionA ship is approaching a cliff of height 105 m above sea level . A gun fitted on the ship can fire shots with a speed of 110ms−1 . Find the maximum distance from the foot of the cliff from where the gun can hit an object on the top of the cliff.
View Solution(a) With what velocity (v0) should a ball be projected horizontally from the top of a tower so that the horizontal distance on the ground is ηH, where H is the height of the tower ?
(b) Also determine the speed of the ball when it reaches the ground.
 .
.
View Solution
