Consider a reaction A(g)k=0.1M−1min−−−−−−−→2B(g). If initial concentration of A is 0.5 M then select correct graph.
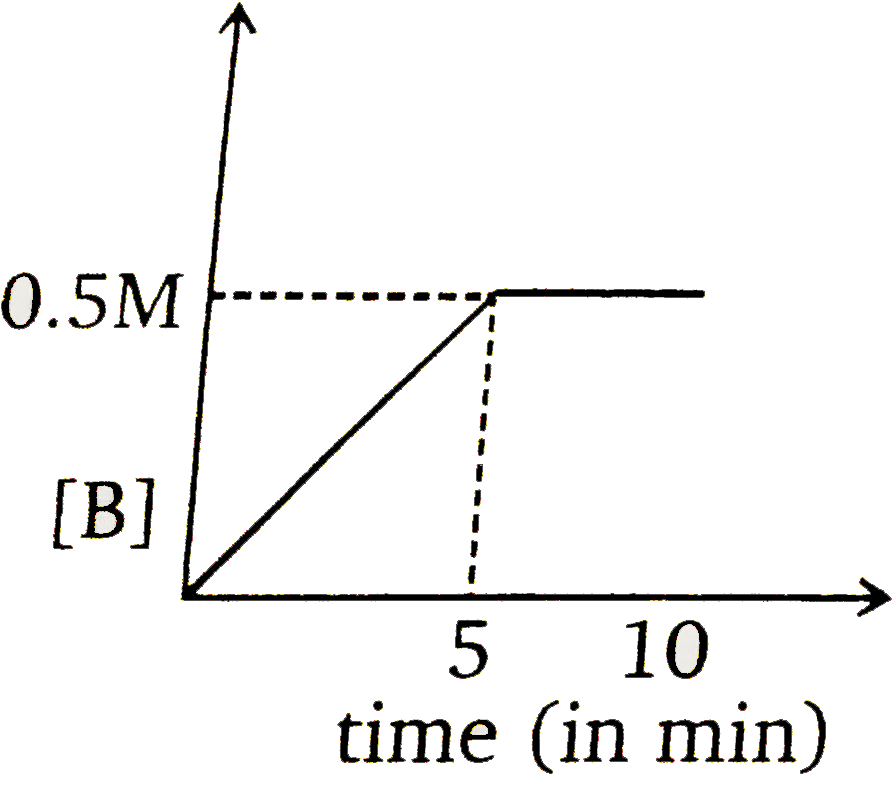
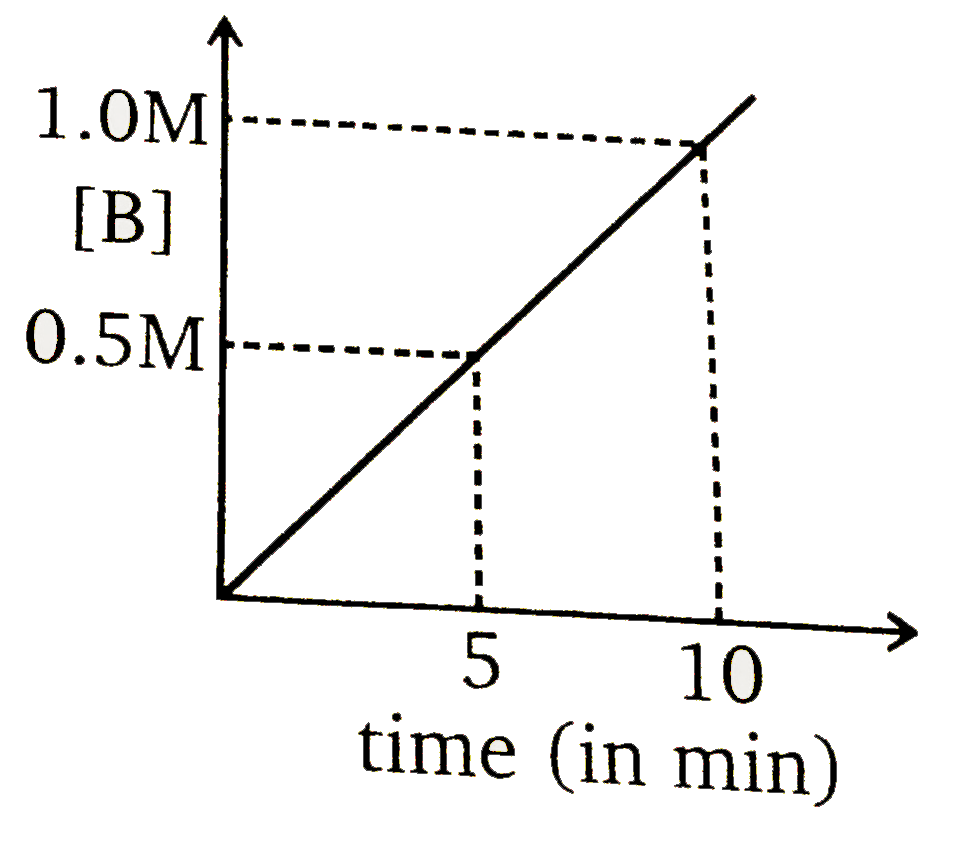
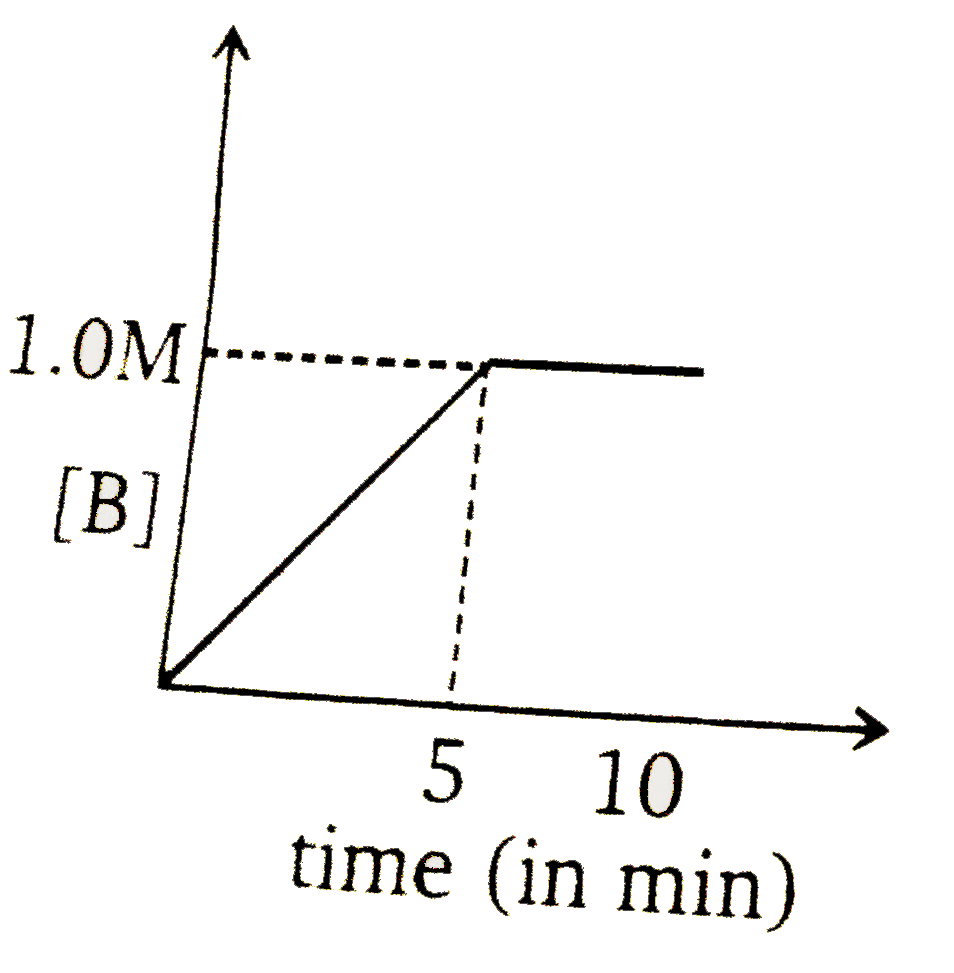
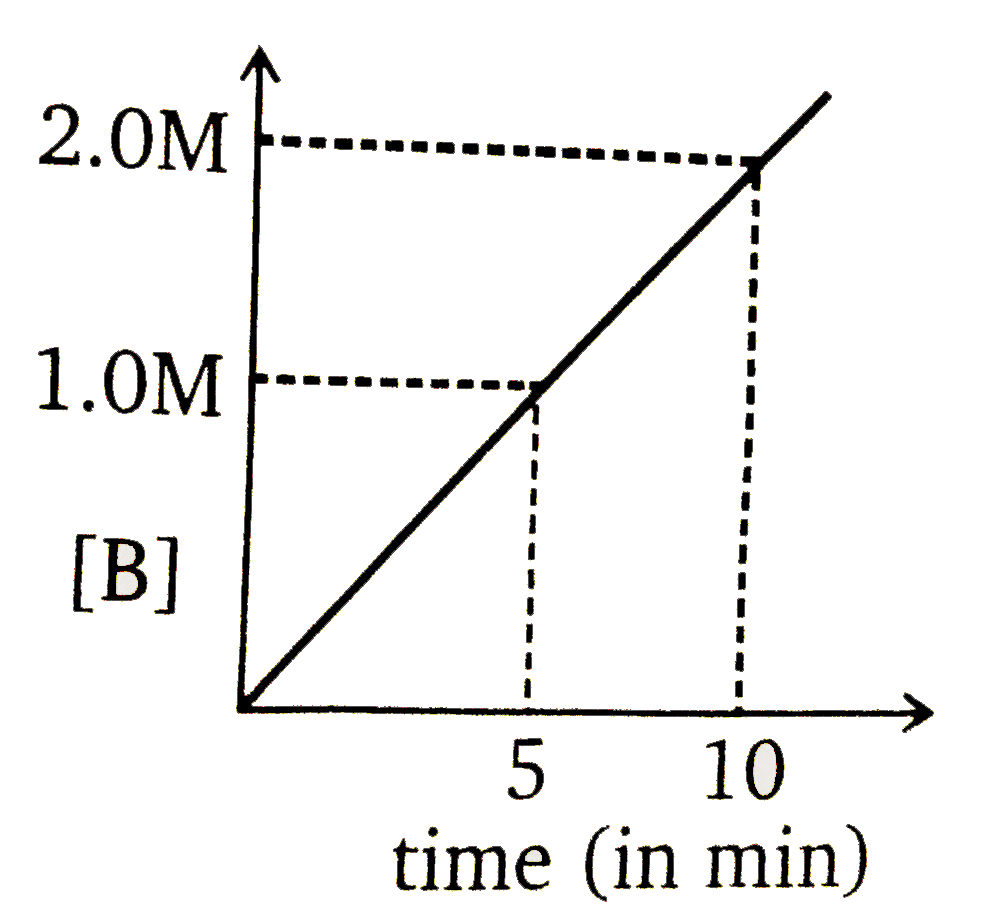
The correct Answer is:C
To solve the problem, we need to analyze the reaction and its kinetics step by step.
Step 1: Identify the Reaction Order
The reaction given is:
A(g)k=0.1M min−1→2B(g)
The rate constant k has units of M min−1. This suggests that the reaction is a zero-order reaction because the unit of the rate constant for a zero-order reaction is M⋅time−1.
Step 2: Write the Rate Law Expression
For a zero-order reaction, the rate law can be expressed as:
Rate=k
And the concentration of the reactant A over time can be described by the equation:
(A)=(A0)−kt
Where:
- (A0) is the initial concentration of A
- k is the rate constant
- t is time
Step 3: Substitute Known Values
Given:
- (A0)=0.5M
- k=0.1M min−1
Substituting these values into the equation gives:
(A)=0.5−(0.1⋅t)
Step 4: Calculate Concentration of A at Different Times
1. At t=0 min:
(A)=0.5−(0.1⋅0)=0.5M
2. At t=5 min:
(A)=0.5−(0.1⋅5)=0.5−0.5=0M
3. At t=10 min:
(A)=0.5−(0.1⋅10)=0.5−1=−0.5M
(This is not possible, indicating that the reaction will stop before reaching this time.)
Step 5: Determine Concentration of B
Since the stoichiometry of the reaction shows that 1 mole of A produces 2 moles of B:
- At t=5 min, when (A)=0:
(B)=2×(0.5)=1M
Step 6: Analyze the Graphs
We need to select the correct graph based on the concentration of B:
- At t=0: (B)=0M
- At t=5: (B)=1M
- At t>5: (B) remains constant at 1M because A is fully consumed.
Conclusion
The correct graph should show:
- An increase in the concentration of B from 0 to 1M at t=5 minutes.
- A plateau at 1M for t>5 minutes.
The reaction given is:
A(g)k=0.1M min−1→2B(g)
For a zero-order reaction, the rate law can be expressed as:
Rate=k
And the concentration of the reactant A over time can be described by the equation:
(A)=(A0)−kt
Where:
- (A0) is the initial concentration of A
- k is the rate constant
- t is time
Given:
- (A0)=0.5M
- k=0.1M min−1
(A)=0.5−(0.1⋅t)
1. At t=0 min:
(A)=0.5−(0.1⋅0)=0.5M
(A)=0.5−(0.1⋅5)=0.5−0.5=0M
(A)=0.5−(0.1⋅10)=0.5−1=−0.5M
(This is not possible, indicating that the reaction will stop before reaching this time.)
Since the stoichiometry of the reaction shows that 1 mole of A produces 2 moles of B:
- At t=5 min, when (A)=0:
(B)=2×(0.5)=1M
We need to select the correct graph based on the concentration of B:
- At t=0: (B)=0M
- At t=5: (B)=1M
- At t>5: (B) remains constant at 1M because A is fully consumed.
The correct graph should show:
- An increase in the concentration of B from 0 to 1M at t=5 minutes.
- A plateau at 1M for t>5 minutes.
Topper's Solved these Questions
- BOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 1 (Q.3 To Q.32)2videos
CHEMICAL KINETIC & NUCLEAR CHEMISTRY
- BOOK - NARENDRA AWASTHICHAPTER - CHEMICAL KINETIC & NUCLEAR CHEMISTRYEXERCISE - Level 1 (Q.33 To Q.62)3videos
CHEMICAL KINETIC & NUCLEAR CHEMISTRY
- BOOK - NARENDRA AWASTHICHAPTER - SURFACE CHEMISTRYEXERCISE - Level 3 - Assertion - Reason Type Questions1videos
SURFACE CHEMISTRY
Similar Questions
The rate constant for a first order reaction was found to be 0.082−1min. If initial concentration of reactant is 0.15M, how long would it take:
(a) to reduce the concentration of A to 0.03M?
(b) to reduce the concentration of A by 0.03M?View SolutionFor a zero order reaction : A → 2B, initial rate of a reaction is 10−1M min−1. If concentration of 'A' is 0.1 after 120 sec then what would be concentration of B after 60 sec.
View Solution
Knowledge Check
- Question 1 - Select One
For a reaction Akr=0.6M−1min−−−−−−−−→2B
starting with 1 M of 'A' only, concentration of B (in M) after 100 sec. and 200 sec. is respectively?A2 and 4B1 and 2C2 and 3Dnone of these - Question 2 - Select One
For a reaction Akr=0.6M−1min−−−−−−−−→2B
starting with 1 M of 'A' only, concentration of B (in M) after 100 sec. and 200 sec. is respectively?A2 and 4B1 and 2C2 and 3DNone of these - Question 3 - Select One
The rate law expresses the relationship of the rate of a reaction to the rate constant and the concentration of the reactants raised to some powers for the general reaction.
aA+bB→cC+dD
Rate law takes the form
r=k[A]x[B]y
where x and y are number that must be determined experimentely k is the rate constant and [A] and [B] are concentration of A & B respectively.
The initial rate of zero order reaction of the gaseous equation A(g)→2B(g) is 10−2M−1min If the initial concentration of A is 0.1 M what would be concentration of B after 60 seconds ?A0.09 MB0.01 MC0.02 MD0.03 M
For any reaction A(g)→B(g), rate constant k=8.21×10−2 atm/min at 300 K. If initial concentration of A is 2M then what is the half life (in hr.)?
View SolutionFor a particular reaction (A → B) the rate constant is 0.693 −1min If the initial concentration of the reactant, A is 1 M, then the rate of reaction after 1 minute will be
View SolutionConsider the reaction :
P(g)+2Q(g)KC=1012⇐===⇒R(g)+S(g)Initial conc.2M4M00
then select correct statement(s)View SolutionIn a first order reaction A→B, if k is rate constant and initial concentration o f the reactant A is 0.5 M then the half-life is
View SolutionIn a first order reaction A→B, if k is rate constant and initial concentration o f the reactant A is 0.5 M then the half-life is
View Solution

